A uniform cylinder (mass M) of radius R is kept on an accelerating platform (mass M) as shown in figure. If the cylinder rolls withut slipping on the platform, determine the magnitude of acceleration of the centre of mass of the cylinder. Assuming th coefficient of friction μ=0.40, determinete maximum acceleration the platform may have without slip between the clyinder and the platform.
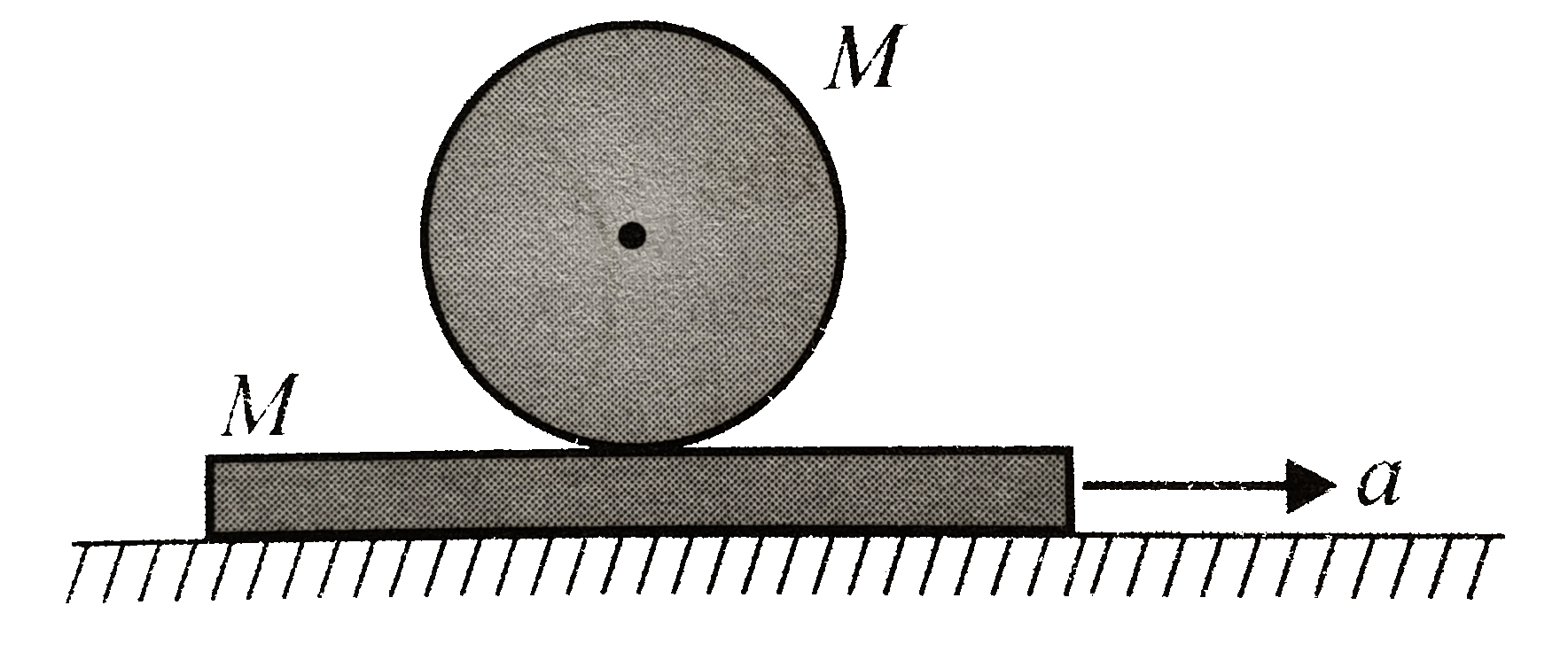
Figure shows the free body diagram of the cylinder. We have assumed the angular acceleration of the cylinder to be clockwise. In case of pure rolling the contact point of cylinder with surface does not slide. Therefore, the acceleration of th point P must be equal to that of the platform.

But a=a0−Rα ........i
Which yields a0=Rα+a
the equation of motion are
ΣFx=f=(M)a0
Στ=−f×R=(12MR2)α..........iii
Negative sign shows that the torque of friction is opposite to the assume α.
From eqn i and ii we get
f=M(Rα)..........iv
Sunstituting the expression for f in eqn ii we obtain
Rα=−23a
From eqn i a0=−23+=13a
The maximum value of frictional force fmax=μN where N=Mg, from eqn ii
0.40N=M(13a)
=0.0Mg=M(13a)
a=(0.40)(3g)=12m/s2
Which is the maximum acceleration the platform may have without slipping between the cylinder and the platform.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Solved Examples12videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.111videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
A wheel is rolling without slipping on a horizontal plane with velocity v and acceleration a of centre of mass as shown in figure. Acceleration atView Solution
A uniform solid cylinder having mass M and radius R is pulled by a horiozntal force F acting through the center as shown. The cylinder rolls to the right without slipping. What is the magnitude of the force of friction between the cylinder and the ground?View SolutionThe rope shown in figure is wound around a cylinder of mass 4kg and moment of inertia 0.02kgm2 about the cylinder axis. If the cylinder rolls without slipping, then the linear acceleration of its centre of mass is.
 .View Solution
.View Solution
A uniform cylinder of mass M and radius R is released from rest on a rough inclined surface of inclination θ with the horizontal as shown in figure. As the cylinder rolls down the inclined surface, the maximum elongation it the spring stiffness k isView SolutionA homogeneous cylinder of mass Mand radius r is pulled on a horizontal plane by a horizontal force F acting through its centre of mass. Assuming rolling without slipping, find the angular acceleration of the cylinder,
View SolutionA homogeneous cylinder of mass Mand radius r is pulled on a horizontal plane by a horizontal force F acting through its centre of mass. Assuming rolling without slipping, find the angular acceleration of the cylinder,
View Solution
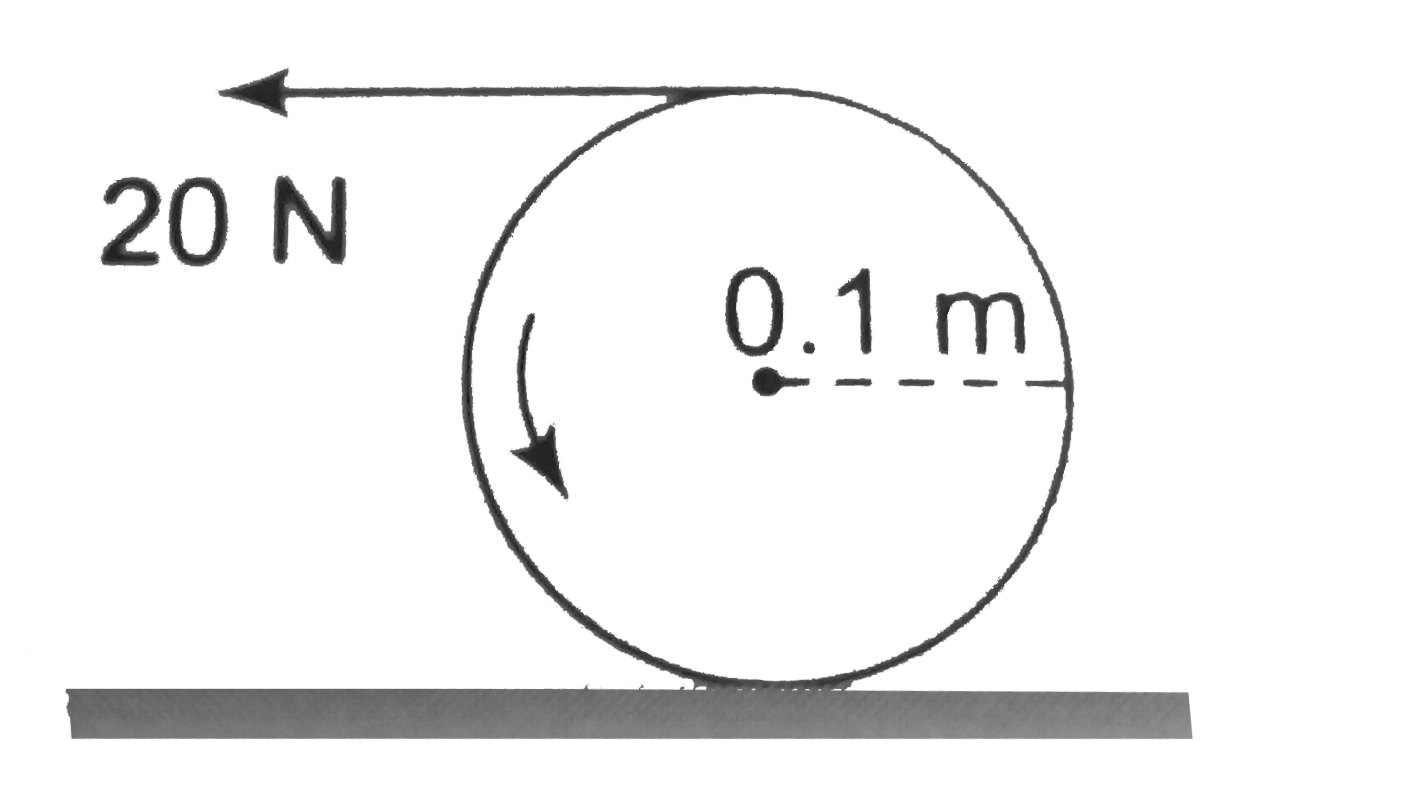
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder isView Solution
A cylinder of radius R is to roll without slipping between two planks as shown in the figure. ThenView SolutionA solid cylinder of mass M and radius R pure rolls on a rough surface as shown in the figure. Choose the correct alternative (s).
 View Solution
View SolutionA disc of mass m and radius R is rolling without slipping as shown in the figure. The magnitude of net velocity of the point P is (OP=R2)
View Solution

