A thin square plate of side 5cm is suspended vertically a balance so that lower side just dips into water with side to surface. When the plate is clean (θ=0∘), it appears to weigh 0.044N. But when the plate is greasy (θ=180∘) it appears to weigh 0.03N. The surface tension of water is
3.5×10−2N/m
7.0×10−2N/m
14.0×10−2N/m
1.08N/m
The correct Answer is:B
To find the surface tension of water based on the given problem, we can follow these steps:
1. Identify the parameters:
- Side of the square plate, a=5cm=0.05m
- Weight of the plate when clean, W=0.044N
- Apparent weight of the plate when greasy, W′=0.03N
2. Calculate the perimeter of the plate:
The perimeter P of the square plate is given by:
P=4a=4×0.05m=0.2m
3. Understand the forces acting on the plate:
- When the plate is clean, the weight W is balanced by the spring force (the reading on the balance).
- When the plate is greasy, the apparent weight W′ is reduced due to the upward force from the surface tension.
4. Set up the equation for the greasy plate:
The surface tension force Fs acts upwards and can be expressed as:
Fs=T×P
where T is the surface tension and P is the perimeter of the plate.
The relationship when the plate is greasy can be written as:
W′=W−Fs
Substituting for Fs:
W′=W−T×P
5. Rearranging the equation:
Rearranging the above equation gives:
T×P=W−W′
Therefore:
T=W−W′P
6. Substituting the values:
Now we can substitute the known values into the equation:
T=0.044N−0.03N0.2m=0.014N0.2m=0.07N/m
7. Final answer:
The surface tension of water is:
T=0.07N/m
- Side of the square plate, a=5cm=0.05m
- Weight of the plate when clean, W=0.044N
- Apparent weight of the plate when greasy, W′=0.03N
The perimeter P of the square plate is given by:
P=4a=4×0.05m=0.2m
- When the plate is clean, the weight W is balanced by the spring force (the reading on the balance).
- When the plate is greasy, the apparent weight W′ is reduced due to the upward force from the surface tension.
The surface tension force Fs acts upwards and can be expressed as:
Fs=T×P
where T is the surface tension and P is the perimeter of the plate.
W′=W−Fs
Substituting for Fs:
W′=W−T×P
Rearranging the above equation gives:
T×P=W−W′
Therefore:
T=W−W′P
Now we can substitute the known values into the equation:
T=0.044N−0.03N0.2m=0.014N0.2m=0.07N/m
The surface tension of water is:
T=0.07N/m
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Multiple Correct
17 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Assertion- Reasoning
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Subjective
16 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
Two large glass plates are placed vertically and parallel to each other inside a tank of water with separation between the plates equal to 1 mm. Find the rise of water in the space between the plates. Surface tension of water =0.075Nm−1.
View SolutionA thin uniform square plate ABCD of side a and mass m is suspended in a vertical plane as shown in the figure. AE and BF are two massless inextensible strings. The line AB is horizontal. The tension in AE just after BF is cut will be
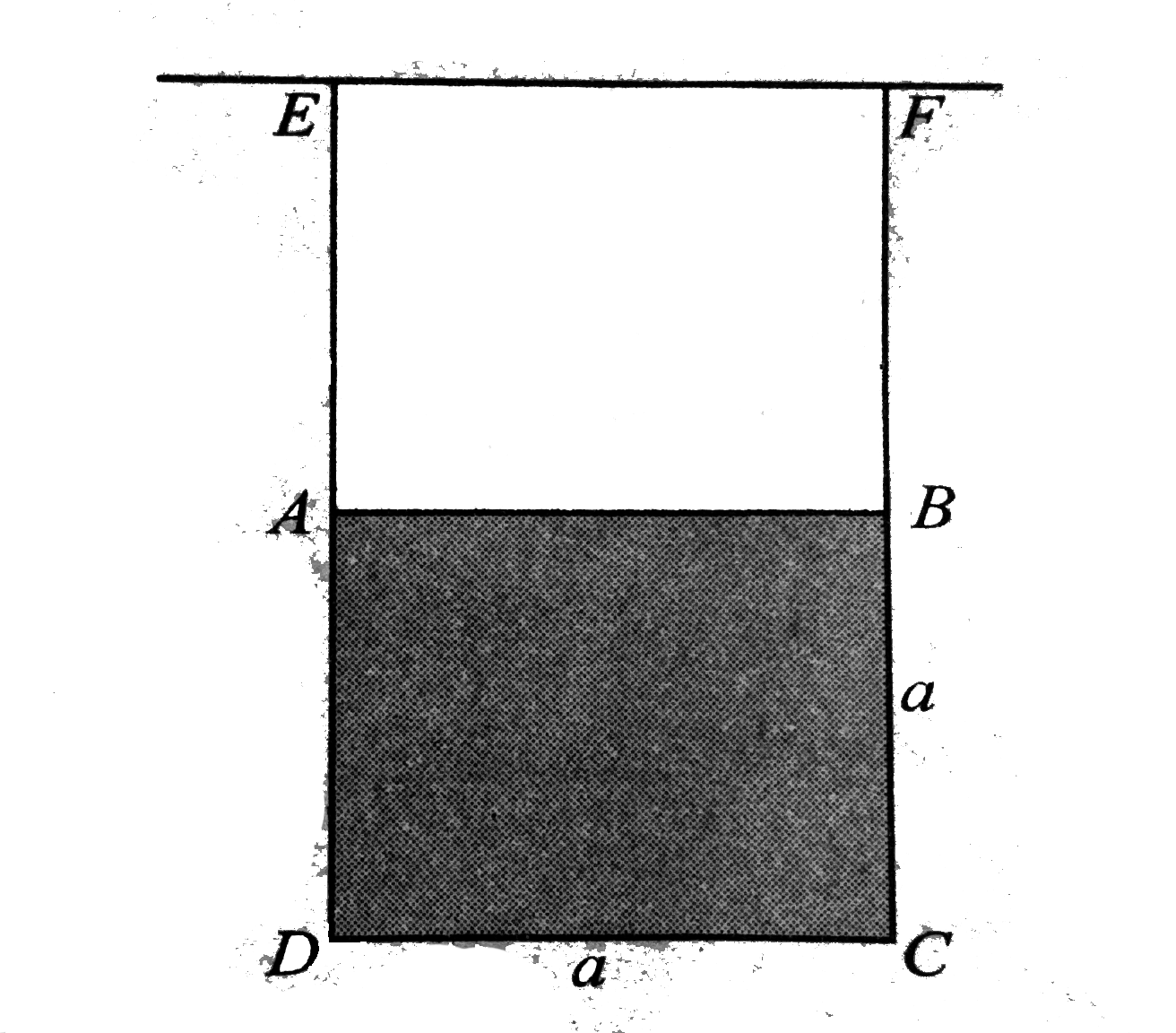
View Solution
Knowledge Check
If the surface tension of water is 0.06 N/m, then the capillary rise in a tube of diameter 1mm is (θ=0∘)
A1.22 cmB2.44 cmC3.12 cmD3.86 cm
A square glass plate of length 10 cm and thickness 0.4 cm, weight 40 g in air. It is held vertically such that its lower edge rests on water surface. What is the apparent weight of glass plate now? (Given surface tension of water = 0.073 N m−1)
View SolutionA metal ball weighs 0.096N.When suspended in water it has an apparent weight of 0.071N. Find the density of the metal.
View SolutionA tube of conical bore is dipped into water with apex upwards. The length of the tube is 20cm and radii at the upper and lower ends are 0.1 and 0.3 cm. Find the height to which the liquid rises in the tube ( Surface tension of water = 80 dyne / cm ).
View SolutionA capillary tube of radius 0.25 mm is submerged vertically in water so that 25 mm of its length is outside water. The radius of curvature of the meniscus will be
(surface tension of water =75×10−3 N/m)
View SolutionA rectangular glass plate of dimensions 5 cm × 4 cm is placed flat on the surface of water. Find the downward force on the plate due to surface tension. Given surface tension of water = 0.073 N m−1
View SolutionTwo square metal plates of side 1m are kept 0.01m apart like a parallel plate capacitor in air in such a way that one of their edges is perpendicualr to an oil surface in the a tank filled with an insulating oil. The plates are connected to a battery of emf 500V. The plates are then lowered vertically into the oil at a speed of 0.001ms−1. Calculate the current drawn from the battery during the process.
(Dielectric constant of oil =11,ε0=8.85×10−12N−1m−2).
View SolutionCalculate the force required to separate the glass plates of area 10−2m2 with a film of water 0.05 mm thickness between them (surface tension of water =70×10−3N/m))
View Solution
