A man whose mass is m kg jumps vertically into air from the sitting position in which his centre of mass is at a height h1 from the ground. When his feet are just about to leave the ground his centre of mass is at height h2 from the ground and finally centre of mass rises to h3 above the ground when he is at the top of the jump. what is the average upward force exerted by the ground on him?
(a)mg(h3−h1)(h3−h2)
(b)mg(h3−h1)h3
(c)mg(h3−h1)(h2−h1)
(d)none of these
The correct Answer is:C
To find the average upward force exerted by the ground on a man who jumps vertically, we can follow these steps:
Step 1: Understand the Problem
We have a man with mass m kg who jumps from a sitting position. His center of mass is at different heights during the jump:
- Initial height when sitting: \( h_1 \)
- Height just before leaving the ground: \( h_2 \)
- Maximum height reached: \( h_3 \)
Step 2: Analyze the Motion
When the man jumps, he accelerates upwards until he reaches the maximum height \( h_3 \). At this point, his velocity becomes zero. We can use the kinematic equation to relate the heights and velocities:
\(
v^2 = u^2 + 2as
\)
Where:
- \( v \) = final velocity (0 at height \( h_3 \))
- \( u \) = initial velocity at height \( h_2 \)
- \( a \) = acceleration (which is \(-g\) due to gravity)
- \( s \) = displacement (\( h_3 - h_2 \))
Step 3: Apply the Kinematic Equation
Since the final velocity \( v = 0 \) at height \( h_3 \), we can write:
\(
0 = v^2 - 2g(h_3 - h_2)
\)
Rearranging gives:
\(
v^2 = 2g(h_3 - h_2)
\)
Step 4: Calculate Change in Kinetic Energy
The change in kinetic energy as the man jumps from height \( h_1 \) to height \( h_2 \) is equal to the work done by the average force exerted by the ground. The work done can be expressed as:
\(
\text{Work done} = F \cdot (h_2 - h_1)
\)
And the change in kinetic energy is:
\(
\Delta KE = \frac{1}{2} mv^2
\)
Step 5: Set Up the Equation
Equating the work done to the change in kinetic energy:
\(
F(h_2 - h_1) = \frac{1}{2} mv^2
\)
Substituting \( v^2 \) from the previous step:
\(
F(h_2 - h_1) = \frac{1}{2} m(2g(h_3 - h_2))
\)
This simplifies to:
\(
F(h_2 - h_1) = mg(h_3 - h_2)
\)
Step 6: Solve for Average Force \( F \)
Rearranging gives:
\(
F = \frac{mg(h_3 - h_2)}{h_2 - h_1}
\)
Step 7: Include Weight of the Man
The total force exerted by the ground must also counteract the weight of the man:
\(
F - mg = \frac{mg(h_3 - h_2)}{h_2 - h_1}
\)
Thus,
\(
F = mg + \frac{mg(h_3 - h_2)}{h_2 - h_1}
\)
Factoring out \( mg \):
\(
F = mg\left(1 + \frac{h_3 - h_2}{h_2 - h_1}\right)
\)
Final Result
The average upward force exerted by the ground on the man is:
\(
F = mg \cdot \frac{h_3 - h_1}{h_2 - h_1}
\)
We have a man with mass m kg who jumps from a sitting position. His center of mass is at different heights during the jump:
- Initial height when sitting: \( h_1 \)
- Height just before leaving the ground: \( h_2 \)
- Maximum height reached: \( h_3 \)
When the man jumps, he accelerates upwards until he reaches the maximum height \( h_3 \). At this point, his velocity becomes zero. We can use the kinematic equation to relate the heights and velocities:
\(
v^2 = u^2 + 2as
\)
Where:
- \( v \) = final velocity (0 at height \( h_3 \))
- \( u \) = initial velocity at height \( h_2 \)
- \( a \) = acceleration (which is \(-g\) due to gravity)
- \( s \) = displacement (\( h_3 - h_2 \))
Since the final velocity \( v = 0 \) at height \( h_3 \), we can write:
\(
0 = v^2 - 2g(h_3 - h_2)
\)
Rearranging gives:
\(
v^2 = 2g(h_3 - h_2)
\)
The change in kinetic energy as the man jumps from height \( h_1 \) to height \( h_2 \) is equal to the work done by the average force exerted by the ground. The work done can be expressed as:
\(
\text{Work done} = F \cdot (h_2 - h_1)
\)
And the change in kinetic energy is:
\(
\Delta KE = \frac{1}{2} mv^2
\)
Equating the work done to the change in kinetic energy:
\(
F(h_2 - h_1) = \frac{1}{2} mv^2
\)
Substituting \( v^2 \) from the previous step:
\(
F(h_2 - h_1) = \frac{1}{2} m(2g(h_3 - h_2))
\)
This simplifies to:
\(
F(h_2 - h_1) = mg(h_3 - h_2)
\)
Rearranging gives:
\(
F = \frac{mg(h_3 - h_2)}{h_2 - h_1}
\)
The total force exerted by the ground must also counteract the weight of the man:
\(
F - mg = \frac{mg(h_3 - h_2)}{h_2 - h_1}
\)
Thus,
\(
F = mg + \frac{mg(h_3 - h_2)}{h_2 - h_1}
\)
Factoring out \( mg \):
\(
F = mg\left(1 + \frac{h_3 - h_2}{h_2 - h_1}\right)
\)
The average upward force exerted by the ground on the man is:
\(
F = mg \cdot \frac{h_3 - h_1}{h_2 - h_1}
\)
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - MCQ_TYPE
20 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - LC_TYPE
34 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
Similar Questions
A ball is thrown upwards with a speed u from a height h above the ground.The time taken by the ball to hit the ground is
View SolutionA body of mass m=2kg falls from a height h=10m from the ground level .
What is the magnitude of total change in momentum of the body before it strikes the ground?
View SolutionA tank is filled up to a height 2H with a liquid and is placed on a platform of height H from the ground, the distance x from the ground where a small hole is punched to get the maximum range R is
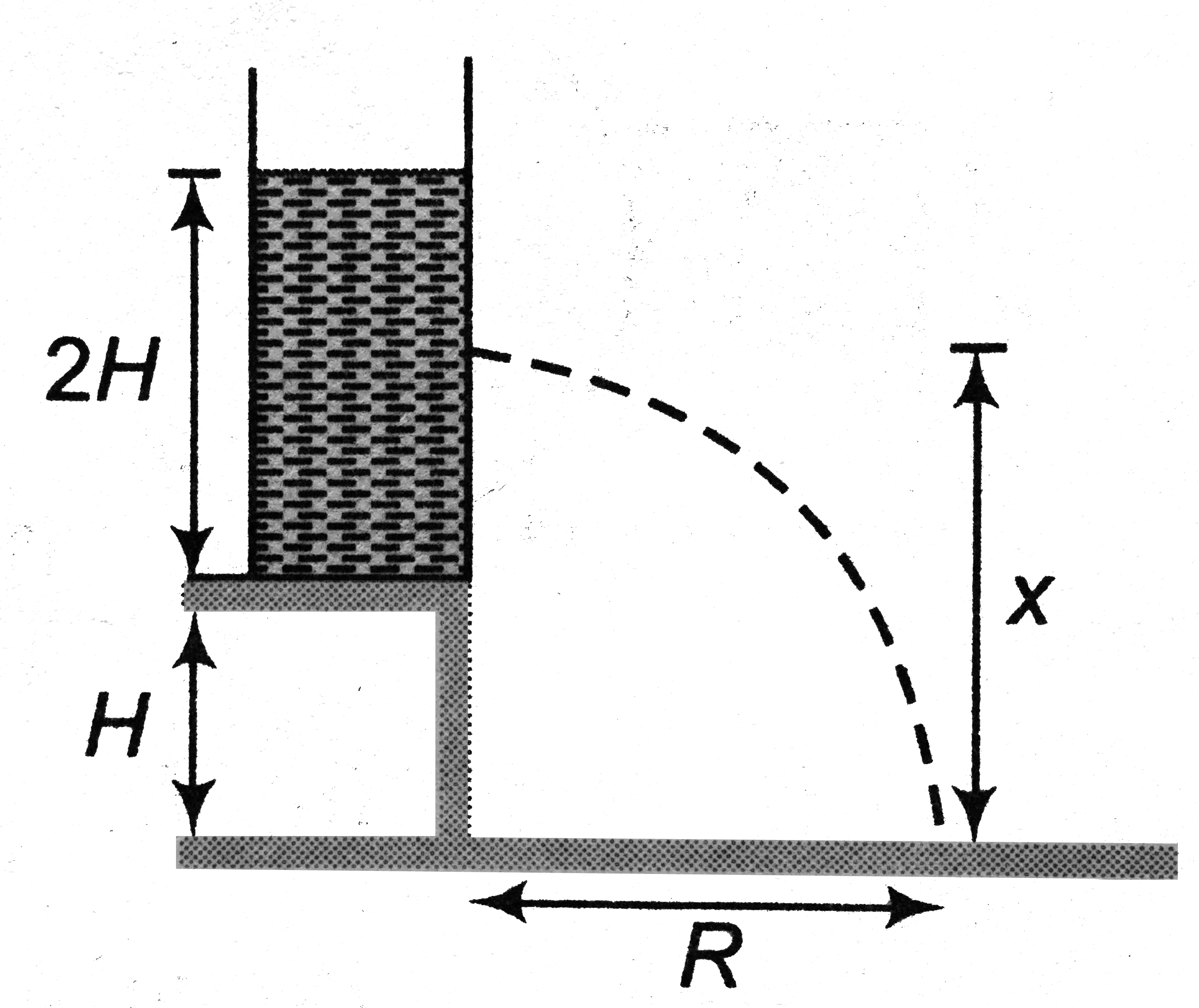 .
.
View SolutionIf a man of ,mass M jumps to the ground from a height h and his centre of mass moves a distance x in the time taken by him to 'hit' the ground the average force acting on him (assuming his retardation to be constant during his impact with the ground) is :
View SolutionA body of mass m falls from height h, on the ground, If e is the coefficient of restitution between body and the ground, then the height upto which it rises after ten collisions is
View SolutionA shell of mass 4kg moving with a velocoity 10m/s vertically upward explodes into three parts at a height 50m from ground. After three seconds, one part of mass 2kg reaches ground and another part of mass 1kg is at height 40m from ground. The height of the third part from the ground is : [g=10m/s2]
View SolutionA shell of mass 4kg moving with a velocoity 10m/s vertically upward explodes into three parts at a height 50m from ground. After three seconds, one part of mass 2kg reaches ground and another part of mass 1kg is at height 40m from ground. The height of the third part from the ground is : [g=10m/s2]
View SolutionA stone is dropped from a rising balloon at a height of 76 m above the ground and reaches the ground in 6s. What was the velocity of the balloon when the stone was dropped?
View Solutionporter lifts a luggage of 15 kg from the ground and puts it on his head 1.5m above the ground. Calculate the work done by him on the luggage
View SolutionWhen a person jumps from a tree to the ground what happens to the momentum of the person upon striking the ground ?
View Solution
