(a)Find the wavelength of the radiation required to excited the electron is Li++ from the first to the third Bohr orbit (b) How many spectral lines are obseved in the emission spactrum of the above excited system?
The energy of the electron in nth orbit of hydrogen-like atom is
En=−Z2Rhcn2 Here , Z=3
and Rhc=13.6eV
a. The energy required to excite the electron in Li++ from (n=1) to (n=3) is
E3−E1=−Z2Rhc32−(−Z2Rhc12)
=Z2Rhc(1−19)
89=Z2Rhc=89×(3)2×13.6eV
=8×13.6eV=8×13.6×1.6×10−19J
Therefore , the wavelength of incidiation radition required for excitation is given by
hcλ=E3−E1
or λ=hcE3−E1=6.63×10−34×3×1088×13.6×1.6×10−19
=114.26×10−10m−114.26Å
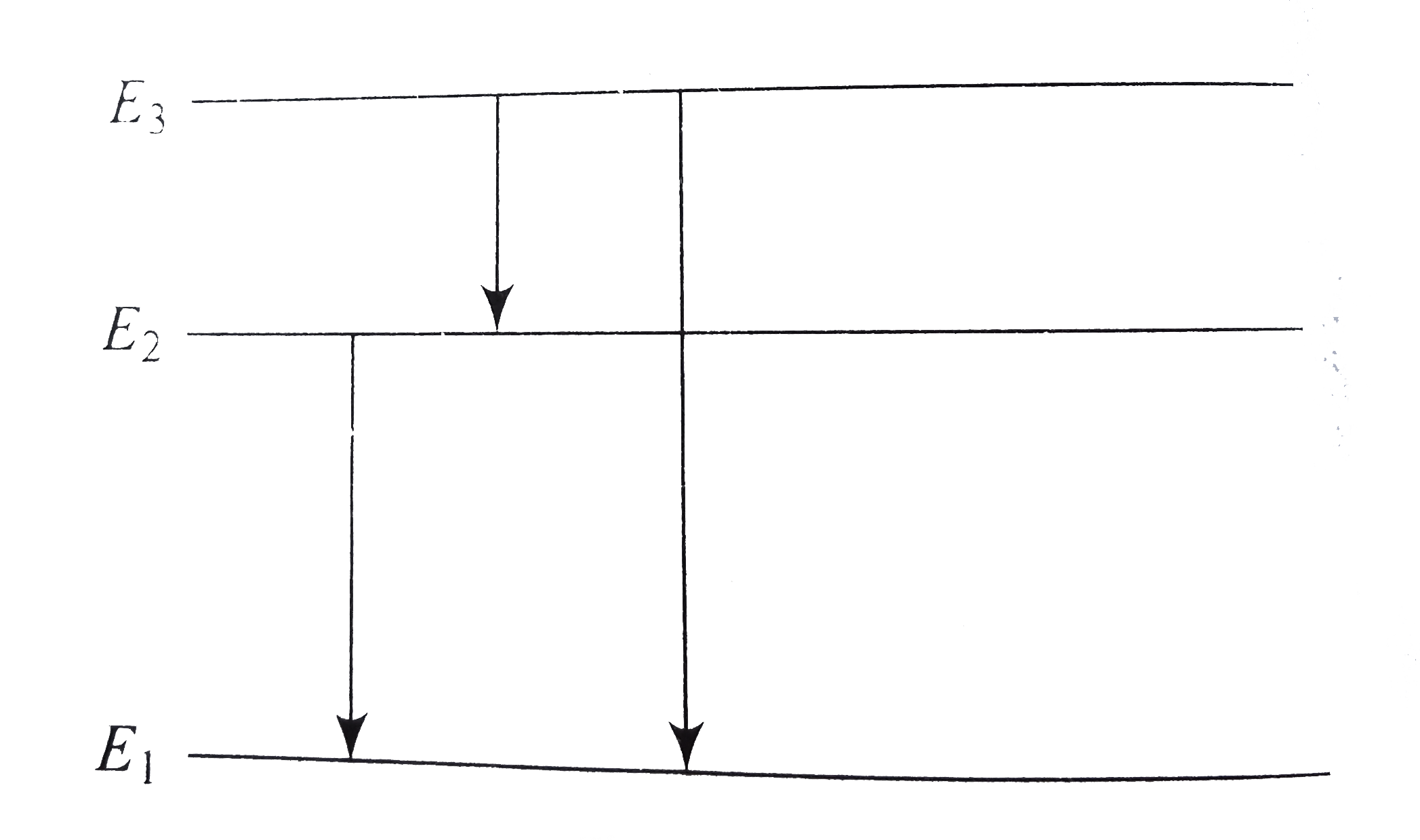
b. The possible lines in the emission spectrum of this excited system are [nn−12=33−12=3]
Three in number , represented in figure.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Exercise 4.115videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Exercise 4.212videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.315videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct10videos
ALTERNATING CURRENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer5videos
CAPACITOR AND CAPACITANCE
Similar Questions
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is:
View SolutionA doubly ionized lithium atom is hydrogen like with atomic number 3. Find the wavelength of the radiation required to excite the electron in Li++ from the first to the third Bohr orbit (ionization energy of the hydrogen atom equals 13.6 eV).
View SolutionA doubly ionized lithium atom is hydrogen like with atomic number 3. Find the wavelength of the radiation required to excite the electron in Li++ from to the third Bohr orbit (ionization energy of the hydrogen atom equals 13.6 eV).
View SolutionFind the wavelength of radiation required to excite an electron in the ground levelll of Li2+(z=3) in the third energy level
View SolutionA doubly ionized lithium atom is hydrogen like with atomic
. number 3. Find the wavelength of the radiation to excite the electron in
. Li++ form the first to the third Bohr orbit. The ionization energy of the hydrogen
. Atom is 13.6V.View SolutionThe wavelength of the radiation emitted when an electron falls from Bohr 's orbit 4 to 2 in H atom is
View SolutionThe ratio of the energy required to remove an electron from the first three Bohr's orbit of Hydrogen atom is
View SolutionA single electron orbit around a stationary nucleus of charge +Ze where Z is a constant and e is the magnitude of the electronic charge. It requires47.2eV to excite the electron from the second bohr orbit to the third bohr orbit. Find
(i) The value of Z
(ii) The energy required by nucleus to excite the electron from the third to the fourth bohr orbit
(iii) The wavelength of the electronmagnetic radiation required to remove the electron from the first bohr orbit to inlinity
(iv) The energy potential energy potential energy and the angular momentum of the electron in the first bohr orbit
(v) The radius of the first bohr orbit (The ionization energy of hydrogen atom =13.6eV bohr radius =5.3×10−11matre velocity of light =3×108m/sec planks 's constant =6.6×10−34 jules - sec )View SolutionWhat will be the wavelength of radiation released in nano-metre when an electron drops from the 5th Bohr orbit to 2nd Bohr orbit in hydrogen atom?
View SolutionA single electron orbits a stationary nucleus of charge +Ze, where Z is a constant and e is the magnitude of electronic charge . It requires 47.2eV to excite electron from second Bohr orbit to third Bhor orbit . Find
a the value of Z
b the energy required to excite the electron from the third to the fourth Bohr orbit.
c. the wavelength of electromagnetic rediation required to remove the electron from the first Bohr orbit to infinity.
d Find the KE,PE, and angular momentum of electron in the first Bohr orbit.
e. the redius of the first Bohr orbit
[The ionization energy of hydrogen atom =13.6eV Bohr radius =5.3×10_11m, "velocity of light" =3×10−8jms−1, Planck's constant =6.6×10−34j−s]View Solution

