The electrons in a H- atom kept at rest , jumps from the mth shell to the nth shell (m>n). Suppose instead of emitting electromagnetic wave, the energy released is converted into kinetic energy of the atom. Assuming Bohr's model and conservation of angular momentum are valid. Now , answer the following questions:
Calculate the angular velocity of the atom about the nucleus if l is the momentum of inertia
(m+n)6.28hl
(m+n)1.57hl
(m−n)6.28hl
(m−n)1.57hl
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Understand the Concept of Angular Momentum
In a hydrogen atom, the angular momentum L of an electron in a shell is given by the formula:
L=nh2π
where n is the principal quantum number of the shell, and h is Planck's constant.
Step 2: Calculate the Change in Angular Momentum
When the electron jumps from the m-th shell to the n-th shell, the change in angular momentum ΔL is given by:
ΔL=Ln−Lm=nh2π−mh2π=(n−m)h2π
Since m>n, we can express this as:
ΔL=(m−n)h2π
Step 3: Relate Angular Momentum to Moment of Inertia and Angular Velocity
According to the conservation of angular momentum, the angular momentum of the atom can also be expressed in terms of its moment of inertia I and angular velocity ω:
L=Iω
Given that the moment of inertia is represented as l, we have:
L=lω
Step 4: Set the Two Expressions for Angular Momentum Equal
Setting the two expressions for angular momentum equal gives us:
(m−n)h2π=lω
Step 5: Solve for Angular Velocity ω
Rearranging the equation to solve for ω:
ω=(m−n)h2πl
This simplifies to:
ω=(m−n)h2πl
Final Answer
Thus, the angular velocity ω of the atom about the nucleus is:
ω=(m−n)h2πl
---
In a hydrogen atom, the angular momentum L of an electron in a shell is given by the formula:
L=nh2π
where n is the principal quantum number of the shell, and h is Planck's constant.
When the electron jumps from the m-th shell to the n-th shell, the change in angular momentum ΔL is given by:
ΔL=Ln−Lm=nh2π−mh2π=(n−m)h2π
Since m>n, we can express this as:
ΔL=(m−n)h2π
According to the conservation of angular momentum, the angular momentum of the atom can also be expressed in terms of its moment of inertia I and angular velocity ω:
L=Iω
Given that the moment of inertia is represented as l, we have:
L=lω
Setting the two expressions for angular momentum equal gives us:
(m−n)h2π=lω
Rearranging the equation to solve for ω:
ω=(m−n)h2πl
This simplifies to:
ω=(m−n)h2πl
Thus, the angular velocity ω of the atom about the nucleus is:
ω=(m−n)h2πl
Topper's Solved these Questions
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Integer
4 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Fill In The Blanks
8 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Multiple Correct
13 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct
10 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
Similar Questions
The electrons in a H- atom kept at rest , jumps from the mth shell to the nth shell (m>n). Suppose instead of emitting electromagnetic wave, the energy released is converted into kinetic energy of the atom. Assuming Bohr's model and conservation of angular momentum are valid. Now , answer the following questions:
What principal is violated of inertia
View SolutionThe electrons in a H- atom kept at rest , jumps from the mth shell to the nth shell (m>n). Suppose instead of emitting electromagnetic wave, the energy released is converted into kinetic energy of the atom. Assuming Bohr's model and conservation of angular momentum are valid. Now , answer the following questions:
If the above comprehension be true , ehat is not valid here?
View SolutionAn electron in an H-atom kept at rest , jumps from the mth shell to the nth shell (m>n). Suppose instead of emitting electromagnetic wave, the energy reased is converted into the kinetic energy of the atom. Assume the Bohr model and conservation of angular momentum are valid. If I is the moment of inertia the angular velocity of the atom about the nucleus is 4(m−n)h/kI. Calculate k
View Solution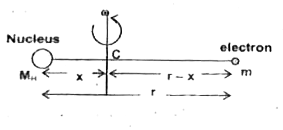
The angular momentum of the atom about the centre of mass will be
View SolutionIn an atom when an electron jumps from K-shel to M-shell
View SolutionAn electron in H atom jumps from the third energy level to the first energy .The charge in the potential energy of the electron is
View SolutionIf the magnitude of energy of the electron in nth Bohr orbit is EnandJn is its angular momentum , then
View SolutionThe energy of an excited H-atom is −3.4eV. Calculate angular momentum of e−
View SolutionThe electron in a hydrogen atom makes a transition from M shell to L-shell. The ratio of magnitude of initial to final acceleration of the electron is
View SolutionThe electron in a hydrogen atom makes a transition from M shell to L-shell. The ratio of magnitude of initial to final acceleration of the electron is
View Solution