A source of sonic oscillation with frequency n0=600Hz moves away and at right angles to a wall with velocity u=30ms. A stationary reciever is located on the line of source in succession wall→source→receiver. If velocity of osund propagation is v=330ms, then
Q. The wavelength of reflected waves received by the receiver is
(a)120cm
(b)50cm
(c)90cm
(d)60cm
The correct Answer is:D
To solve the problem step by step, we will start by calculating the frequency of the reflected wave and then find the wavelength of that wave.
Step 1: Calculate the frequency of the reflected wave (n_r)
The formula for the frequency of the reflected wave when the source is moving away from the wall is given by:
nr=n0×vv+u
Where:
- n0=600Hz (frequency of the source)
- v=330m/s (velocity of sound)
- u=30m/s (velocity of the source)
Substituting the values into the formula:
nr=600×330330+30
Calculating the denominator:
330+30=360
Now substituting back into the equation:
nr=600×330360
Calculating the fraction:
330360=1112
Now substituting this back:
nr=600×1112=550Hz
Step 2: Calculate the wavelength of the reflected wave (λ)
The wavelength can be calculated using the formula:
λ=vnr
Where:
- nr=550Hz
Substituting the values into the formula:
λ=330550
Calculating the fraction:
λ=35m
Step 3: Convert the wavelength to centimeters
To convert the wavelength from meters to centimeters, we multiply by 100:
λ=35×100=60cm
Final Answer
The wavelength of the reflected waves received by the receiver is 60 cm.
---
- n0=600Hz (frequency of the source)
- v=330m/s (velocity of sound)
- u=30m/s (velocity of the source)
- nr=550Hz
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Assertion-Reasoning24videos
SOUND WAVES AND DOPPLER EFFECT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger2videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type5videos
SUPERPOSITION AND STANDING WAVES
Similar Questions
A source of sonic oscillation with frequency n0=600Hz moves away and at right angles to a wall with velocity u=30ms. A stationary reciever is located on the line of source in succession wall→source→receiver. If velocity of osund propagation is v=330ms, then
Q. The wavelength of direct waves received by the receiver isView SolutionA source of sonic oscillation with frequency n0=600Hz moves away and at right angles to a wall with velocity u=30ms. A stationary reciever is located on the line of source in succession wall→source→receiver. If velocity of osund propagation is v=330ms, then
Q. The beat frequency recorded by the receiver isView SolutionA source of sonic oscillations with frequency v0=100Hz moves at right angles to the wall with a velocity u=0.17m/s. Two stationary receivers R1 and R2 are located on a straight line, coinciding with the trajectory of the source , in the following succession :R1− source −R2− wall. Which receiver registers the beatings and what is the beat frequency ? The velocity of sound is equal to υ=340m/s.
View SolutionA source of sonic oscillations with frequency v0=100Hz moves at right angles to the wall with a velocity u=0.17m/s. Two stationary receivers R1 and R2 are located on a straight line, coinciding with the trajectory of the source , in the following succession :R1− source −R2− wall. Which receiver registers the beatings and what is the beat frequency ? The velocity of sound is equal to υ=340m/s.
View SolutionA source of sound of frequency 256 Hz moves rapidly towards a wall with a velocity of 5ms−1. How many beats per second will be heard if sound travels at a speed of 330 m/s?
View SolutionA source of sonic oscillations with frequency n=1700Hz and a receiver are located on the same normal to a wall. Both the source and receiver are stationary, and the wall recedes from the source with velocity u=6.0ms. Find the beat frequency registered by the receiver. The velocity of sound is v=340ms.
View SolutionA source of sonic oscillations with frequency n=1700Hz and a receiver are located on the same normal to a wall. Both the source and receiver are stationary, and the wall recedes from the source with velocity u=6.0ms. Find the beat frequency registered by the receiver. The velocity of sound is v=340ms.
View SolutionA source of sound which emitting a sound of frequency 600 Hz is moving towards a wall with a velocity 30 m/s. Three observes A, B, C moving with velocity 20 m/s, A away from the wall and B towards the wall and Inside the source, Velocity of sound in air is 330 m/s.

Find the frequency of reflected sound observed by observer BView SolutionA source of sound of frequency 256Hz is moving rapidly towards wall with a velocity of 5m/sec. How many beats per second will be heard if sound travels at a speed of 330m/sec.
View SolutionA car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
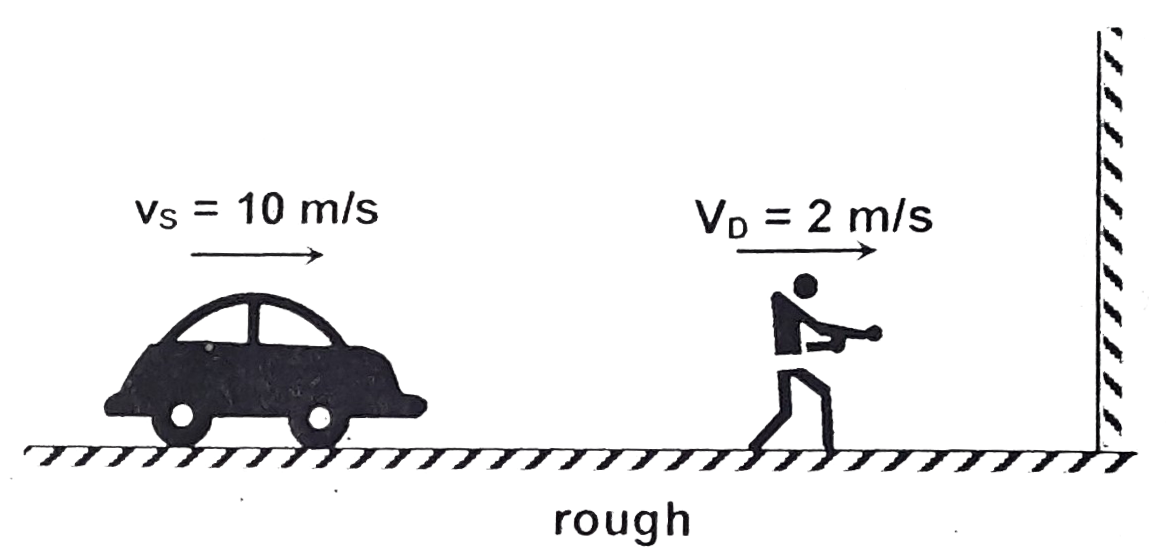 View Solution
View Solution

