A heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross section 100cm2. The thermal conductivity of copper is 400W/m/∘C The two ends of this rod must be kept at a temperature difference of
1∘C
10∘C
100∘C
1000∘C
The correct Answer is:C
To solve the problem of finding the temperature difference required to pass a heat flux of 4000 J/s through a copper rod, we can use the formula for heat conduction, which is derived from Fourier's law:
dQdt=k⋅A⋅ΔTΔx
Where:
- dQdt is the heat flux (in watts, which is equivalent to J/s),
- k is the thermal conductivity of the material (in W/m·°C),
- A is the cross-sectional area (in m²),
- ΔT is the temperature difference (in °C),
- Δx is the length of the rod (in meters).
1. Identify the given values:
- Heat flux, dQdt=4000J/s
- Length of the rod, Δx=10cm=0.1m
- Area of cross-section, A=100cm2=100×10−4m2=0.01m2
- Thermal conductivity of copper, k=400W/m⋅°C
2. Rearrange the formula to solve for ΔT:
ΔT=dQdt⋅Δxk⋅A
3. Substitute the values into the equation:
ΔT=4000J/s⋅0.1m400W/m⋅°C⋅0.01m2
4. Calculate the denominator:
k⋅A=400⋅0.01=4W/°C
5. Now substitute this back into the equation:
ΔT=4000⋅0.14
6. Perform the calculations:
ΔT=4004=100°C
Final Answer:
The temperature difference required across the ends of the copper rod is 100°C.
---
- dQdt is the heat flux (in watts, which is equivalent to J/s),
- k is the thermal conductivity of the material (in W/m·°C),
- A is the cross-sectional area (in m²),
- ΔT is the temperature difference (in °C),
- Δx is the length of the rod (in meters).
- Heat flux, dQdt=4000J/s
- Length of the rod, Δx=10cm=0.1m
- Area of cross-section, A=100cm2=100×10−4m2=0.01m2
- Thermal conductivity of copper, k=400W/m⋅°C
ΔT=dQdt⋅Δxk⋅A
ΔT=4000J/s⋅0.1m400W/m⋅°C⋅0.01m2
k⋅A=400⋅0.01=4W/°C
ΔT=4000⋅0.14
ΔT=4004=100°C
The temperature difference required across the ends of the copper rod is 100°C.
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Multiple Correct
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Comprehension
30 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Subjective
25 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
The ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK

View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionFigure shows an aluminium rod joined to a copper rod. Each of the rods has a length of 20cm and area of cross section 0.20cm2 . The junction is maintained at a constant temperature 40∘C and the two ends are maintained at 80∘C . Calculate the amount of heat taken out from the cold junction in one minute after the steady state is reached. The conductivities are KAl=200Wm−1C−1 . and KCu=400Wm−1C−1 .

View SolutionFigure shows a steel rod joined to a brass rod. Each of the rods has length of 31cm and area of cross-section 0.20cm2. The junction is maintained at a constant temperature 50∘C and the two ends are maintained at 100∘C. The amount of heat taken out from the cold junction in 10 minutes after the steady state is reached in n×102J. Find 'n' the thermal conductivities are Ksteel=46W/m−∘C and Kbrass=109W/m−∘C.
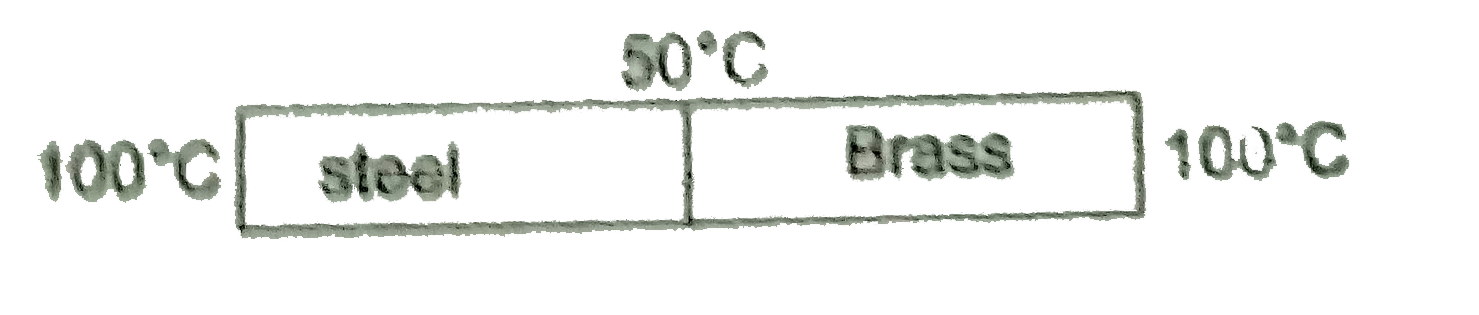
View Solution
As shown in Fig. AB is rod of length 30 cm and area of cross section 1.0cm2 and thermal conductivity 336 SI units. The ends A and B are maintained at temperatures 20∘C and 40∘C, respectively .A point C of this rod is connected to a box D, containing ice at 0∘C through a highly conducting wire of negligible heat capacity. The rate at which ice melts in the box is (assume latent heat of fusion for ice Lf=80cal/g)
View Solution
As shown in Fig. AB is rod of length 30 cm and area of cross section 1.0cm2 and thermal conductivity 336 SI units. The ends A and B are maintained at temperatures 20∘C and 40∘C, respectively .A point C of this rod is connected to a box D, containing ice at 0∘C through a highly conducting wire of negligible heat capacity. The rate at which ice melts in the box is (assume latent heat of fusion for ice Lf=80cal/g)
View SolutionOne end of a copper rod of length 1.0 m and area of cross-section 10−3 is immersed in boiling water and the other end in ice. If the coefficient of thermal conductivity of copper is 92cal/m−s−.∘C and the latent heat of ice is 8×104cal/kg, then the amount of ice which will melt in one minute is
View SolutionOne end of a copper rod of length 1 m and area of cross - section 400×(10−4)m2 is maintained at 100∘C. At the other end of the rod ice is kept at 0∘C. Neglecting the loss of heat from the surrounding, find the mass of ice melted in 1h. Given, KCu=401W/m−KandLf=3.35×(105)J/kg.
View SolutionA rod of negligible heat capacity has a length of 50 cm, area of cross section 5cm2 and thermal conductivity 500 W/m∘C. The temperature of one end is maintained at 0∘C and that fo the other end is slowly and linearly varied from 0∘C to 60∘C in 20 min. Assuming no heat loss through the lateral side, the total heat transmitted throught he rod in 20 minutes is
View Solution
