Water at 0∘C was heated until it started to boil and then until it all changed to steam. The time required to heat water from 0∘C to 100∘C is 5 min and the time to change boiling water to steam is 28 minutes. If the flame supplied heat at a constant rate, the specific latent heat of vaporization of water (neglecting heat losses, container etc. is (in J/g). (specific heat of water s =4.2J/g )
540
2268
2352
2356
The correct Answer is:C
To find the specific latent heat of vaporization of water, we can follow these steps:
Step 1: Calculate the total heat supplied to raise the temperature of water from 0°C to 100°C.
The formula for heat supplied (Q) is given by:
Q=m⋅s⋅ΔT
Where:
- m = mass of water (in grams)
- s = specific heat of water = 4.2 J/g°C
- ΔT = change in temperature = 100°C−0°C=100°C
So, the heat supplied to raise the temperature from 0°C to 100°C is:
Q1=m⋅4.2⋅100
Q1=420mJ
Step 2: Calculate the total heat supplied to convert boiling water to steam.
The heat required to convert boiling water to steam is given by:
Q=m⋅L
Where:
- L = specific latent heat of vaporization (in J/g)
The time taken to convert boiling water to steam is 28 minutes, which is:
t2=28×60=1680s
The heat supplied during this time is:
Q2=P⋅t2
Where P is the power (rate of heat supply).
Step 3: Relate the power to the heat supplied.
The power can also be expressed in terms of the heat supplied to raise the temperature:
P=Q1t1
Where t1=5×60=300s (time to heat water from 0°C to 100°C).
Thus:
P=420m300
P=1.4mW
Step 4: Substitute power into the heat equation for boiling water to steam.
Using the expression for power in the heat equation for boiling water to steam:
Q2=P⋅t2
Q2=(1.4m)⋅(1680)
Q2=2352mJ
Step 5: Set the two expressions for heat equal to each other.
From the heat required to convert boiling water to steam:
Q2=m⋅L
So we have:
2352m=m⋅L
Step 6: Solve for the specific latent heat of vaporization (L).
Dividing both sides by m (assuming m≠0):
L=2352J/g
Final Answer:
The specific latent heat of vaporization of water is 2352J/g.
---
Q=m⋅s⋅ΔT
Where:
- m = mass of water (in grams)
- s = specific heat of water = 4.2 J/g°C
- ΔT = change in temperature = 100°C−0°C=100°C
Q1=m⋅4.2⋅100
Q1=420mJ
Q=m⋅L
Where:
- L = specific latent heat of vaporization (in J/g)
t2=28×60=1680s
Q2=P⋅t2
Where P is the power (rate of heat supply).
P=Q1t1
Where t1=5×60=300s (time to heat water from 0°C to 100°C).
P=420m300
P=1.4mW
Q2=P⋅t2
Q2=(1.4m)⋅(1680)
Q2=2352mJ
Q2=m⋅L
So we have:
2352m=m⋅L
L=2352J/g
The specific latent heat of vaporization of water is 2352J/g.
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Multiple Correct
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Comprehension
30 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Subjective
25 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
Due to thermal expansion with rise in temperature:
View SolutionA body cools in a surrounding of constant temperature 30∘C Its heat capacity is 2J/∘C. Initial temperature of cooling is valid. The body of mass 1 kg cools to 38∘C in 10 min
When the body temperature has reached 38∘C, it is heated again so that it reaches 40∘C in 10 min. The heat required from a heater by the body is
View SolutionA lead ball at 25∘C is dropped from a height of 2 km. It is heated due to air resistance and it is assumed that all of its kinetic energy is used in increasing the temperature of ball. Find the final temperature of the ball.
View SolutionThe displacement of a particle is given by y=(6t2+3t+4)m, where t is in seconds. Calculate the instantaneous speed of the particle.
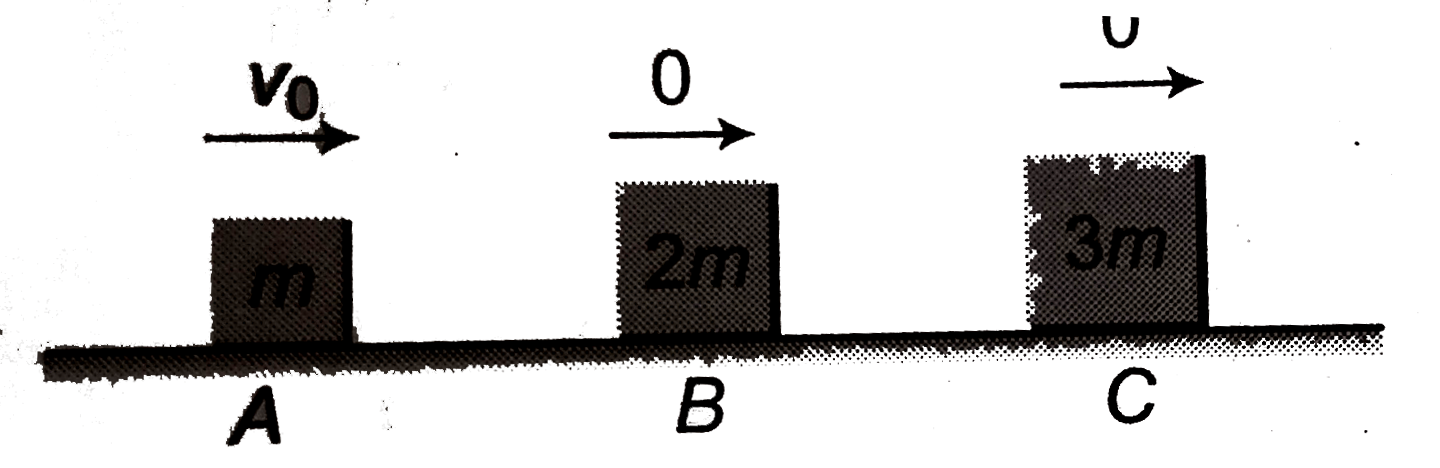
View SolutionThree objects A ,B and C are kept in a straight line on a smooth horizontal surface. These have masses m, 2m and 3m , respectively . The head - on elastic collision takes place between A and B and then B makes completely inelastic collision with C. All motions occur on the same straight line. The final speed of C will be
View SolutionWater at 0^@C was heated until it started to boil and then until it all changed to steam. The time required to heat water from 0^@C to 100^@C is 5 min and the time to change boiling water to steam is 28 minutes. If the flame supplied heat at a constant rate, the specific latent heat of vaporisation of water (neglecting heat losses, container etc. is (in J//g ). (specific heat of water s =4.2 J//g )
View SolutionBoiling water is changing into steam. Under this condition the specific heat of water is
View SolutionCalculate the amount of heat required to convert 10g of water at 30^(@)C into steam at 100^(@)C . ( Specific latent heat of vaporization of water = 540 cal//g )
View SolutionCalculate the amount of heat required to convert 10g of water at 30∘C into steam at 100∘C . ( Specific latent heat of vaporization of water =540cal/g )
View SolutionBoiling water is changing into steam.Under this condition the specific heat of water is ……………. .
View SolutionBoiling water is changing into steam, under this condition the specific heat of water in cals/g/∘C is:
View SolutionAn electric kettle takes 20 min. to heat a certain quantity of water from 0∘C to boiling point. It requires 90 minutes to turn all the water at 100∘C into steam. Find the latest heat of vaporisation.
(specific heat of water= 1 cal/gm/∘C)
View SolutionBoiling water is changing into steam. Under this condition the specific heat of water is
View SolutionBoiling water is changing into steam. The specific heat of boiling water is
View SolutionBoiling water is changing into steam. Under this condition the specific heat of water is
View Solution
