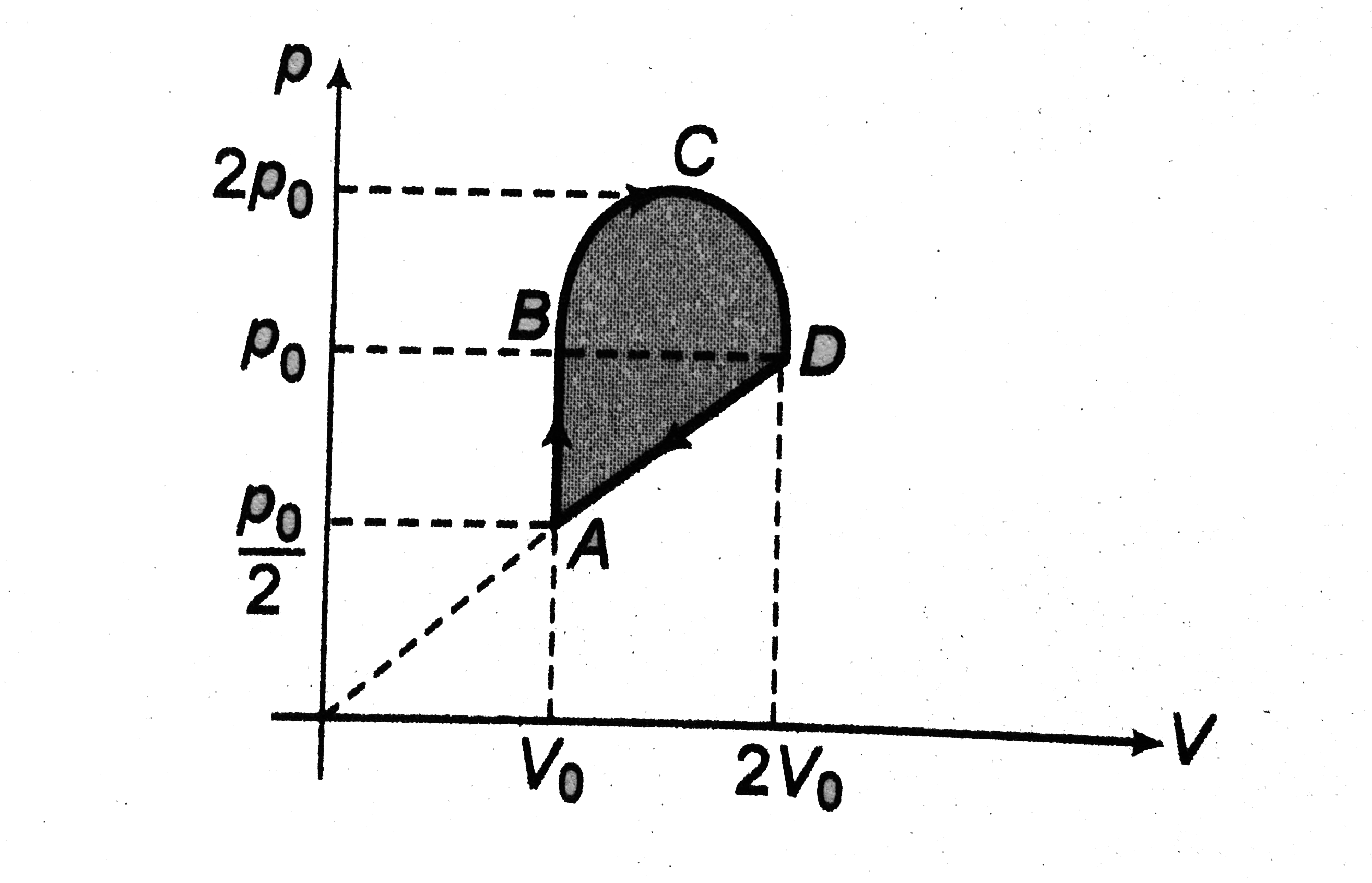
A monatomic ideal gas undergoes the shown cyclic process in which path of the process 2→3 is a semicircle. If 2mol of gas is taken, find

Total heat rejected in one cycle
P0V0(32−π)4
P0V0(32+π)4
P0V0(32+π)8
P0V0(32−π)8
The correct Answer is:D
Process 1→2 ( Isochoric process )
W12=0
Q12=ΔU12=nCV(T2−T1)
=n32R(T2−T1)
=32[P0V0−(P0/2)V0]
=3P0V04 (heat absorbed )
Process 3→1
W3→1= Area under process 3→1
=−(P02+P0).V02=−3P0V04
Work done during 3→1 will be negative as volume is decreasing.
ΔU3→1=n3R2(T1−T2)32(P0V02−P02V0)=−9P0V04
Q3→1=ΔU3→1+W3→1=−3P0V0 ( heat rejected )
process 2→3
The temperature will be maximum at x=2P0,Tx=3P0V02R
Wr→x=Wx→3=14πP0V02+P0V02=P0V02(π4+1)
W2rar3=P0V04(π+4)
ΔU2→x=n32R(Tx−T2)=32(3P0V0−P0V0)=3P0V0
ΔUx→3=n32R(Tx−T2)=32(2P0V0−3P0V0)
=−32P0V0
∴ΔQ2→x=ΔU2→x+ΔW2→x
=3P0V0+P0V02(π4+1)
=P0V0(3+12+π2)=P0V02(7+π4)
ΔQx→3=ΔUx→3+Wx→3
=−32P0V0+P0V02(π4+1)=−P0V02(2−π4)
Total heat rejected in one cycle
=3P0V0+P0V02(2−π4)=4P0V0−πP0V08
=P0V0(4−π8)=P0V0(32−π)8
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Assertion-Reasoning
6 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single correct anwer type
14 Videos
Similar Questions
A monatomic ideal gas undergoes the shown cyclic process in which path of the process 2→3 is a semicircle. If 2mol of gas is taken, find

Heat given to the system in semicircular process
View SolutionAn ideal gas undergoes the cyclic process shown in a graph below :
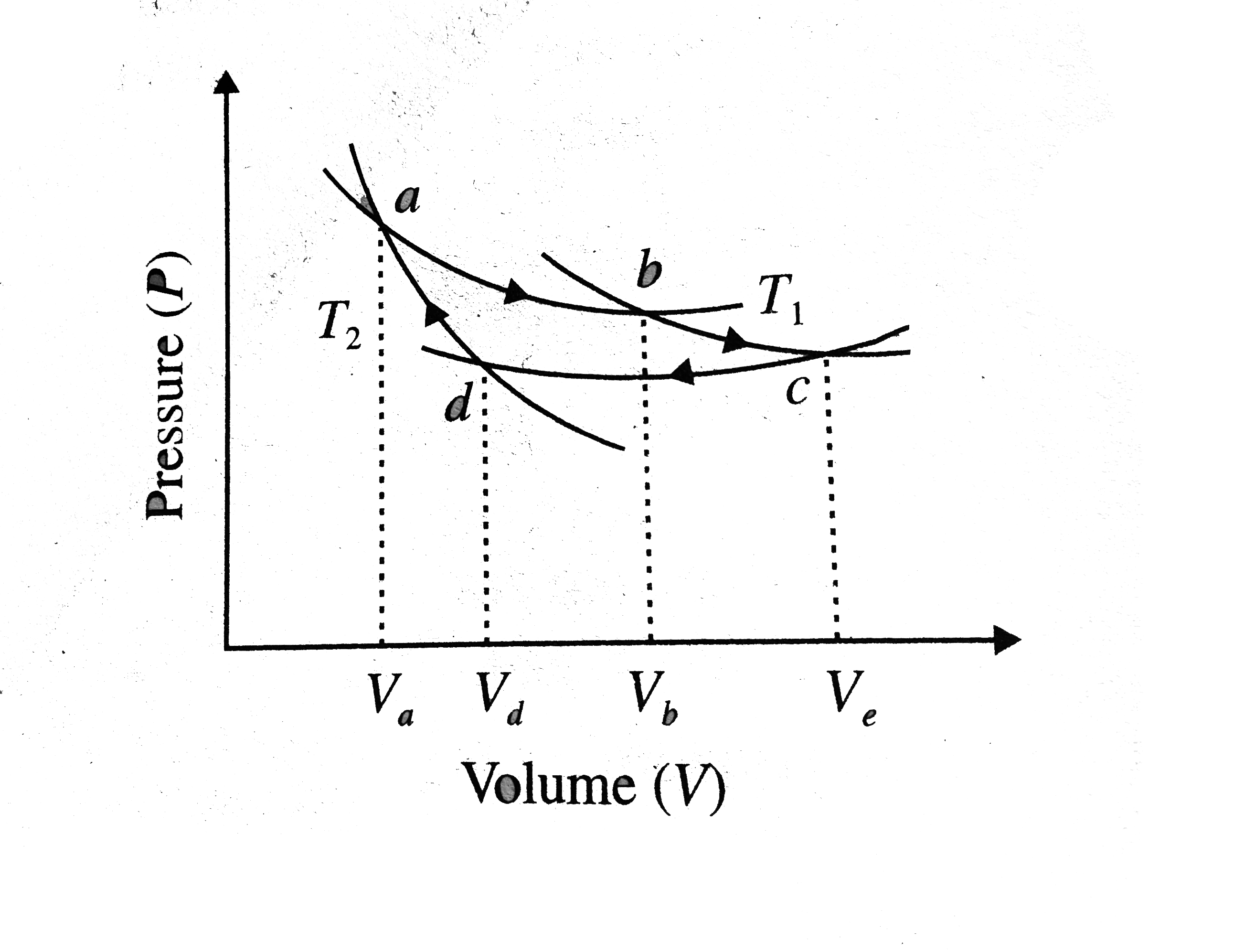
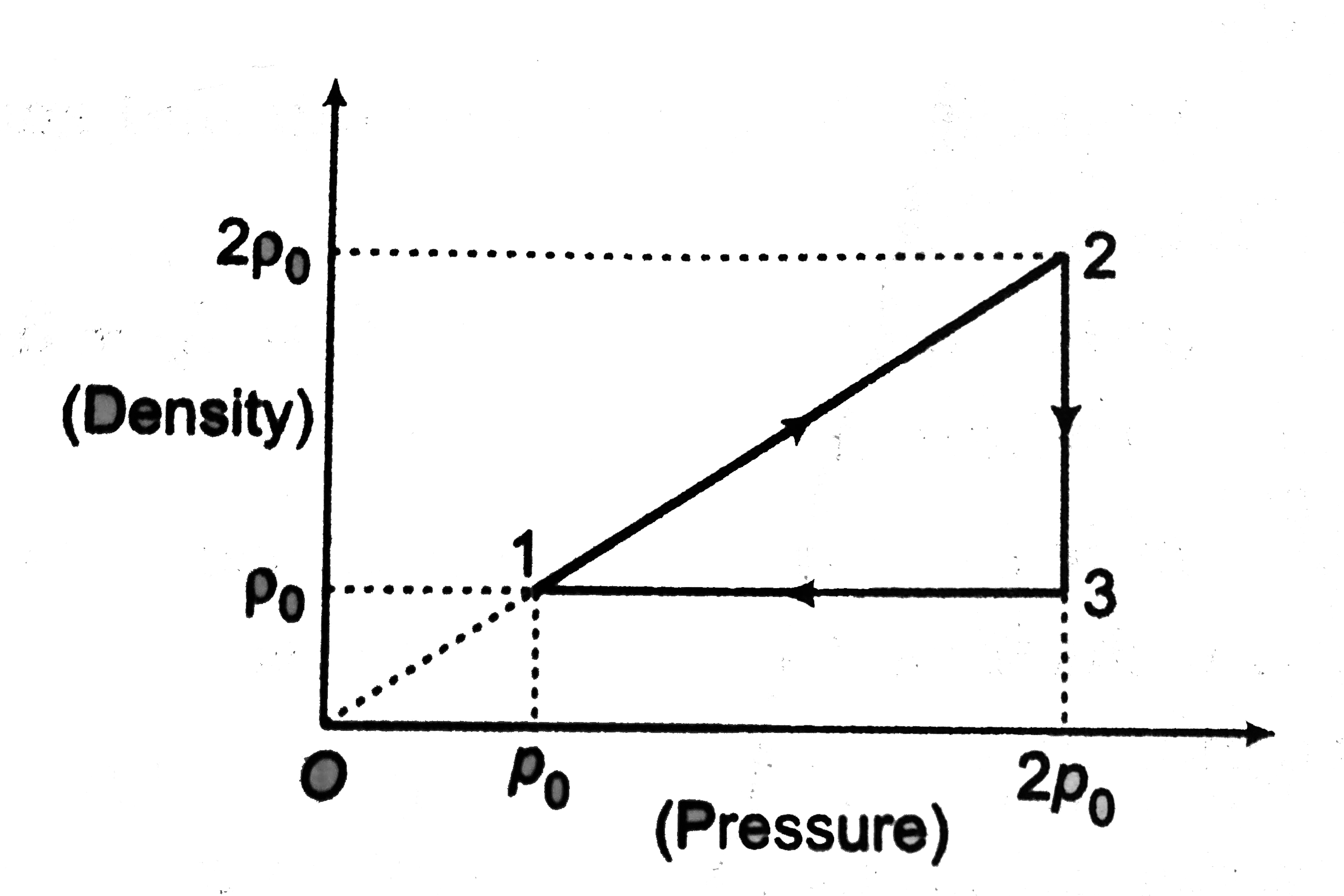
View SolutionAn ideal gas undergoes a cyclic process abcda which is shown by pressure-density curve,

View SolutionAn ideal gas undergoes a cyclic process abcda which is shown by pressure-density curve,

View SolutionTwo moles of a monatomic ideal gas undergo a cyclic process ABCDA as shown in figure. BCD is a semicircle. Find the efficiency of the cycle.
View SolutionThe density (ρ) versus pressure (p) graph of one mole of an ideal monoatomic gas undergoing a cyclic process is shown in figure. Molecular mass of gas is M.
(a) Find work done in each process.
(b) Find heat rejected by gas in one complete cycle.
(c) Find the efficiency of the cycle.
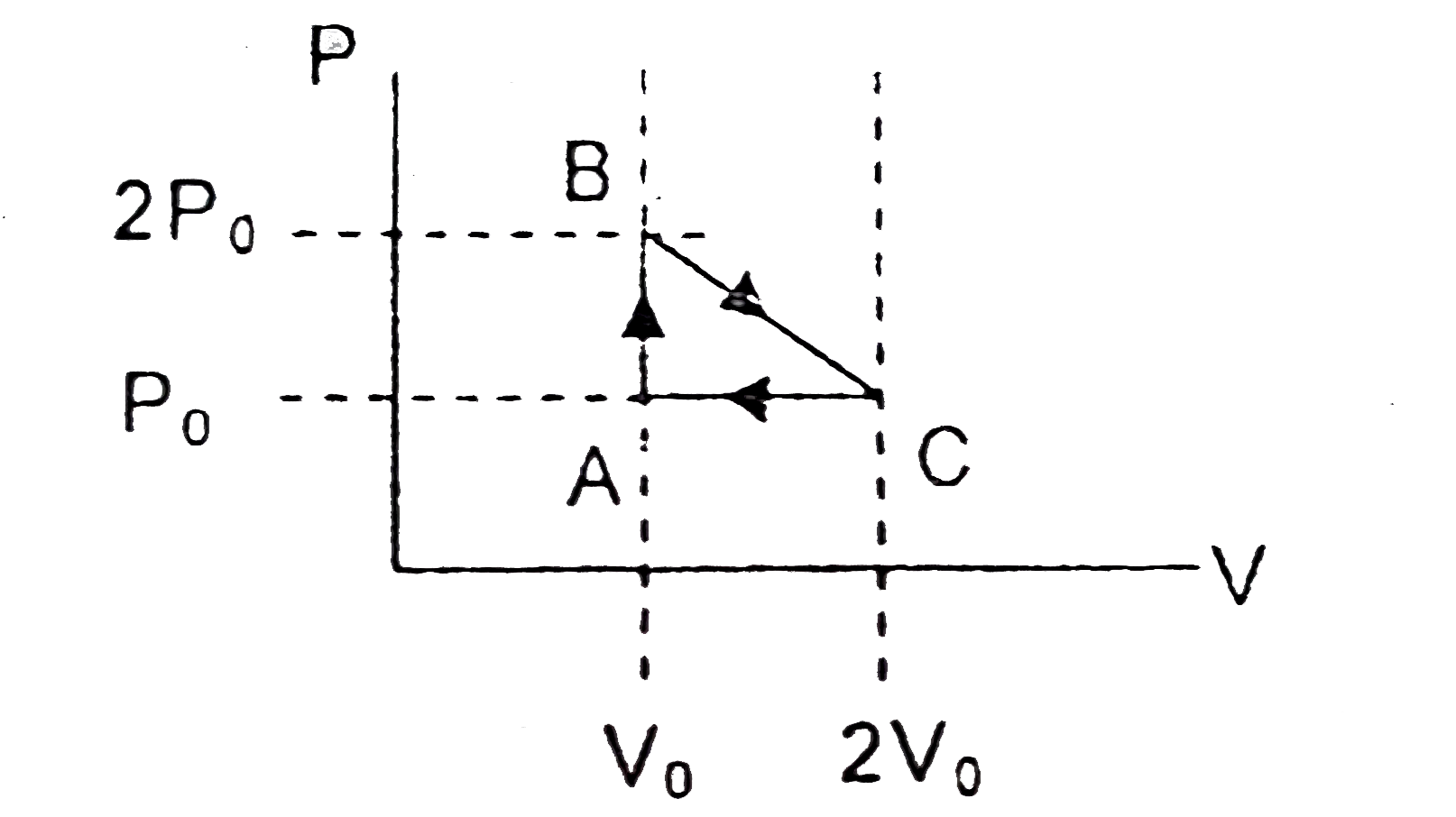
View SolutionA sample of helium is undergoing a cyclic process ABCA as shown. In which of the following processes heat is a absorbed by the gas?
View SolutionOne mole of monoatomic gas is taken round the cyclic process. Heat absorbed by gas in process AB is
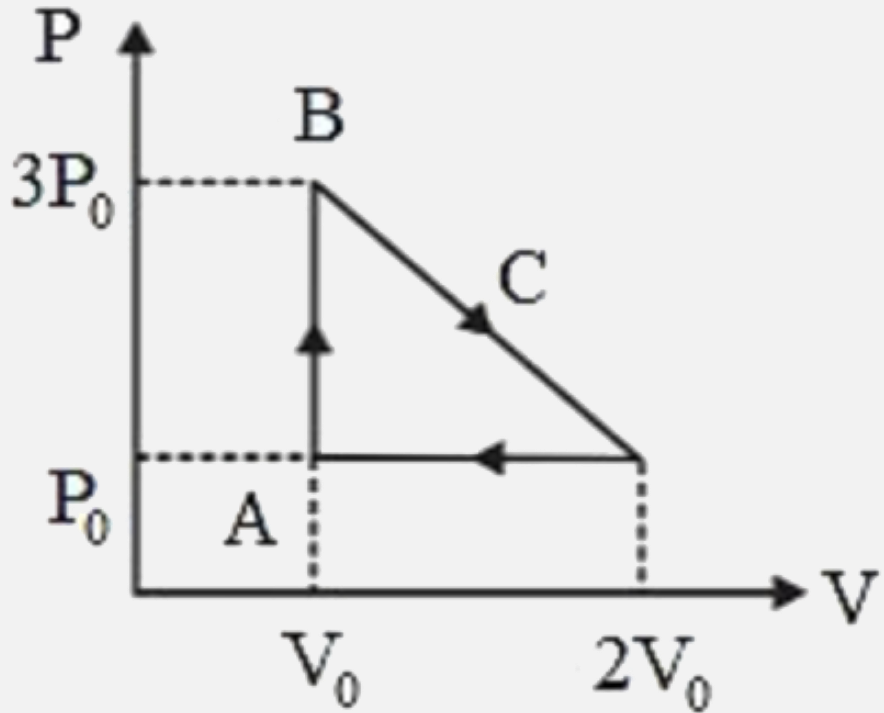
View Solution
An ideal gas undergoes a cyclic process, in which one process is isochoric, one process is isothermal and one process is adiabatic. During the isothermal process, 40 J heat is released by the gas, and during the isochoric process, 80 J heat is absorbed by the gas. if work done by the gas during adiabatic process is W1 and during isothermal process is W2 then the magnitude of W1W2 will be equal to:
View SolutionOne mole of an ideal monoatomic gas undergoes a cyclic process, as shown in the figure. If the temperature of the gas at state 1 is 300 K and at state 4 is 500 K, then heat exchanged during process 2→3, is
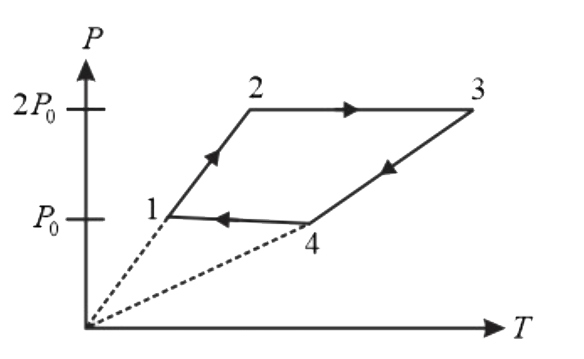
View Solution