The figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
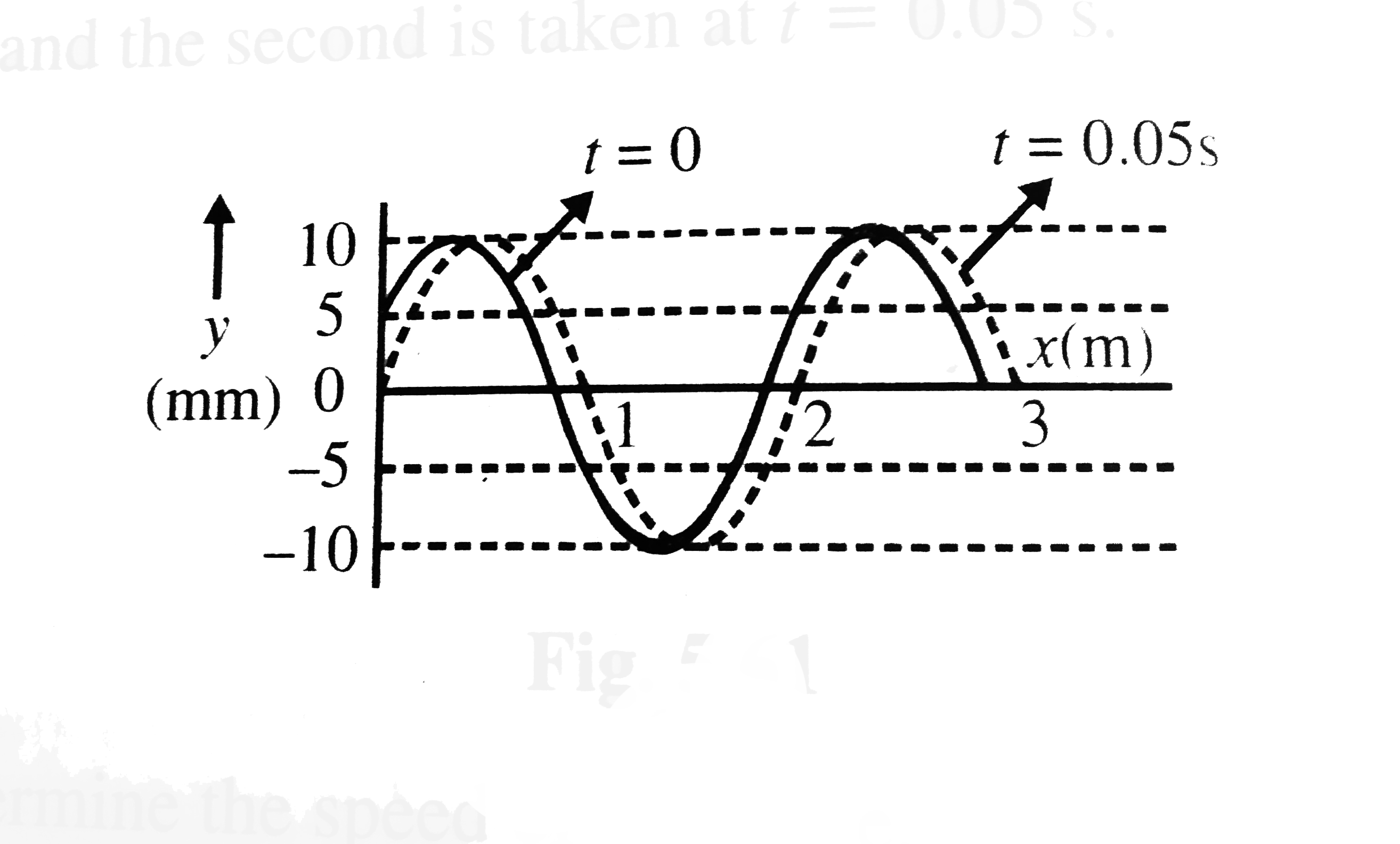
Determine the equation of the wave.
y10sin2πx−10/3πtπ/6
yπ10sinππx−π10/3ππtπππ/3ππ
yπ10sinππx−π10/3ππtπππ/6ππ
yπ10sinππx−π5/3ππtπππ/3ππ
The correct Answer is:c
The waves is travelling along the positivex-axis
∴y=Asin[kx−ωt+ϕ]
at x=0,y=Asin[−ωt+ϕ]
Also, at t=0,y=Asinϕ=A/2,
sinϕ=1/2
⇒ϕ=(π/6),(5π/6),…
and at t=0.05s,y=Asin(−ω20+ϕ)=0
or, −ω20+ϕ=0,π,2π,...
For t=0.05s and x=1m,
y=Asin(k−ω20+ϕ)=0
since, λ=2m
∴π−ω20+ϕ=0,π,2π,...
from Eqs. (i),(ii) and (ii), we get
ϕ=π/6
ϕ−ω20=0
ω=10π3
f=ω2π=53Hz
velocity of wave is
V=λf=(2)(5/3)=10/3m/s
Maximum velocity of the particle is
Vmax=oemgaA)10π/3))(10x10−3)=π/30m/s
Tension in the string is
T=μV2=(0.25)(10/3)2=25/9N.
the equation of the wave is
y=10sin[πx−(10/3)πt+(π/6)]
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning
6 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
The figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
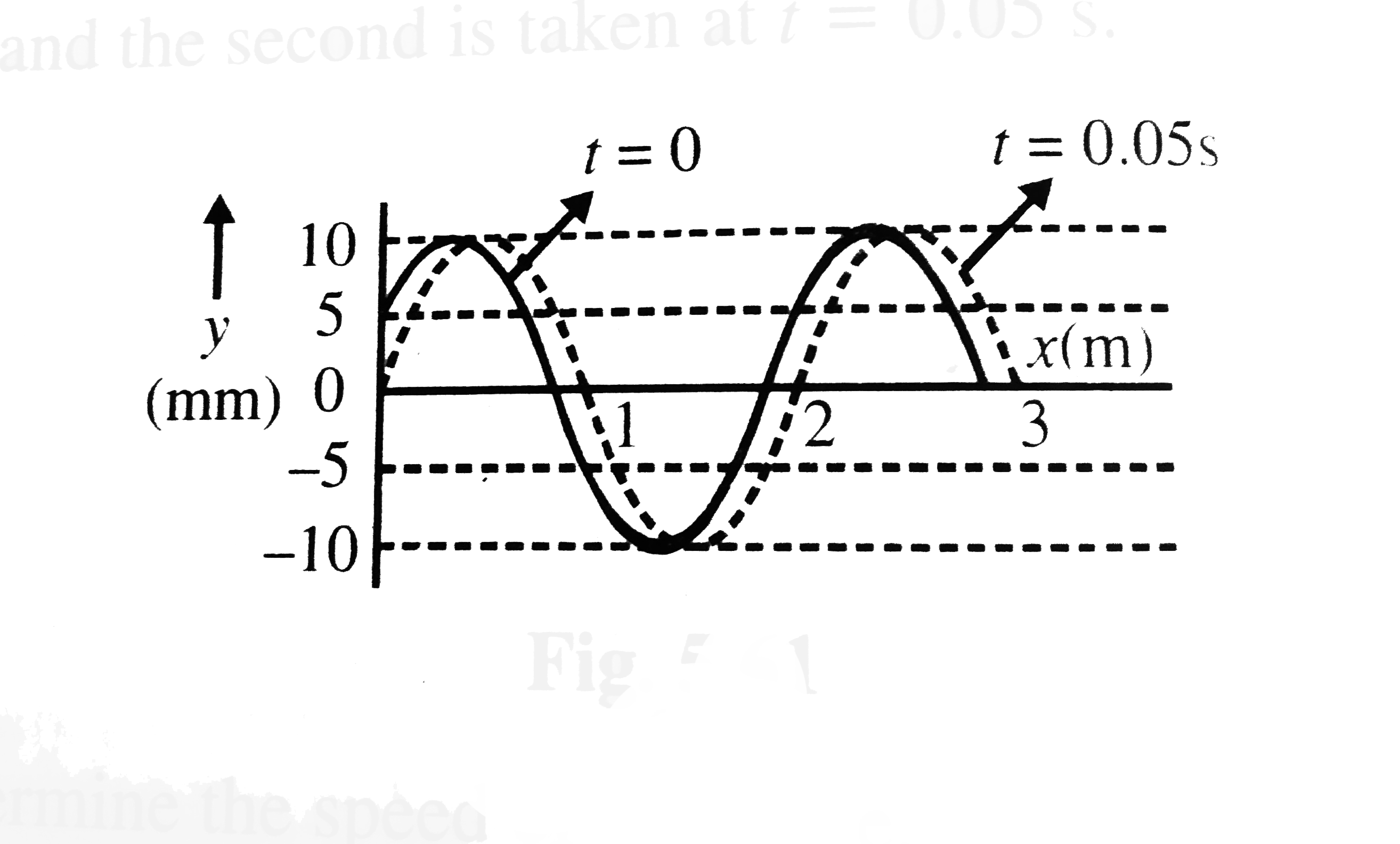
Determine the frequency of the wave.
View SolutionThe figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
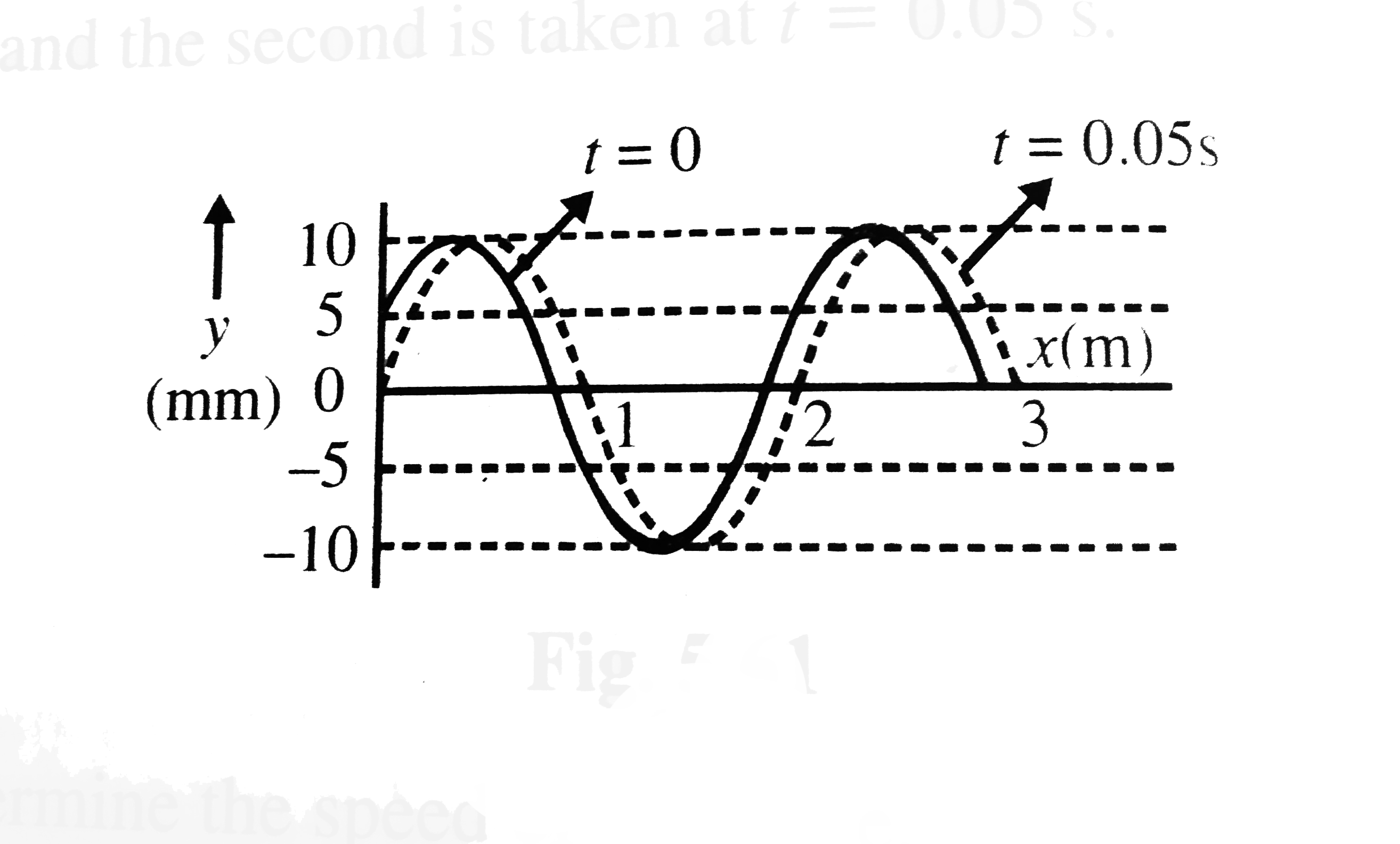
Determine the speed of the wave. Determine the frequency of the wave.
View SolutionThe figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
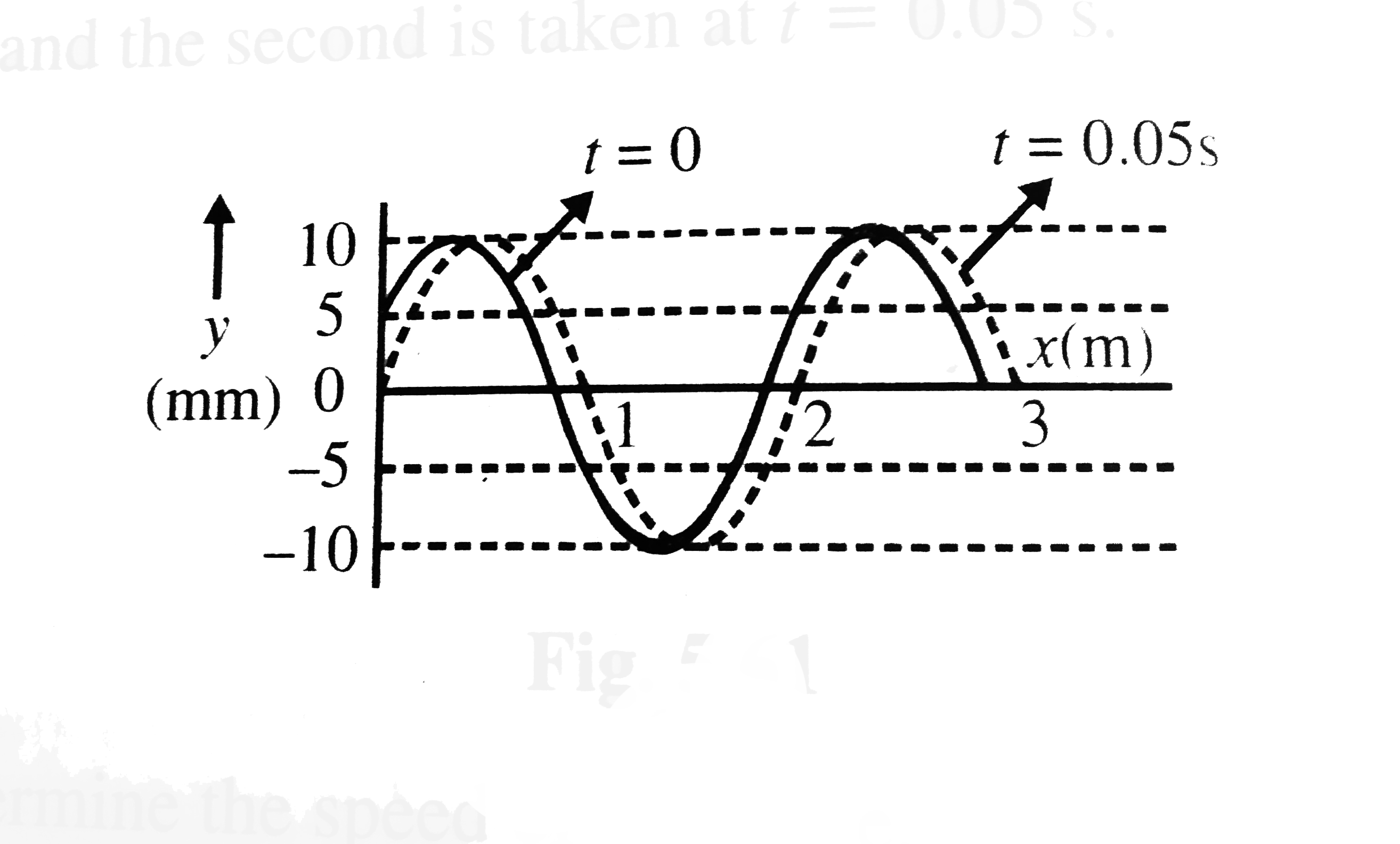
Determine the speed of the wave.
View SolutionThe figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
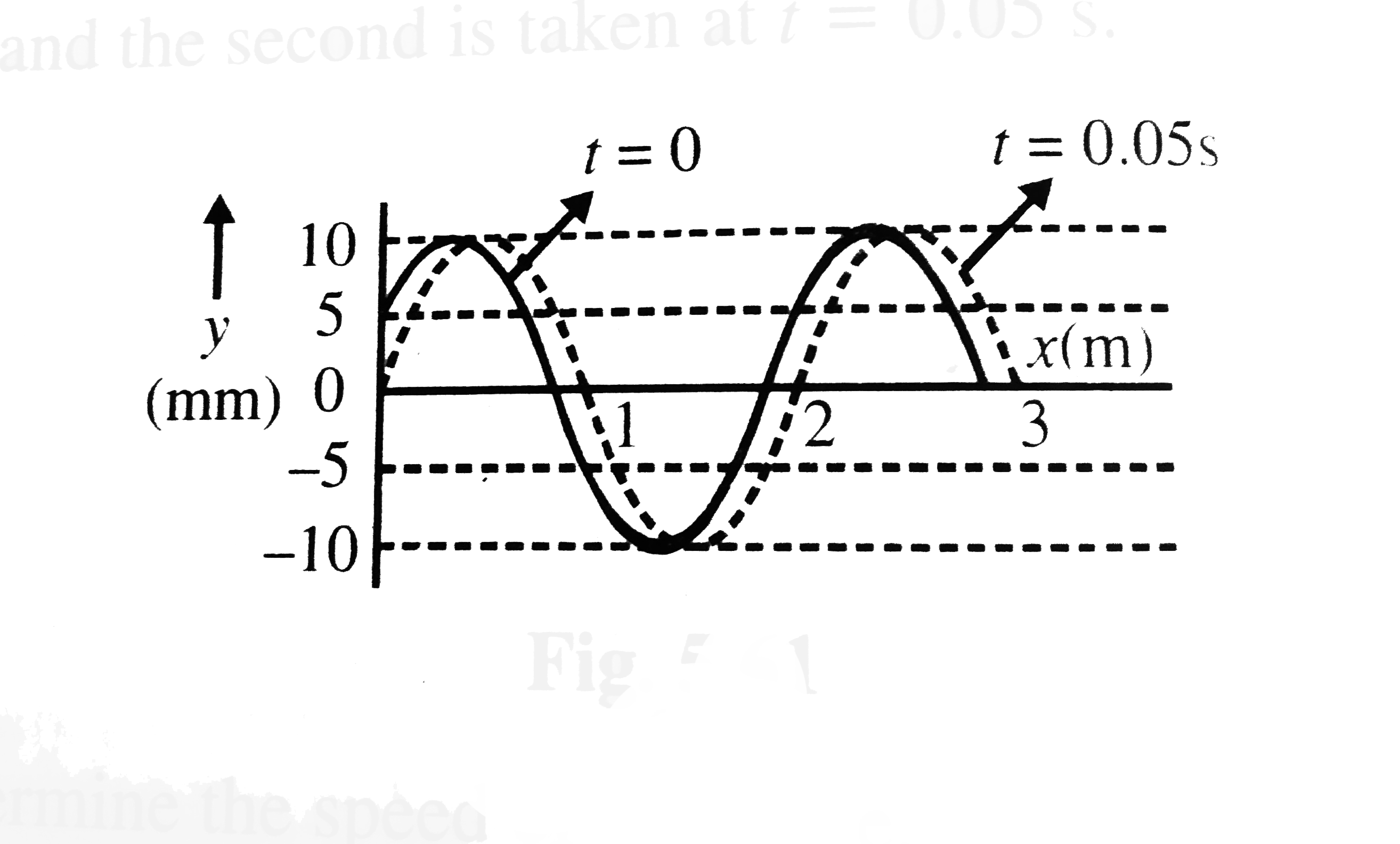
Determine the tension in the string.
View SolutionThe figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
Determine the maximum speed of the particle.
View SolutionFigure shows a snapshot for a travelling wave. The equation of wave at t=0 will be
_PHY_E06_334_Q01.png)
View SolutionFigure shows a snapshot of a sinusoidal travelling wave taken at t=0.3s.The wavelength is 7.5cm and the amplitude is 2cm. If the crest P was at x=0at t=0, write the equation of travelling wave. ,

View SolutionThe equation of a travelling wave on a string is y = (0.10 mm )sin[31.4m−1x+(314s−1)t]
View SolutionConsider a harmonic wave travelling on a string of mass per unit length mu. The wave has a velocity v, amplitude A and frequency if. The power transmitted by a harmonic wave on the string is proportional to (take constant of proportionality as 2π2)
View SolutionA transverse wave of wavelength 50 cm is travelling towards+vex-axis along a string whose linear density is 0.05g/cm. The tension in the string is 450 N. At t=0,the particle at x=0 is passing through its mean position wave. The amplitude of the wave is 2.5 cm.
View Solution
