A point source is emitting sound in all directions. The ratio of distance of two points from the point source where the difference in loudness levels is 3 dB is: .
The correct Answer is:B
To solve the problem, we need to determine the ratio of distances r1 and r2 from a point source of sound where the difference in loudness levels is 3 dB.
1. Understanding Loudness Level: The loudness level β in decibels (dB) is given by the formula:
β=10log10(II0)
where I is the intensity of the sound and I0 is the reference intensity.
2. Intensity Relation: For a point source, the intensity I at a distance r from the source is inversely proportional to the square of the distance:
I=Kr2
where K is a constant.
3. Substituting Intensity in Loudness Formula: We can substitute the expression for intensity into the loudness level equation:
\beta = 10 \log_{10} \left( \frac{K/r^2}{I_0} \right) = 10 \log_{10} \left( \frac{K}{I_0}} \right) - 20 \log_{10}(r)
Let β1 be the loudness level at distance r1 and β2 at distance r2:
β1=10log10(KI0)−20log10(r1)
β2=10log10(KI0)−20log10(r2)
4. Finding the Difference in Loudness Levels: The difference in loudness levels is given by:
β2−β1=−20log10(r2)+20log10(r1)=20log10(r1r2)
We know that this difference is 3 dB:
20log10(r1r2)=3
5. Solving for the Ratio: Dividing both sides by 20 gives:
log10(r1r2)=320=0.15
Now, we can exponentiate both sides to find the ratio:
r1r2=100.15
6. Calculating the Value: We can express 100.15 using the approximation log102≈0.3:
100.15=10320=10log10√2=√2
Therefore, we can express the ratio as:
r1r2=1√2
Final Answer:
The ratio of distances r1 and r2 where the difference in loudness levels is 3 dB is:
r1r2=1√2
β=10log10(II0)
where I is the intensity of the sound and I0 is the reference intensity.
I=Kr2
where K is a constant.
\beta = 10 \log_{10} \left( \frac{K/r^2}{I_0} \right) = 10 \log_{10} \left( \frac{K}{I_0}} \right) - 20 \log_{10}(r)
Let β1 be the loudness level at distance r1 and β2 at distance r2:
β1=10log10(KI0)−20log10(r1)
β2=10log10(KI0)−20log10(r2)
β2−β1=−20log10(r2)+20log10(r1)=20log10(r1r2)
We know that this difference is 3 dB:
20log10(r1r2)=3
log10(r1r2)=320=0.15
Now, we can exponentiate both sides to find the ratio:
r1r2=100.15
100.15=10320=10log10√2=√2
Therefore, we can express the ratio as:
r1r2=1√2
The ratio of distances r1 and r2 where the difference in loudness levels is 3 dB is:
r1r2=1√2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple26videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Assertion - Reasoning6videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Subjective24videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 241videos
THERMODYNAMICS
Similar Questions
A person standing at distance r from a point source listen the sound emitted by the source. When person move to another point having distance r10 from the point source, then increase in sound intensity level is n×101 decibles value of n is ?
View SolutionAn isotropic point source 'S' of sound emits constant power. Two points A and B are situated at distance x and 2x from S. the difference between the loudness of points A and B is about (log 2=0.3)
View SolutionA point source emitting uniformly in all directions is placed above a table top at a distance of 0.50 m from it. The luminous flux of the source is 1570 lumen. Find the illuminance at a small surface area of the table to a.directly below the source and b. at as distance of 0.80 m from the source.
View SolutionThe sound intensity is 0.008W/m2 at a distance of 10 m from an isotropic point source of sound. The power of the source is
View SolutionIf S1andS2 are two point sources emitting light in phase , then what is the shape of interference fringes formed on the screen ?
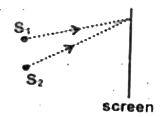 View Solution
View SolutionA point source emits sound equally in all directions in a non-absorbing medium. Two point P and Q are at distance of 2m and 3m respectively from the source. The ratio of the intensities of the wave at P and Q is :
View SolutionThe sound level at a distance of 3.00 m from a source is 120 dB. At what distance will the sound level be (a) 100 dB and (b) 10.0 dB?
View SolutionThe sound level at a distance of 3.00 m from a source is 120 dB. At what distance will the sound level be (a) 100 dB and (b) 10.0 dB?
View SolutionA point source emits sound equally in all directions in a non-absorbing medium. Two points P and Q are at the distance of 9meters and 25 meters respectively from the source. The ratio of amplitudes of the waves at P and Q is…..
View SolutionThe intensity of a point source emitting sound at distance 3 from the source is 0.707 W/m2? What is the average power output of the source ?
View Solution

