In the following equations, the distance x is in metres, the time t in seconds and the velocity v in metressecond. What are the SI units of the constants C1 and C2 ?
(a). v2=2C1x
(b). x=C1cosC2t
(c ). v=C1e−C2t
To find the SI units of the constants C1 and C2 in the given equations, we will analyze each equation step by step.
Step 1: Analyzing the first equation v2=2C1x
1. Identify the units on the left-hand side (LHS):
- The unit of velocity v is m/s.
- Therefore, the unit of v2 is (m/s)2=m2/s2.
2. Identify the units on the right-hand side (RHS):
- The unit of distance x is m.
- Thus, the unit of 2C1x is C1×m.
3. Set the units equal:
m2/s2=C1×m
4. Solve for C1:
C1=m2/s2m=m/s2
Step 2: Analyzing the second equation x=C1cos(C2t)
1. Identify the units on the left-hand side (LHS):
- The unit of distance x is m.
2. Identify the units on the right-hand side (RHS):
- The unit of C1 is m (as we found in the previous step).
- The cosine function is dimensionless, so cos(C2t) must also be dimensionless.
3. Determine the unit of C2:
- For C2t to be dimensionless, C2 must have units of s−1 (seconds inverse).
4. Set the units equal:
m=C1⋅(dimensionless)
- Thus, C1 remains m.
Step 3: Analyzing the third equation v=C1e−C2t
1. Identify the units on the left-hand side (LHS):
- The unit of velocity v is m/s.
2. Identify the units on the right-hand side (RHS):
- The unit of C1 is m/s.
- The exponential function e−C2t must be dimensionless.
3. Determine the unit of C2:
- As established earlier, C2 must have units of s−1 to ensure C2t is dimensionless.
4. Set the units equal:
m/s=C1⋅(dimensionless)
- Thus, C1 remains m/s.
Summary of Units
- The SI unit of C1 is m/s.
- The SI unit of C2 is s−1.
- The unit of velocity v is m/s.
- Therefore, the unit of v2 is (m/s)2=m2/s2.
- The unit of distance x is m.
- Thus, the unit of 2C1x is C1×m.
m2/s2=C1×m
C1=m2/s2m=m/s2
- The unit of distance x is m.
- The unit of C1 is m (as we found in the previous step).
- The cosine function is dimensionless, so cos(C2t) must also be dimensionless.
- For C2t to be dimensionless, C2 must have units of s−1 (seconds inverse).
m=C1⋅(dimensionless)
- Thus, C1 remains m.
- The unit of velocity v is m/s.
- The unit of C1 is m/s.
- The exponential function e−C2t must be dimensionless.
- As established earlier, C2 must have units of s−1 to ensure C2t is dimensionless.
m/s=C1⋅(dimensionless)
- Thus, C1 remains m/s.
- The SI unit of C2 is s−1.
Similar Questions
Knowledge Check
- Question 1 - Select One
The distance x covered in time t by a body having initial velocity v0 and having a constant acceleration a is given by
x=v0t+(1/2)at2
This result follows fromANewton's first lawBNewton's second lawCNewton's third lawDnone of the above
The velocity of a body is given by v=At2+Bt+C. If v and t are expressed in SI, what are the units of A,B and C?
View SolutionThe velocity v of the a particle depends upen the time t according to the equation v=a+bt+cd+1 Write the dimension of a, b,c and d.
View SolutionVelocity v is given by v=at2+bt+c, where t is time. What are the dimensions of a,bandc respectively?
View SolutionProve the following identity: (secA secB+tanAtanB)2−(secA tanB+tanAsecB)2=1
View SolutionFind the equations of the normal to the curve xy=c2 at (ct, c/t) on it.
View SolutionIn the following circuit, the switch S is closed at t = 0. The charge on the capacitor C1 as a function of time will be given by (Ceq=C1C2C1+C2)
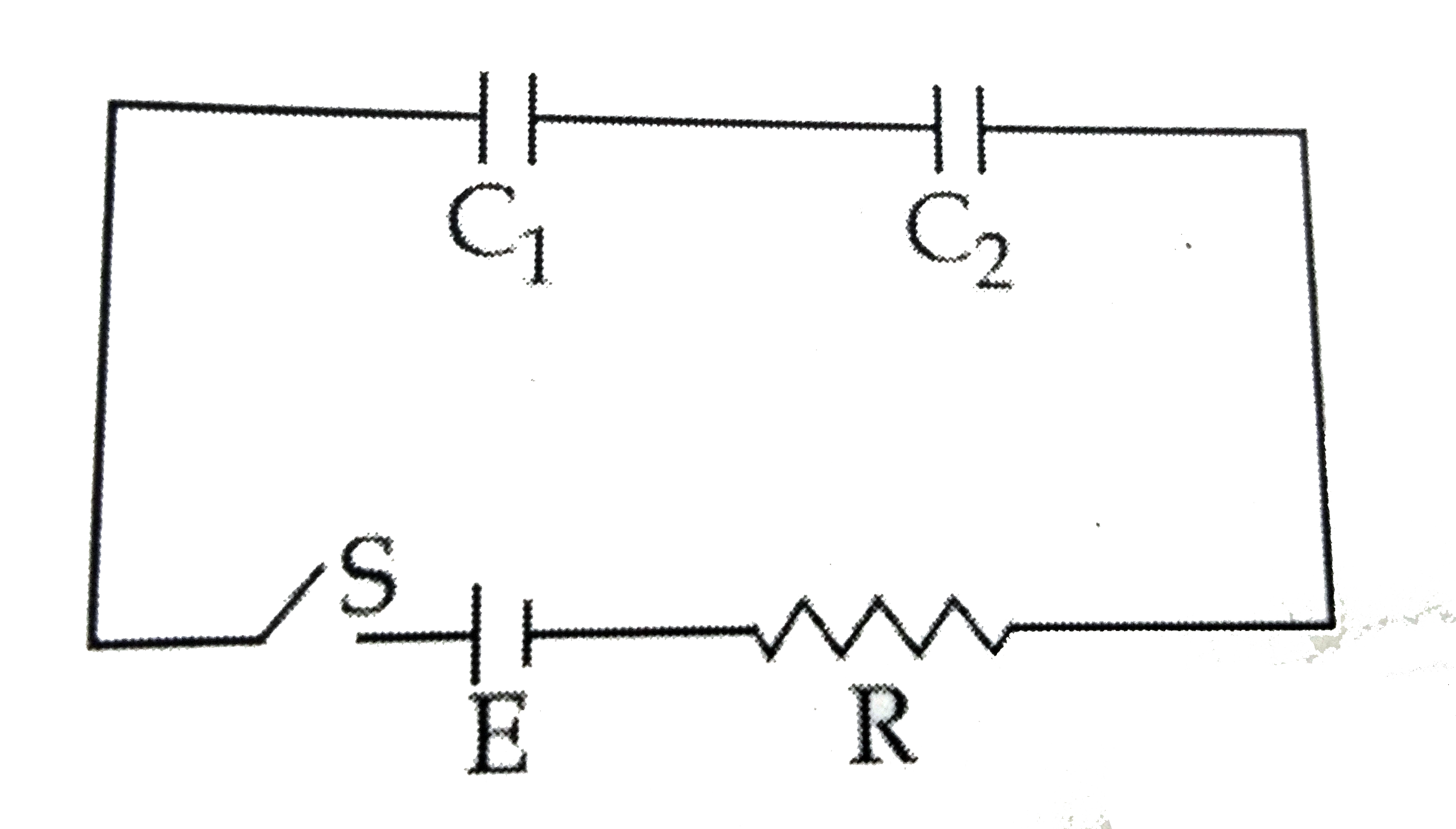 View Solution
View SolutionThe electric field in a region is given by →E=(Ax+B)ˆi Where E is in NC− and x is in metres. The values of constants are A=20SI Unit and B==10SI unit. If the potential at x=1 is V1 and that at x=−5 is V2 then V1−V2 is:
View Solution

