A boat moves with speed of 5 km/h relative to water in a river flowing with a speed of 3 km/h and having a width of 1 km. The minimum time taken around a round trip is
5 min
60 min
20 min
30 min
The correct Answer is:D
To solve the problem of the boat's round trip across a river, we can break down the solution into clear steps:
Step 1: Understand the Problem
We have a boat moving with a speed of 5 km/h relative to the water. The river flows with a speed of 3 km/h, and the width of the river is 1 km. We need to find the minimum time taken for a round trip across the river.
Step 2: Determine the Effective Speed of the Boat
The boat's speed relative to the ground when moving across the river can be calculated using the Pythagorean theorem. The effective speed of the boat v when moving perpendicular to the current can be found as follows:
veffective=√(vboat)2−(vriver)2
Where:
- vboat=5 km/h (speed of the boat relative to water)
- vriver=3 km/h (speed of the river)
Step 3: Calculate the Effective Speed
Substituting the values into the equation:
veffective=√(5)2−(3)2=√25−9=√16=4 km/h
Step 4: Calculate the Time to Cross the River
The time taken to cross the river (one way) can be calculated using the formula:
Time=DistanceSpeed
The distance to cross the river is 1 km, and the effective speed is 4 km/h:
Time to cross one way=1 km4 km/h=0.25 hours
Step 5: Calculate the Total Time for the Round Trip
Since the round trip consists of going to the other bank and returning, the total time is:
Total Time=2×Time to cross one way=2×0.25 hours=0.5 hours
Step 6: Convert Time to Minutes
To convert the time from hours to minutes:
0.5 hours=0.5×60 minutes=30 minutes
Final Answer
The minimum time taken for the round trip is 30 minutes.
---
We have a boat moving with a speed of 5 km/h relative to the water. The river flows with a speed of 3 km/h, and the width of the river is 1 km. We need to find the minimum time taken for a round trip across the river.
The boat's speed relative to the ground when moving across the river can be calculated using the Pythagorean theorem. The effective speed of the boat v when moving perpendicular to the current can be found as follows:
- vboat=5 km/h (speed of the boat relative to water)
- vriver=3 km/h (speed of the river)
Substituting the values into the equation:
The time taken to cross the river (one way) can be calculated using the formula:
Since the round trip consists of going to the other bank and returning, the total time is:
To convert the time from hours to minutes:
The minimum time taken for the round trip is 30 minutes.
Similar Questions
A man can swim in still water at a speed of 4 kmph. He desires to cross a river flowing at a speed of 3 kmph in the shortest time interval. If the width of the river is 3km time taken to cross the river (in hours) and the horizontal distance travelled (in km) are respectively
View SolutionA boat man can row with a speed of 10 km/hr. in still water. The river flow steadily at 5 km/hr. and the width of the river is 2 km. if the boat man cross the river with reference to minimum distance of approach then time elapsed in rowing the boat will be:-
View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite point on the other bank. If the river is flowing at a speed of 3 kmph, and the width of the river is 2 km, the time taken to cross the river is (in hours)
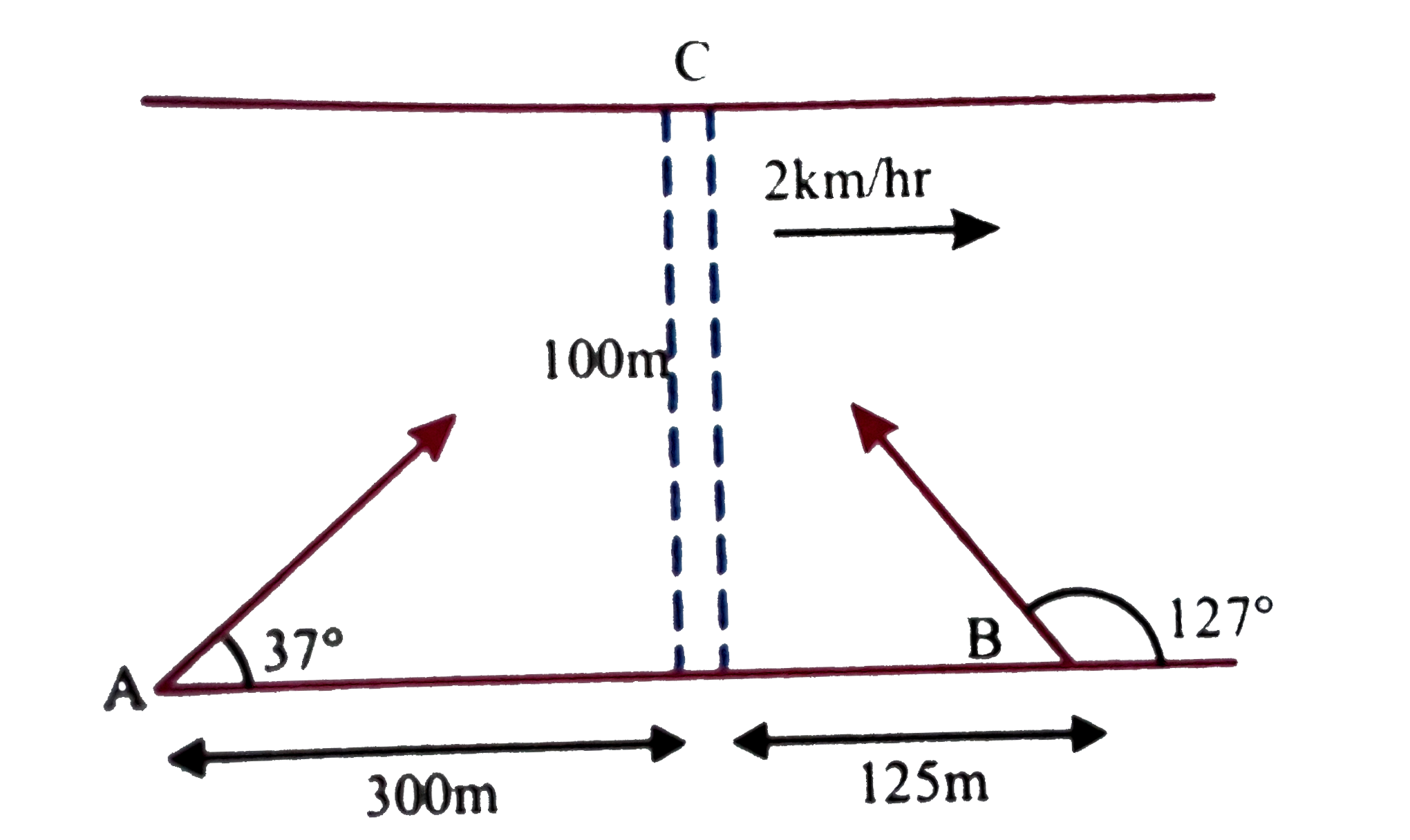
View SolutionTwo swimmers start a race. One who reaches the point C first on the other bank wins the race.A makes his strokes in a direction of 370 to the river flow with velocity 5km/hr relative to water. B makes his strokes in a direction 1270 to the river flow with same relative velocity.River is flowing with speed of 2km/hr and is 100m wide.speeds of A and B on the ground are 8km/hr and 6km/hr respectively.
 View Solution
View SolutionA boat man can row with a speed of 10kmh−1 in still water. If the river flows steadily at 5km/h, in which direction should the boatman row in order to reach a point on the other bank directly opposite to the point from where he stared ? The width of the river is 2km.
View SolutionA man can swin with speed 6 km/h in still water. If he tries of swim across a river flowing with speed 5km/h, how fast does a leaf floating on water appears to go , to the man ?
View SolutionA boat, which can travel at a speed of 8 km/h in still water on a lake, is rowing in the flowing water in river. If the stream speed is 3 km/h, how fast the boat can cross a tree on the shore in traveling
upstream ?View SolutionA car is running at a speed of 15 km h−1 while another Similar car is moving at a speed of 45 km h−1 . Find the ratio of their kinetic energies.
View SolutionA boat which has a speed of 5km/hr in steel water crosses a river of width 1km along the shortest possible path in 15minutes. The velocity of the river water in km/hr is
View SolutionA boat which has a speed of 5km/hr in steel water crosses a river of width 1km along the shortest possible path in 15minutes. The velocity of the river water in km/hr is
View Solution

