When volume of system is increased two times and temperature is decreased half of its initial temperature, then pressure becomes ………….
The correct Answer is:A, D
To solve the problem step by step, we will use the ideal gas law, which is given by the equation:
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles of gas
- R = Universal gas constant
- T = Temperature in Kelvin
1. Define Initial Conditions:
- Let the initial volume be Vi.
- Let the initial pressure be Pi.
- Let the initial temperature be Ti.
2. Define Final Conditions:
- The volume is increased to two times its initial volume:
Vf=2Vi
- The temperature is decreased to half of its initial temperature:
Tf=Ti2
3. Apply Ideal Gas Law for Initial and Final States:
- For the initial state:
PiVi=nRTi(1)
- For the final state:
PfVf=nRTf(2)
4. Substitute Final Conditions into the Ideal Gas Law:
- Substitute Vf and Tf into equation (2):
Pf(2Vi)=nR(Ti2)
5. Simplify the Final State Equation:
- Rearranging gives:
Pf⋅2Vi=nRTi2
- Dividing both sides by 2:
PfVi=nRTi4(3)
6. Substitute Equation (1) into Equation (3):
- From equation (1), we know that nRTi=PiVi.
- Substitute this into equation (3):
PfVi=PiVi4
7. Solve for Final Pressure Pf:
- Divide both sides by Vi (assuming Vi≠0):
Pf=Pi4
Conclusion:
The final pressure Pf becomes one-fourth of the initial pressure Pi:
Pf=Pi4
- P = Pressure
- V = Volume
- n = Number of moles of gas
- R = Universal gas constant
- T = Temperature in Kelvin
- Let the initial volume be Vi.
- Let the initial pressure be Pi.
- Let the initial temperature be Ti.
- The volume is increased to two times its initial volume:
Vf=2Vi
- The temperature is decreased to half of its initial temperature:
Tf=Ti2
- For the initial state:
PiVi=nRTi(1)
- For the final state:
PfVf=nRTf(2)
- Substitute Vf and Tf into equation (2):
Pf(2Vi)=nR(Ti2)
- Rearranging gives:
Pf⋅2Vi=nRTi2
- Dividing both sides by 2:
PfVi=nRTi4(3)
- From equation (1), we know that nRTi=PiVi.
- Substitute this into equation (3):
PfVi=PiVi4
- Divide both sides by Vi (assuming Vi≠0):
Pf=Pi4
The final pressure Pf becomes one-fourth of the initial pressure Pi:
Pf=Pi4
Topper's Solved these Questions
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Multiple correct
3 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Comprehension Type
5 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
Similar Questions
Knowledge Check
When temperature decrease, oxy-Hb curve becomes
Amore steepBstraightCparabolaDnone of theseWhen temperature decreases, oxy-Hb curve will become
Amore steepBstraightCparabolaDall of these
The pressure of a gas filled in a closed containeer increases by 1% when temperature is increased by 1∘C. Find the initial temperature of the gas.
View SolutionThe pressure of a gas filled in a closed jar increases by 0.2%, when temperature is increased by 2∘C. Find the initial temperature of the gas.
View SolutionThe pressure of a gas filled in a closed vessel increase by 0.4% when temperature is increased by 1∘C. Find the initial temperature of the gas.
View SolutionThe temperature of the system decreases in an
View SolutionA graph is plotted between pressure and volume at different temperatures . On the basis of the graph what changes will you observe in the volume if
(i) the pressure is increased at constant temperature .
(ii) the temperature is decreased at constant pressure .
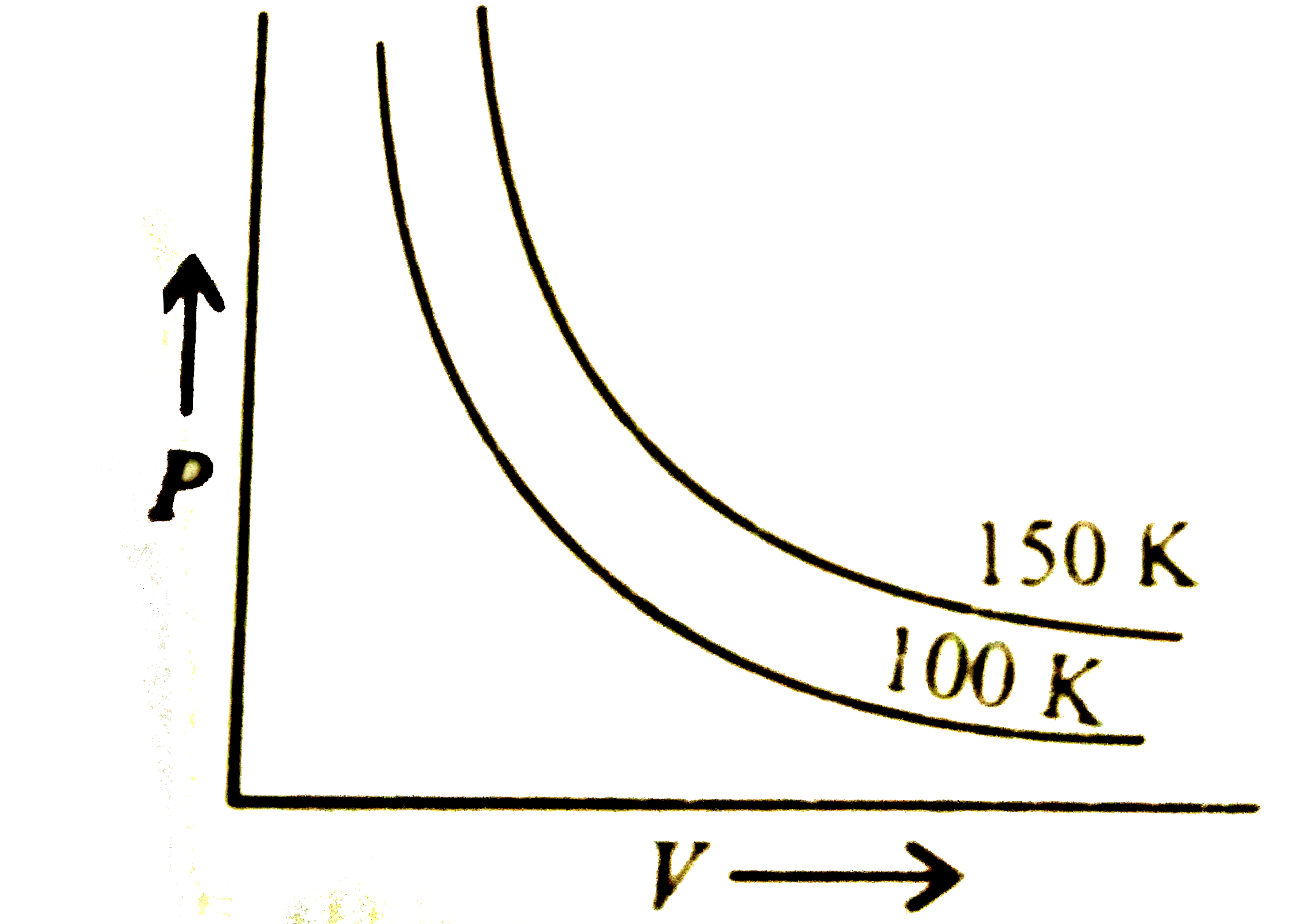
View SolutionA sample of O2 is at a pressure of 1 atm when the volume is 100ml and its temperature is 27C .what will be the temperature of the gas if the pressure becomes 2 atm and volume remains 100 ml.
View Solution
