A body at the end of a spring executes SHM with a period while the corresponding period for another spring is If the period of oscillation with the two spring in series is T, then
The correct Answer is:B
To solve the problem, we need to find the period of oscillation T when two springs with periods t1 and t2 are connected in series. We will use the relationship between the time period and the spring constant.
1. Understanding the Time Period of a Spring:
The time period t of a mass-spring system is given by the formula:
t=2π√mk
where m is the mass and k is the spring constant.
2. Relating Time Period to Spring Constant:
Squaring both sides of the equation gives:
t2=4π2mk
Rearranging this, we find:
k=4π2mt2
3. Finding the Spring Constants:
For the two springs with periods t1 and t2:
- For spring 1:
k1=4π2mt21
- For spring 2:
k2=4π2mt22
4. Calculating the Equivalent Spring Constant:
When the two springs are connected in series, the equivalent spring constant keq is given by:
1keq=1k1+1k2
Substituting the values of k1 and k2:
1keq=t214π2m+t224π2m
This simplifies to:
1keq=t21+t224π2m
Therefore, the equivalent spring constant is:
keq=4π2mt21+t22
5. Finding the Period of the Combined System:
Now, using the formula for the time period with the equivalent spring constant:
T=2π√mkeq
Substituting for keq:
T=2π
⎷m4π2mt21+t22
This simplifies to:
T=2π√t21+t224π2=√t21+t224=12√t21+t22
Final Answer:
The period of oscillation T when the two springs are connected in series is:
T=12√t21+t22
The time period t of a mass-spring system is given by the formula:
t=2π√mk
where m is the mass and k is the spring constant.
Squaring both sides of the equation gives:
t2=4π2mk
Rearranging this, we find:
k=4π2mt2
For the two springs with periods t1 and t2:
- For spring 1:
k1=4π2mt21
- For spring 2:
k2=4π2mt22
When the two springs are connected in series, the equivalent spring constant keq is given by:
1keq=1k1+1k2
Substituting the values of k1 and k2:
1keq=t214π2m+t224π2m
This simplifies to:
1keq=t21+t224π2m
Therefore, the equivalent spring constant is:
keq=4π2mt21+t22
Now, using the formula for the time period with the equivalent spring constant:
T=2π√mkeq
Substituting for keq:
T=2π
⎷m4π2mt21+t22
This simplifies to:
T=2π√t21+t224π2=√t21+t224=12√t21+t22
The period of oscillation T when the two springs are connected in series is:
T=12√t21+t22
Similar Questions
A mass is suspended at the end of a spring and performs SHM with a time period 3 seconds, while the time period f same mass wih other spring is 4 second. If that mass is connected with the combination of the two springs connected in series then time period of oscillator is
View SolutionThe time period of oscillations of a block attached to a spring is t1. When the spring is replaced by another spring, the time period of the block is t2. If both the springs are connected in series and the block is made to oscillate using the combination, then the time period of the block is
View Solution
Knowledge Check
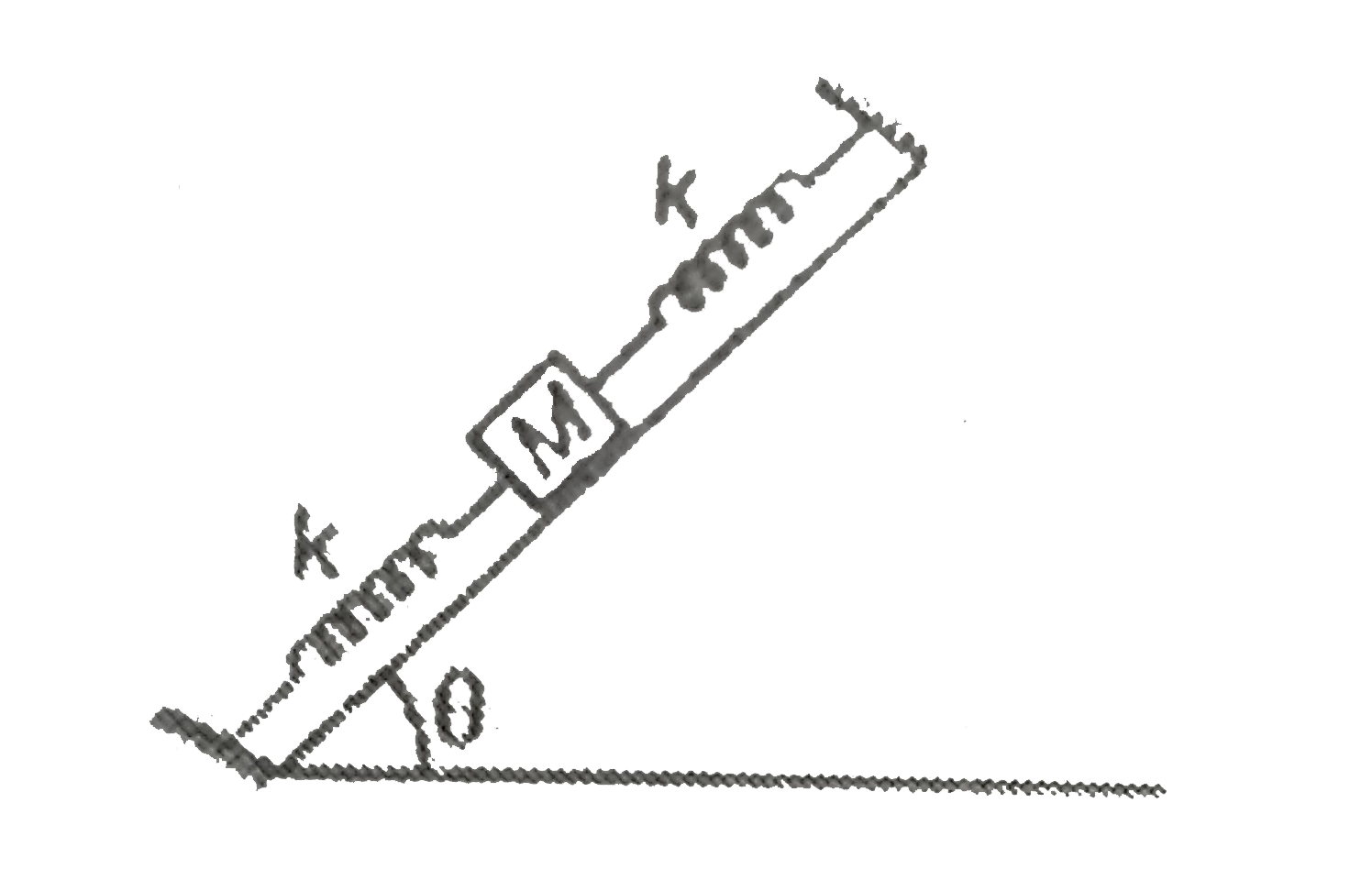
On a smooth inclined plane, a body of mass M is attached between two springs. The other ends of the springs are fixed to firm supports. If each spring has force constant K , the period of oscillation of the body (assuming the springs as massless) is
 A1. 2π√M2kB2. 2π√2MkC3. 2π√Mgsinθ2kD4. 2π√2Mgk
A1. 2π√M2kB2. 2π√2MkC3. 2π√Mgsinθ2kD4. 2π√2Mgk
In the adjacent figure, if the incline plane is smooth and the springs are identical then the period of oscillation of this body is

View SolutionWhen a boy is playing on a swing in the sitting position , the time period of oscillations of the swing is T. If the boy stands up , the time period of oscillation of the spring will be
View SolutionTime period of a given spring-mass system is

View SolutionA body is in SHM with period T when oscillated from a freely suspended spring. If this spring is cut in two parts of length ratio 1:3& again oscillated from the two the two parts separatedly, then the periods are T1&T2 then find T1/T2.
View SolutionOn a smooth inclined plane a body of mass M is attached between two springs. The other ends of the springs are fixed to firm supports. If each spring has a force constant k, the period of oscilation of the body is (assuming the spring as massless)
View SolutionA body of mass m hung at one end of the spring executes simple harmonic motion . The force constant of a spring is k while its period of vibration is T. Prove by dimensional method that the equation T=2π√m/k is correct. Dervive the correct equation , assuming that they are related by a power law.
View SolutionIf the period of oscillation of mass M suspended from a spring is one second, then the period of 9M will be
View Solution
