A proton of charge +e and mass m enters a uniform magnetic Field →B=ˆB with an intial velocity →v=v0xˆi+v0yˆj. Find an expression in unit-vector notation for its velocity at any later time t.
The correct Answer is:→v(t)=v0xˆi+v0y(sinωt)ˆk
To find the expression for the velocity of a proton moving in a uniform magnetic field at any later time t, we start with the given information:
1. Charge of the proton: +e
2. Mass of the proton: m
3. Initial velocity: →v=v0x^i+v0y^j
4. Magnetic field: →B=B^i
Step 1: Analyze the motion in the magnetic field
The magnetic force acting on a charged particle moving in a magnetic field is given by the Lorentz force equation:
→F=q→v×→B
In our case, the magnetic field →B is in the ^i direction, and the velocity →v has components in both the ^i and ^j directions.
Step 2: Calculate the magnetic force
Substituting the values into the Lorentz force equation:
→F=e(v0x^i+v0y^j)×(B^i)
Using the cross product properties:
^i×^i=0and^j×^i=−^k
Thus, we have:
→F=ev0y^j×B^i=eBv0y(−^k)=−eBv0y^k
Step 3: Determine the acceleration
Using Newton's second law, →F=m→a, we can find the acceleration:
m→a=−eBv0y^k
This gives:
→a=−eBv0ym^k
Step 4: Integrate to find velocity
The acceleration is constant in the ^k direction, so we can integrate to find the velocity in the ^k direction:
vz(t)=v0z+azt=0−eBv0ymt=−eBv0ymt
Step 5: Combine the components of velocity
The x and y components of the velocity remain unchanged because the magnetic field does not exert a force in those directions. Therefore, the velocity at time t can be expressed as:
→v(t)=v0x^i+v0y^j+vz(t)^k
Substituting vz(t):
→v(t)=v0x^i+v0y^j−eBv0ymt^k
Final Expression
Thus, the expression for the velocity of the proton at any later time t is:
→v(t)=v0x^i+v0y^j−eBv0ymt^k
2. Mass of the proton: m
3. Initial velocity: →v=v0x^i+v0y^j
4. Magnetic field: →B=B^i
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple
2 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Comphension Based
2 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Comprehension type
8 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A proton of charge e and mass m enters a uniform magnetic field B=Bi with an initial velocity v=vxˆi+vyˆj. Find an expression in unit vector notation for its velocity at time t.
View Solution
A particle of charge 'q' and mass 'm' enters a uniform magnetic field region →B=−Bˆk at origin 'O' with a velocity →v0=6ˆi m/s and after some time it exist the magentic field region with a velocity →v=(3ˆi+3√3)m/s as shown. The time interval for which the particle has moved in the magnetic field region is
View Solution
Knowledge Check
An electron is moving with an initial velocity →v=v0ˆi and is in a magnetic field →B=B0ˆj. Then it's de-Broglie wavelength
Aremains constantBincreases with timeCdecreases with timeDincreases and decreases periodically
A particle of charge -q and mass m enters a uniform magnetic field →B (perpendicular to paper inward) at P with a velocity v0 at an angle α and leaves the field at Q with velocity v at angle β as shown in fig.
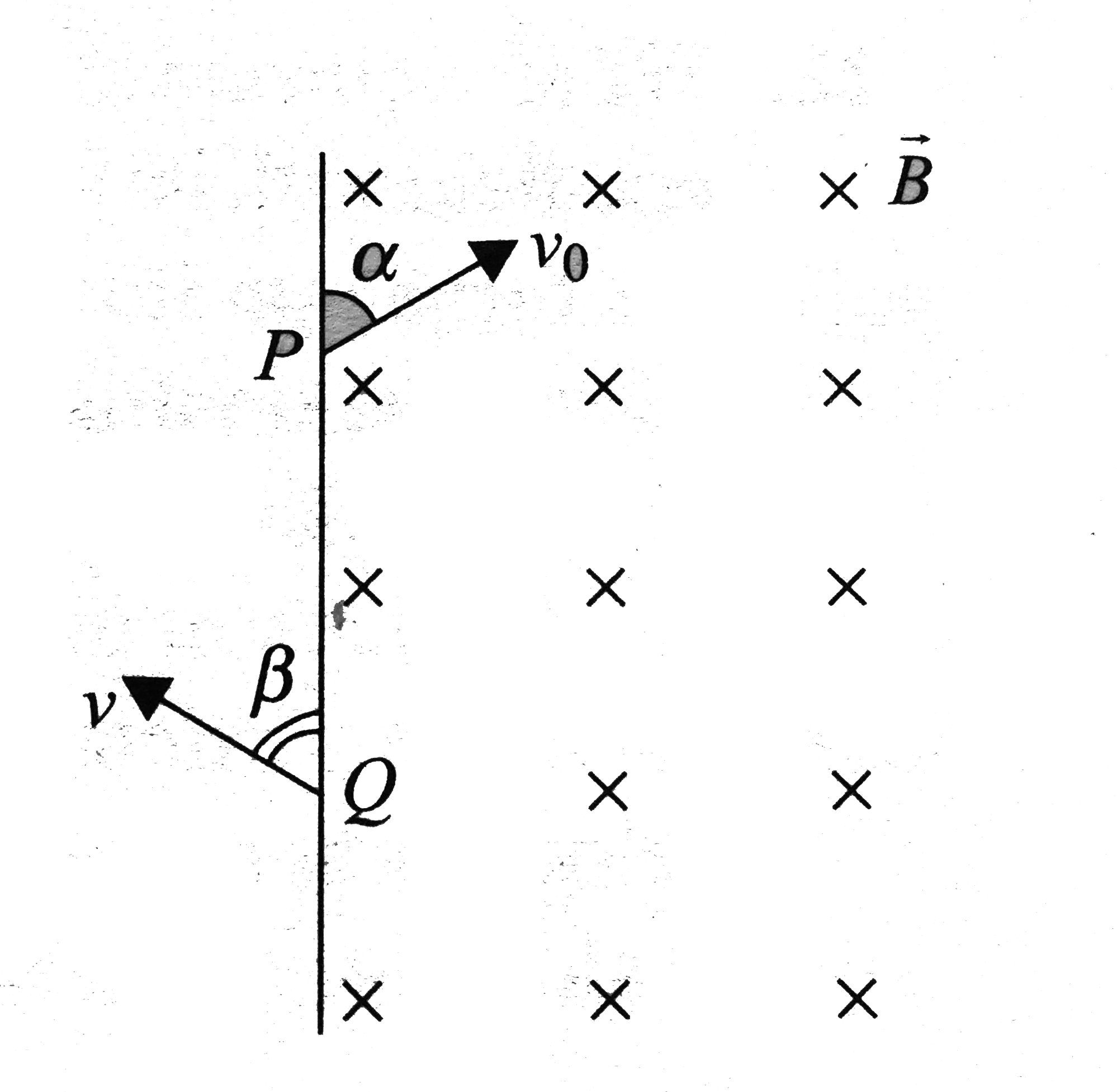
View SolutionA particle of spectfic charge α enters a uniform magnetic field B=−B0ˆk with velocity V=v0ˆi from the origin Find the time dependence of velocity and position of the particle
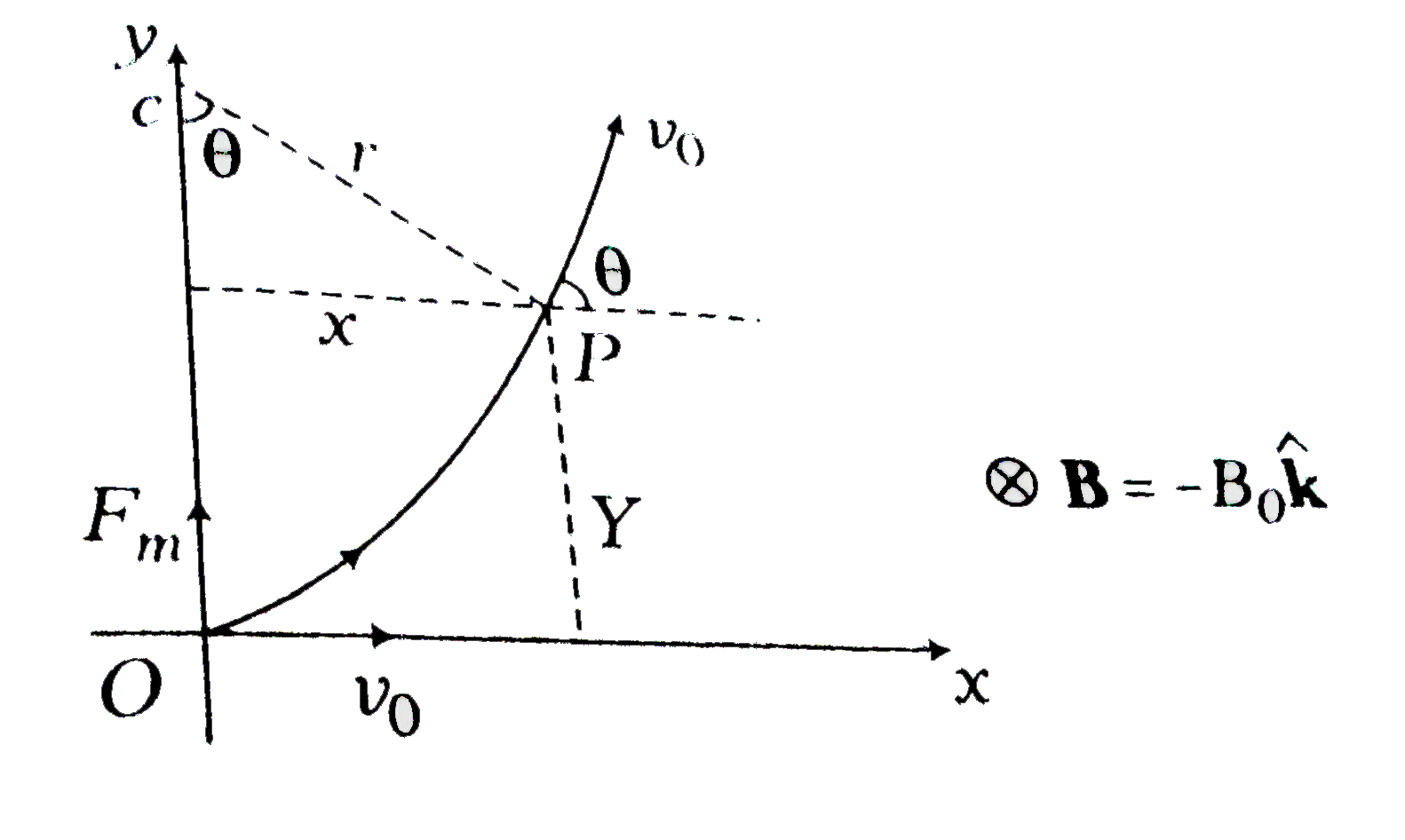
View SolutionA particle of specific charge alpha enters a uniform magnetic field B=−B0ˆk with velocity v=v0ˆi from the origin. Find the time dependence of velocity and position of the particle.

View SolutionA charge q of mass m enters a region of electric field E=−E0ˆj with a velocity v0ˆi+v0ˆj at origin. Find the x coordinate where the charge hits the x axis again. (Neglect effect of gravity)
View SolutionA positive charge particle of charge q and mass m enters into a uniform magnetic field with velocity v as shown in Fig. There is no magnetic field to the left of PQ. Find (a) time spent, (b) distance travelled in the magnetic field, (c) impulse of magnetic force.
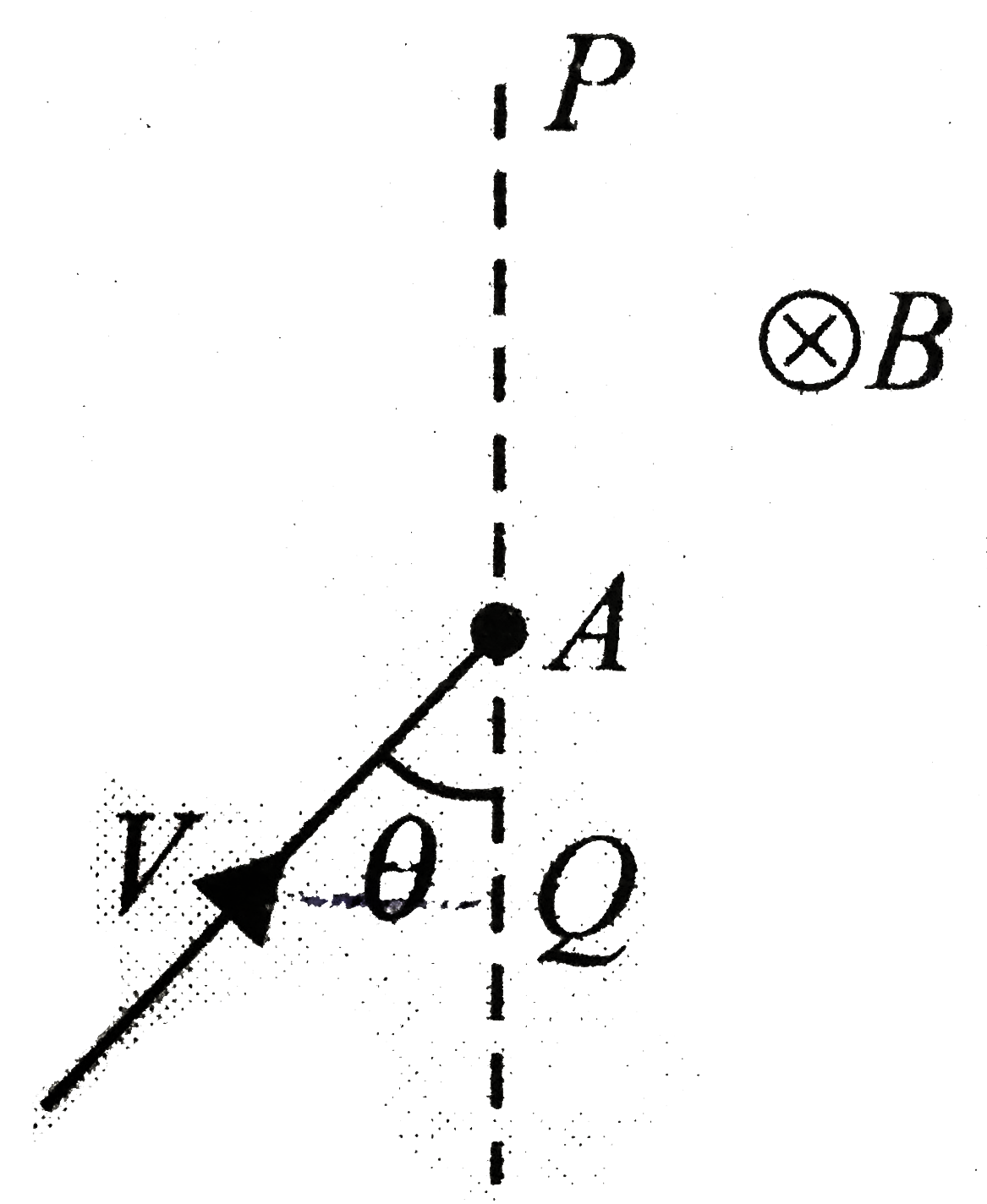
View SolutionA positive charge particle of charge q and mass m enters into a uniform magnetic field with velocity v as shown in Fig. There is no magnetic field to the left of PQ. Find (a) time spent, (b) distance travelled in the magnetic field, (c) impulse of magnetic force.
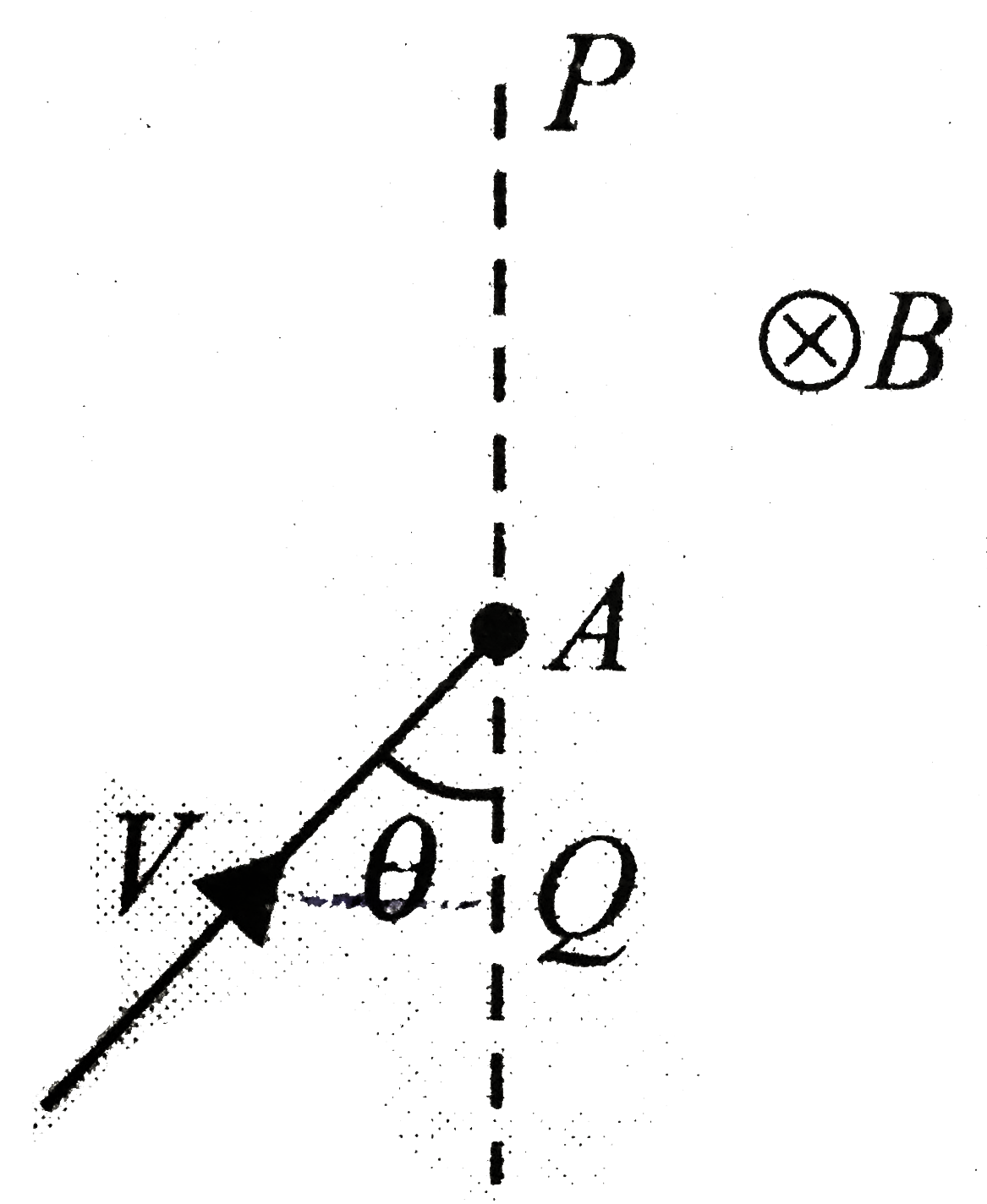
View SolutionAn electron is moving with an initial velocity →v=v0ˆi and is in a magnetic field →B=B0ˆj. Then it's de-Broglie wavelength
View Solution