Which of the following processes would lead to an increase in the average speed of the molecules of an ideal gas system?
Decreasing the temperature of the system
Compressing the gas with a piston
Expanding the gas into a vacuum
Heating the system keeping V and P constant.
To determine which processes would lead to an increase in the average speed of the molecules of an ideal gas system, we will analyze each option based on the relationship between average speed, temperature, and volume.
1. Understanding Average Speed of Gas Molecules:
The average speed (v) of gas molecules in an ideal gas is given by the equation:
v=√8RTπm
where:
- R is the universal gas constant,
- T is the absolute temperature in Kelvin,
- m is the mass of a gas molecule.
2. Identifying Factors Affecting Average Speed:
From the equation, we can see that:
- The average speed is directly proportional to the square root of the temperature (T).
- The average speed is inversely proportional to the square root of the mass of the gas molecules (m).
3. Analyzing Each Process:
- Process 1: Decreasing the Temperature of the System:
- If the temperature decreases, the average speed will also decrease.
- Conclusion: This process does not lead to an increase in average speed.
- Process 2: Compressing the Gas with a Piston:
- Compressing the gas reduces its volume. According to the ideal gas law (PV=nRT), if the volume decreases while the number of moles and temperature remain constant, the pressure increases.
- An increase in pressure at constant temperature can lead to an increase in average speed due to the relationship between pressure and speed.
- Conclusion: This process leads to an increase in average speed.
- Process 3: Expanding the Gas into Vacuum:
- Expanding the gas into a vacuum means the volume increases without any external pressure acting on it. This does not change the temperature or the number of moles of gas.
- An increase in volume without a corresponding increase in temperature will not increase the average speed.
- Conclusion: This process does not lead to an increase in average speed.
- Process 4: Heating the System while Keeping Volume and Pressure Constant:
- If the system is heated, the temperature increases. Since average speed is directly proportional to the square root of temperature, this will increase the average speed of the gas molecules.
- Conclusion: This process leads to an increase in average speed.
4. Final Answer:
The processes that lead to an increase in the average speed of the molecules of an ideal gas are:
- Compressing the gas with a piston (Process 2).
- Heating the system while keeping volume and pressure constant (Process 4).
Summary:
The correct options that lead to an increase in average speed are:
- Option B: Compressing the gas with a piston.
- Option D: Heating the system while keeping volume and pressure constant.
The average speed (v) of gas molecules in an ideal gas is given by the equation:
v=√8RTπm
where:
- R is the universal gas constant,
- T is the absolute temperature in Kelvin,
- m is the mass of a gas molecule.
From the equation, we can see that:
- The average speed is directly proportional to the square root of the temperature (T).
- The average speed is inversely proportional to the square root of the mass of the gas molecules (m).
- Process 1: Decreasing the Temperature of the System:
- If the temperature decreases, the average speed will also decrease.
- Conclusion: This process does not lead to an increase in average speed.
- Compressing the gas reduces its volume. According to the ideal gas law (PV=nRT), if the volume decreases while the number of moles and temperature remain constant, the pressure increases.
- An increase in pressure at constant temperature can lead to an increase in average speed due to the relationship between pressure and speed.
- Conclusion: This process leads to an increase in average speed.
- Expanding the gas into a vacuum means the volume increases without any external pressure acting on it. This does not change the temperature or the number of moles of gas.
- An increase in volume without a corresponding increase in temperature will not increase the average speed.
- Conclusion: This process does not lead to an increase in average speed.
- If the system is heated, the temperature increases. Since average speed is directly proportional to the square root of temperature, this will increase the average speed of the gas molecules.
- Conclusion: This process leads to an increase in average speed.
The processes that lead to an increase in the average speed of the molecules of an ideal gas are:
- Compressing the gas with a piston (Process 2).
- Heating the system while keeping volume and pressure constant (Process 4).
The correct options that lead to an increase in average speed are:
- Option B: Compressing the gas with a piston.
- Option D: Heating the system while keeping volume and pressure constant.
Topper's Solved these Questions
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Exercises (Single Correct)
85 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Exercises (Assertion-Reasoning)
24 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Exercises (Linked Comprehensive)
48 Videos
- SOME BASIC CONCEPTS AND MOLE CONCEPTBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOME BASIC CONCEPTS AND MOLE CONCEPTEXERCISE - Archives Subjective
11 Videos
- STOICHIOMETRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective
33 Videos
Similar Questions
Which expression gives average speed of gas molecules?
View SolutionThe expression of average speed of molecules of a gas is given as :
View SolutionDuring the process AB of an ideal gas
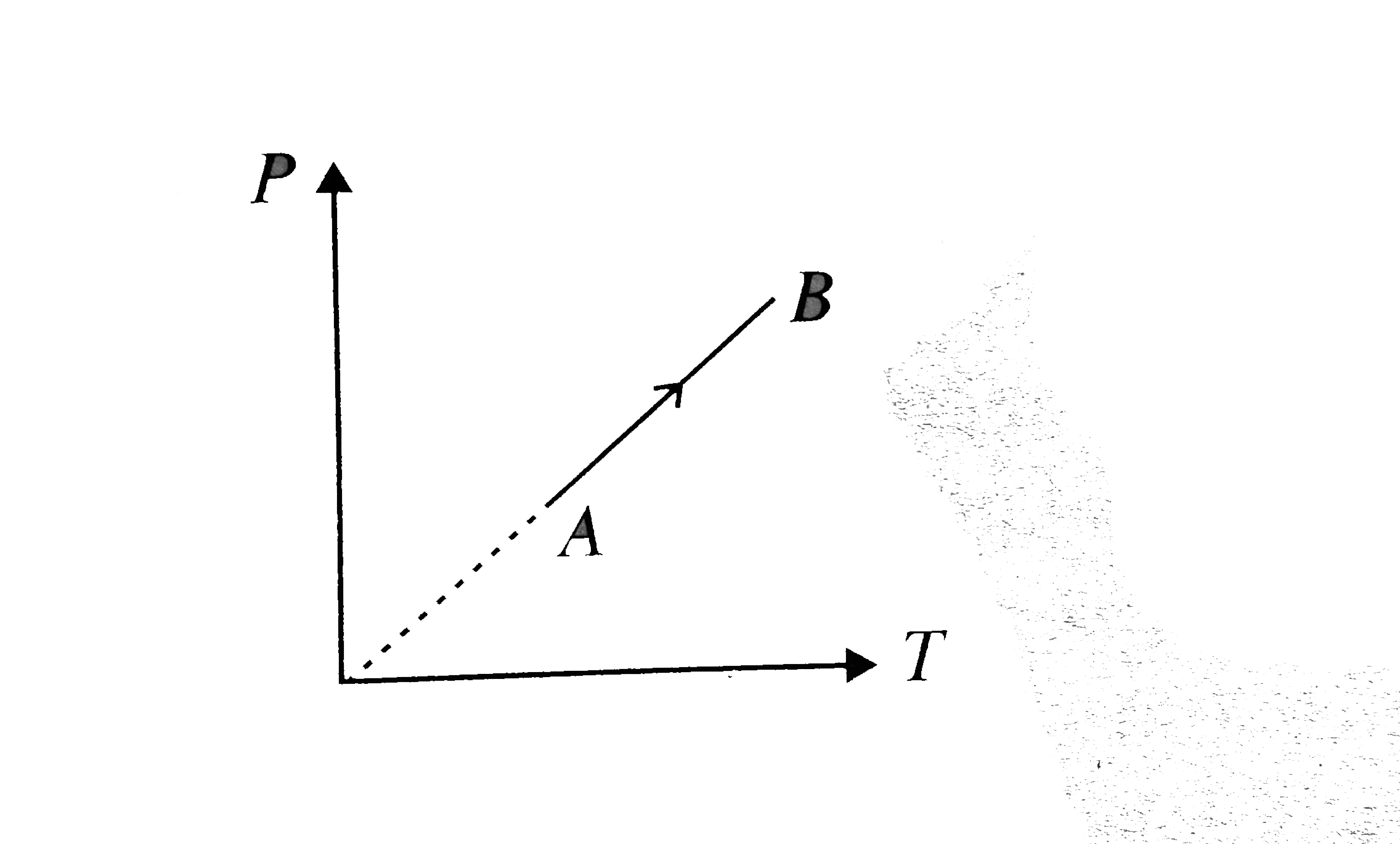
View SolutionDuring the process AB of an ideal gas

View SolutionWhich of the following quantities is zero on an average for the molecules of an ideal gas in equilibrium?
View SolutionThe average velocity of the molecules in a gas in equilibrium is
View SolutionIn an isothermal process for an ideal gas
View SolutionThe average kinetic energy of a gas molecule is
View SolutionTemperature of an ideal gas increases in:
View SolutionThe following graphs shows two isothermal process for a fixed mass of an ideal gas. Find the ratio of r.m.s speed of the molecules at temperatures T1 and T2?
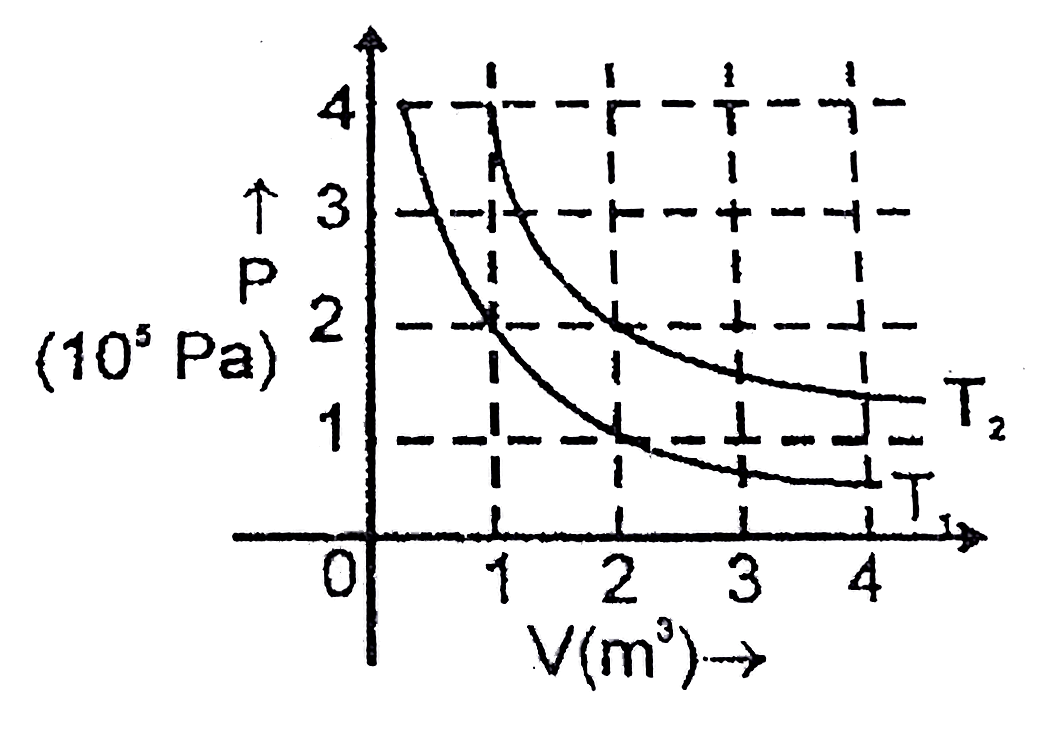
View Solution
