What is the relation between three types of molecular speeds at a given temperature?
To find the relation between the three types of molecular speeds at a given temperature, we will derive the expressions for each speed and then compare them.
Step 1: Define the Types of Molecular Speeds
1. RMS Velocity (C_RMS): This is the root mean square velocity of the gas molecules.
CRMS=√3RTM
where R is the gas constant, T is the temperature, and M is the molecular weight.
2. Average Velocity (C_{average}): This is the average speed of the gas molecules.
Caverage=√8RTπM
3. Most Probable Velocity (C_{mp}): This is the speed of the molecules that are most likely to be found at a given temperature.
Cmp=√2RTM
Step 2: Calculate the Ratios
Now, we will find the ratios of these velocities to understand their relationship.
1. Ratio of Most Probable Velocity to Average Velocity:
CmpCaverage=√2RTM√8RTπM=√2RT⋅πM8RT=√π4=12√π≈0.886
2. Ratio of Average Velocity to RMS Velocity:
CaverageCRMS=√8RTπM√3RTM=√83π≈0.654
3. Ratio of Most Probable Velocity to RMS Velocity:
CmpCRMS=√2RTM√3RTM=√23≈0.816
Step 3: Summary of Relationships
From the calculations, we can summarize the relationships between the three types of molecular speeds:
- Cmp:Caverage:CRMS≈1:1.128:1.224
Final Conclusion
Thus, the relation between the three types of molecular speeds at a given temperature is:
- Most Probable Velocity (C_{mp}) is the smallest,
- Average Velocity (C_{average}) is in the middle,
- RMS Velocity (C_{RMS}) is the largest.
1. RMS Velocity (C_RMS): This is the root mean square velocity of the gas molecules.
CRMS=√3RTM
where R is the gas constant, T is the temperature, and M is the molecular weight.
Caverage=√8RTπM
Cmp=√2RTM
Now, we will find the ratios of these velocities to understand their relationship.
CmpCaverage=√2RTM√8RTπM=√2RT⋅πM8RT=√π4=12√π≈0.886
CaverageCRMS=√8RTπM√3RTM=√83π≈0.654
CmpCRMS=√2RTM√3RTM=√23≈0.816
From the calculations, we can summarize the relationships between the three types of molecular speeds:
- Cmp:Caverage:CRMS≈1:1.128:1.224
Thus, the relation between the three types of molecular speeds at a given temperature is:
- Most Probable Velocity (C_{mp}) is the smallest,
- Average Velocity (C_{average}) is in the middle,
- RMS Velocity (C_{RMS}) is the largest.
Topper's Solved these Questions
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Exercises (Fill In The Blanks)
25 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Exercises (Ture False)
25 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Concept Application 5.3
1 Videos
- SOME BASIC CONCEPTS AND MOLE CONCEPTBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOME BASIC CONCEPTS AND MOLE CONCEPTEXERCISE - Archives Subjective
11 Videos
- STOICHIOMETRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective
33 Videos
Similar Questions
Knowledge Check
Relation between vapour density and molecular weight
AMolecular weight = 2/Vapour densityBMolecular weight = 2 × Vapour densityCMolecular weight × 2 = Vapour densityDNone of these
The relation between given pair is
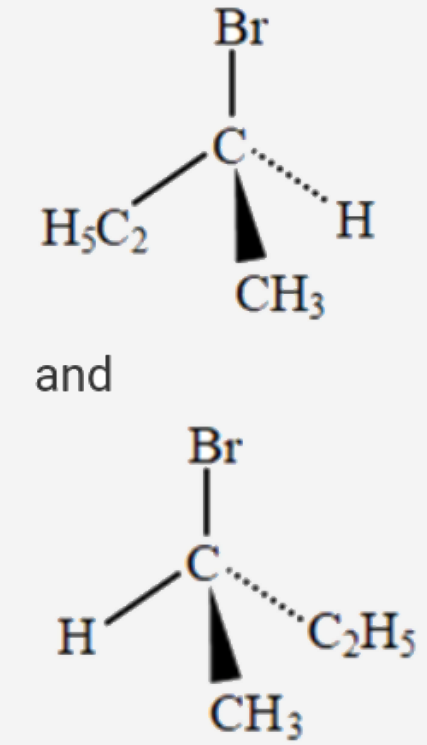
View Solution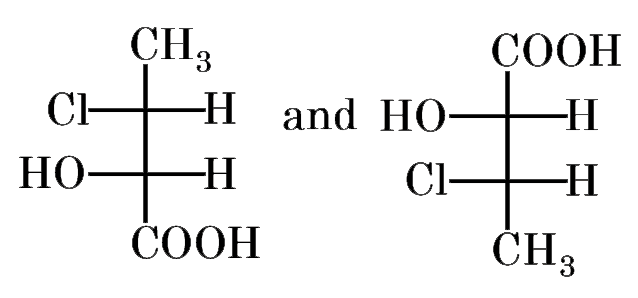
relation between given pairs is:
View SolutionWhat is the relation in between o-cresol and anisol :
View SolutionWhat is the relation between average speed and root mean square speed for a gas ?
View Solutionwhat is the relation between Mass and density.
View SolutionWhat is the relation between mass and number of moles of a gas?
View SolutionWhat is the relation between diamond and graphite ?
View Solution

_S01_028_Q01.png)