If two gases AB2 and B2C are mixed, following equilibria are readily established:
AB2(g)+B2C(g)→AB3(g)+BC(g),
BC(g)+B2C(g)→B3C2(g)
If the reaction started only with AB2 with B2C, then which of the following is necessarily true at equilibrium?
[AB3]eq=[BC]eq
[AB2]eq=[B2C]eq
[AB3]eq>[B3C2]eq
[AB3]eq>[BC]eq
The correct Answer is:C, D
To solve the problem, we need to analyze the two equilibria established when the gases AB2 and B2C are mixed:
1. First Equilibrium:
AB2(g)+B2C(g)⇌AB3(g)+BC(g)
2. Second Equilibrium:
BC(g)+B2C(g)⇌B3C2(g)
Step 1: Initial Conditions
- Let’s assume we start with x moles of AB2 and y moles of B2C. Initially, we have:
- (AB2)=x
- (B2C)=y
- (AB3)=0
- (BC)=0
- (B3C2)=0
Step 2: Change in Concentrations
- Let A moles of AB2 react with B2C to form AB3 and BC. At equilibrium, we have:
- (AB2)=x−A
- (B2C)=y−A
- (AB3)=A
- (BC)=A
Step 3: Second Reaction
- The BC produced can react with B2C to form B3C2. Let Z moles of BC react:
- At equilibrium, we have:
- (BC)=A−Z
- (B2C)=y−A−Z
- (B3C2)=Z
Step 4: Establishing Relationships
- From the above, we can summarize the concentrations at equilibrium:
- (AB2)=x−A
- (B2C)=y−A−Z
- (AB3)=A
- (BC)=A−Z
- (B3C2)=Z
Step 5: Analyzing the Options
1. Option A: (AB3)=(BC)
- A≠A−Z (since Z>0), so this is false.
2. Option B: (AB2)=(B2C)
- x−A and y−A−Z cannot be determined to be equal without knowing x and y, so this is false.
3. Option C: (AB3)>(B3C2)
- A>Z (since Z<A), so this is true.
4. Option D: (AB3)>(BC)
- A>A−Z (since Z>0), so this is true.
Conclusion:
At equilibrium, the necessarily true statements are:
- (AB3)>(B3C2)
- (AB3)>(BC)
AB2(g)+B2C(g)⇌AB3(g)+BC(g)
BC(g)+B2C(g)⇌B3C2(g)
- Let’s assume we start with x moles of AB2 and y moles of B2C. Initially, we have:
- (AB2)=x
- (B2C)=y
- (AB3)=0
- (BC)=0
- (B3C2)=0
- Let A moles of AB2 react with B2C to form AB3 and BC. At equilibrium, we have:
- (AB2)=x−A
- (B2C)=y−A
- (AB3)=A
- (BC)=A
- The BC produced can react with B2C to form B3C2. Let Z moles of BC react:
- At equilibrium, we have:
- (BC)=A−Z
- (B2C)=y−A−Z
- (B3C2)=Z
- From the above, we can summarize the concentrations at equilibrium:
- (AB2)=x−A
- (B2C)=y−A−Z
- (AB3)=A
- (BC)=A−Z
- (B3C2)=Z
1. Option A: (AB3)=(BC)
- A≠A−Z (since Z>0), so this is false.
- x−A and y−A−Z cannot be determined to be equal without knowing x and y, so this is false.
- A>Z (since Z<A), so this is true.
- A>A−Z (since Z>0), so this is true.
At equilibrium, the necessarily true statements are:
- (AB3)>(B3C2)
- (AB3)>(BC)
Topper's Solved these Questions
- CHEMICAL EQUILIBRIUMBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Concept Applicationexercise 7.1
53 Videos
- CHEMICAL EQUILIBRIUMBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Ex 7.2
40 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective
15 Videos
- CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDSEXERCISE - Analytical and Descriptive Type
3 Videos
Similar Questions
Predict ΔH>ΔUorΔH<ΔU.
a. C(graphite)+O2(g)→CO2(g)
b. PCl5(g)→PCl3(g)+Cl2(g)
View SolutionConsider the following reversible chemical reactions :
A2(g)+B2(g)K1⇐⇒2AB(g)....(1)
6AB(g)K2⇐⇒3A2(g)+3B2(g).....(2)
The relation between K1 and K2 is :
View SolutionFor the reaction:
2A(g)+B(g)⇔3C(g)+D(g)
Two moles each of A and B were taken into a flask. The following must always be true when the system attained equilibrium
View SolutionFor the reaction C2H4(g)+H2(g)→C2H6(g) , which of the following expressions between KpandKc is true at 27∘C ?
View Solution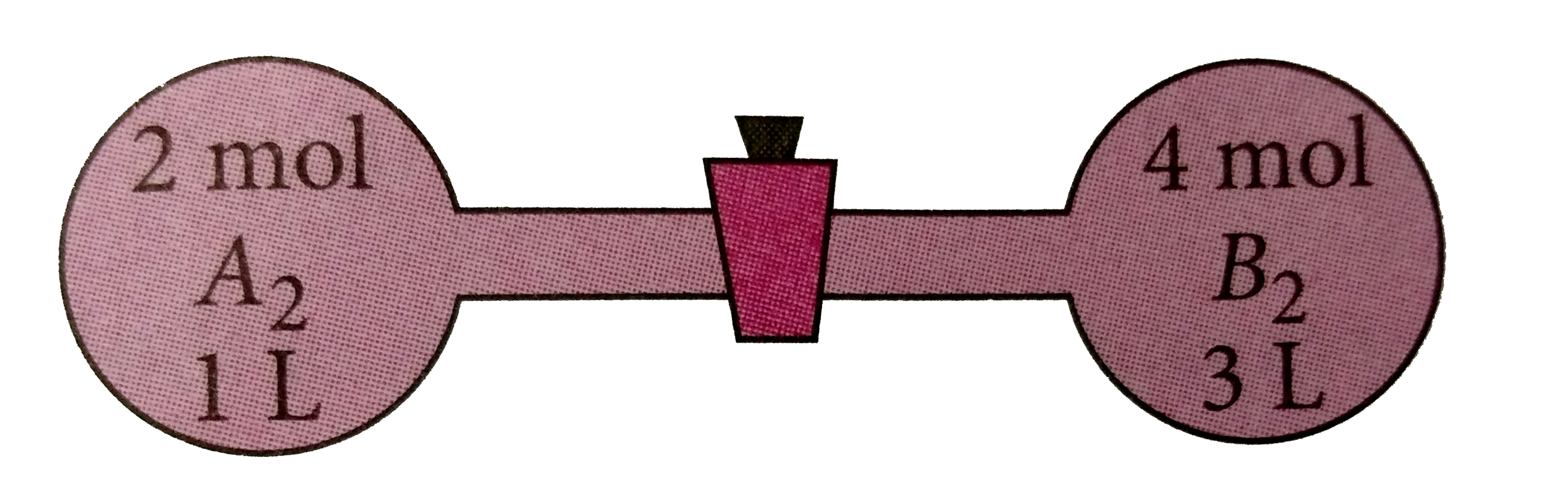
When A2andB2 are allowed to react, the equilibrium constant of the reaction at 27∘C is found (Kc=4).
A2(g)+B2(g)⇔2AB(g)
what will be the equilibrium concentration of AB?
View SolutionThe equilibrium constant for the reaction
A2(g)+B2(g)⇔2AB(g)
is 20 at 500K. The equilibrium constant for the reaction 2AB(g)⇔A2(g)+B2(g) would be
View SolutionIn a reaction A2(g)+4B2(g)⇔2AB4(g),ΔH<0. The formation of AB4 is not favoured by
View SolutionFor the Chemical reaction A2(g)+B2(g)⇔2AB(g) the amount of AB at equilibrium is affected by
View SolutionThe equilibrium constant (Kc) of the reaction A2(g)+B2(g)→2AB(g) is 50. If 1 mol of A2 and 2 mol of B2 are mixed, the amount of AB at equilibrium would be
View SolutionFor a hypothetical reaction of kind.
AB2(g)+12B2(g)⇔AB3(g),ΔH=−xkJ
More AB3 could be produceed at equilibrium by
View Solution
