ΔGɵ for the reaction X+Y⇔C is −4.606kcal at 1000K. The equilibrium constant for the reverse mode of the reaction will be:
100
10
0.01
0.1
The correct Answer is:D
To find the equilibrium constant for the reverse reaction given the standard Gibbs free energy change (ΔG°) for the forward reaction, we can follow these steps:
1. Understand the Relationship Between ΔG° and K:
The relationship between the standard Gibbs free energy change (ΔG°) and the equilibrium constant (K) is given by the equation:
ΔG°=−RTlnK
where R is the universal gas constant and T is the temperature in Kelvin.
2. Convert ΔG° to Appropriate Units:
The given ΔG° is -4.606 kcal. Since we will use R in calories, we need to convert kcal to calories:
−4.606 kcal=−4606 cal
3. Use the Value of R:
The value of R in calories is approximately 2 cal/(K·mol).
4. Plug in the Values:
At T = 1000 K, we can substitute the values into the equation:
−4606=−2×1000lnK
5. Simplify the Equation:
Rearranging the equation gives:
4606=2000lnK
6. Solve for ln K:
Dividing both sides by 2000:
lnK=46062000=2.303
7. Convert ln K to K:
To find K, we exponentiate both sides:
K=e2.303≈10
8. Determine the Equilibrium Constant for the Reverse Reaction:
For the reverse reaction, the equilibrium constant (K') is the reciprocal of K:
K′=1K=110=0.1
Final Answer:
The equilibrium constant for the reverse reaction is 0.1.
The relationship between the standard Gibbs free energy change (ΔG°) and the equilibrium constant (K) is given by the equation:
ΔG°=−RTlnK
where R is the universal gas constant and T is the temperature in Kelvin.
The given ΔG° is -4.606 kcal. Since we will use R in calories, we need to convert kcal to calories:
−4.606 kcal=−4606 cal
The value of R in calories is approximately 2 cal/(K·mol).
At T = 1000 K, we can substitute the values into the equation:
−4606=−2×1000lnK
Rearranging the equation gives:
4606=2000lnK
Dividing both sides by 2000:
lnK=46062000=2.303
To find K, we exponentiate both sides:
K=e2.303≈10
For the reverse reaction, the equilibrium constant (K') is the reciprocal of K:
K′=1K=110=0.1
The equilibrium constant for the reverse reaction is 0.1.
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Concept Applicationexercise 7.153videos
CHEMICAL EQUILIBRIUM
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Ex 7.240videos
CHEMICAL EQUILIBRIUM
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective15videos
CHEMICAL BONDING AND MOLECULAR STRUCTURE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDSEXERCISE - Analytical and Descriptive Type3videos
CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDS
Similar Questions
Knowledge Check
- Question 1 - Select One
The equilibrium constant of a reversible reaction at a given temperature
Adepends on initial concentration of reactantsBdepends on the concentration of products at equilibriumCdoes not depend on the initial concentrationDis not a characteristic of the reaction.
The equilibrium constant for the following reaction is
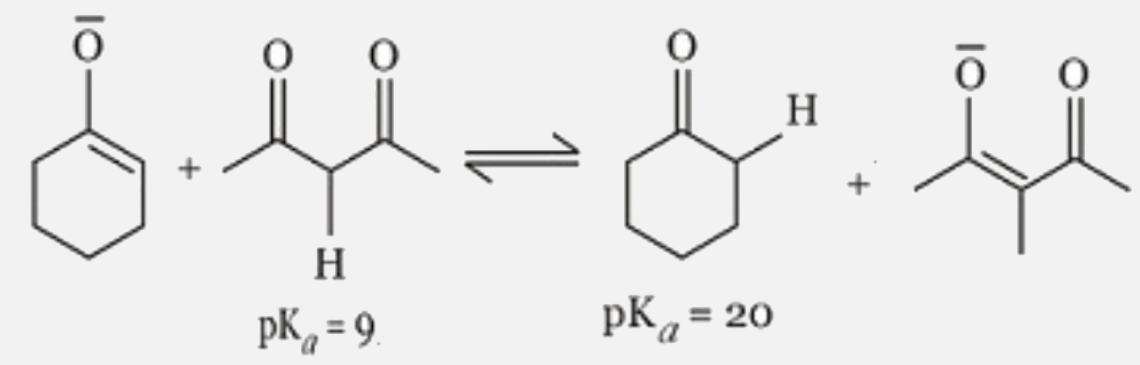 View Solution
View SolutionΔG∘ for the reaction X+Y⇔Z is -4.606 kcal. The value of equilibrium constant of the reaction at 227∘C is (R = 2 Cal/mol K)
View SolutionThe equilibrium constant (K) for the reaction. A+2B⇔2C+D is:
View SolutionIf the equilibrium for a reaction is 4.0 what will be the equilibrium constant for the reverse reaction.
View SolutionThe equilibrium constant for the reactions
A+B⇔AB is 0.5 at 200K. The equilibrium constant for the reaction AB⇔A+B would beView SolutionThe unit of equilibrium constant Kc for the reaction A+B⇔C would be
View SolutionEquilibrium constant of a reaction is related to :
View Solution

