At what concentration of [c−OH] does the following half reaction has a potential of 0V when other species are at 1M?
NOc−3+H2O+2e−→NOc−2+2c−OH,Ec−.cell=−0.06V
2.0M
1.0M
0.1M
0.01M
The correct Answer is:c
To solve the problem, we need to determine the concentration of (OH−) at which the half-reaction has a potential of 0V when all other species are at 1M.
1. Identify the half-reaction and standard potential:
The given half-reaction is:
NO−3+H2O+2e−→NO−2+2OH−
The standard cell potential E∘cell is given as −0.06V.
2. Set up the Nernst equation:
The Nernst equation for the half-reaction can be written as:
Ecell=E∘cell−0.059nlogKeq
where n is the number of electrons transferred (which is 2 in this case).
3. Set the cell potential to 0V:
We want to find the concentration of (OH−) when Ecell=0V. Thus, we can set up the equation:
0=−0.06−0.0592logKeq
4. Calculate Keq:
Rearranging the equation gives:
0.06=0.0592logKeq
Multiplying both sides by 2:
0.12=0.059logKeq
Dividing by 0.059:
logKeq=0.120.059≈2.0339
Therefore, Keq=102.0339≈108.7.
5. Express Keq in terms of concentrations:
The equilibrium constant Keq for the reaction can be expressed as:
Keq=(NO−2)(OH−)2(NO−3)(H2O)
Since (NO−3) and (H2O) are both 1M, we simplify this to:
Keq=(NO−2)(OH−)2
6. Assume (NO−2)=1M:
Substituting (NO−2)=1M into the equation gives:
Keq=1⋅(OH−)2
Thus, we have:
108.7=(OH−)2
7. Solve for (OH−):
Taking the square root of both sides:
(OH−)=√108.7≈10.43
8. Final concentration:
Since we want the concentration of (OH−) to be in molarity, we can express it as:
(OH−)≈0.1M
Conclusion:
The concentration of (OH−) at which the half-reaction has a potential of 0V when other species are at 1M is approximately 0.1M.
The given half-reaction is:
NO−3+H2O+2e−→NO−2+2OH−
The standard cell potential E∘cell is given as −0.06V.
The Nernst equation for the half-reaction can be written as:
Ecell=E∘cell−0.059nlogKeq
where n is the number of electrons transferred (which is 2 in this case).
We want to find the concentration of (OH−) when Ecell=0V. Thus, we can set up the equation:
0=−0.06−0.0592logKeq
Rearranging the equation gives:
0.06=0.0592logKeq
Multiplying both sides by 2:
0.12=0.059logKeq
Dividing by 0.059:
logKeq=0.120.059≈2.0339
Therefore, Keq=102.0339≈108.7.
The equilibrium constant Keq for the reaction can be expressed as:
Keq=(NO−2)(OH−)2(NO−3)(H2O)
Since (NO−3) and (H2O) are both 1M, we simplify this to:
Keq=(NO−2)(OH−)2
Substituting (NO−2)=1M into the equation gives:
Keq=1⋅(OH−)2
Thus, we have:
108.7=(OH−)2
Taking the square root of both sides:
(OH−)=√108.7≈10.43
Since we want the concentration of (OH−) to be in molarity, we can express it as:
(OH−)≈0.1M
The concentration of (OH−) at which the half-reaction has a potential of 0V when other species are at 1M is approximately 0.1M.
Topper's Solved these Questions
- ELECTROCHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Ex 3.2 (Objective)
26 Videos
- ELECTROCHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Ex 3.3 (Objective)
10 Videos
- ELECTROCHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Solved Examples(Electrolysis And Electrolytic Cells)
12 Videos
- D AND F BLOCK ELEMENTSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - D AND F BLOCK ELEMENTSEXERCISE - Archives Subjective
29 Videos
- GENERAL PRINCIPLES AND PROCESS OF ISOLATION OF ELEMENTSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - GENERAL PRINCIPLES AND PROCESS OF ISOLATION OF ELEMENTSEXERCISE - Archives (Subjective)
14 Videos
Similar Questions
Knowledge Check
Rank the transition states that occur during the following reaction steps in order of increasing stability (least → most stable) :
1. H3C−+OH2→CH+3+H2O
2. (CH3)3C−+CH2→(CH3)3C++H2O
3. (CH3)2CH−+OH2→(CH3)2CH++H2OA1<2<3B2<3<1C1<3<2D2<1<3
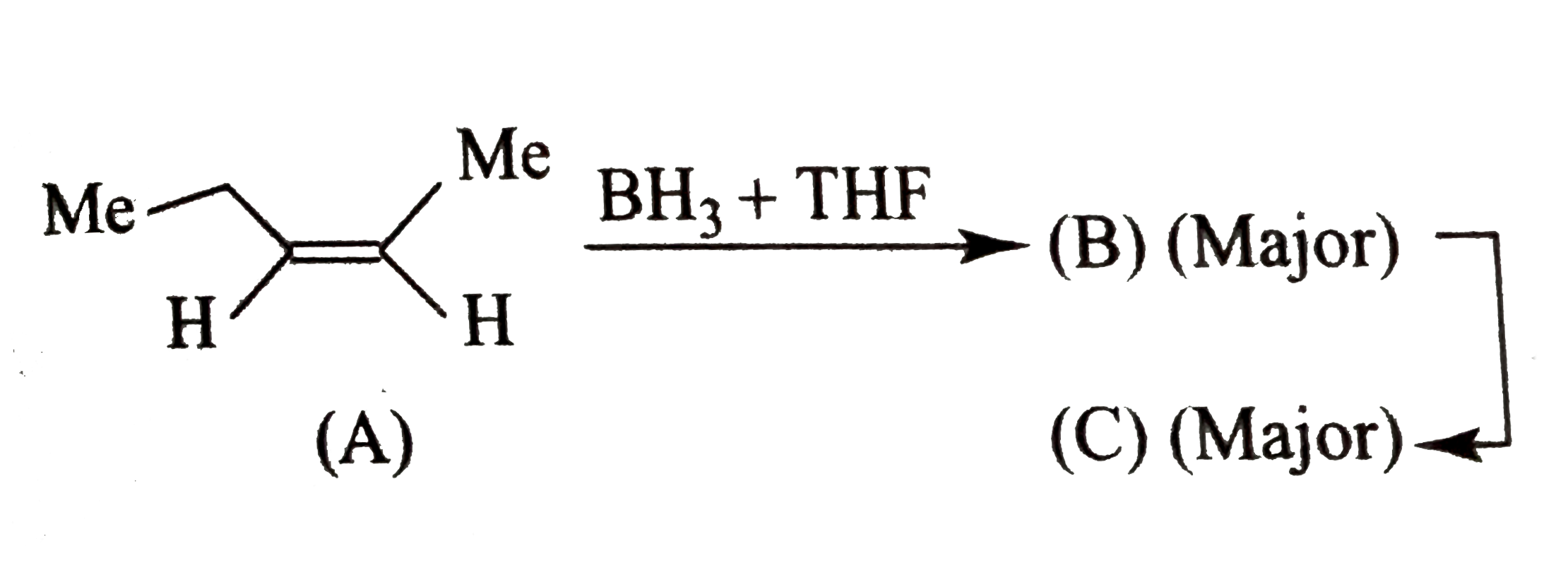 H2O2+ΘOH
H2O2+ΘOH
B and C are:
View SolutionComplete the following reactions :
C2H4+H2OH2SO4−−−→
View SolutionIn the following reaction 'A' is :
C2H5MgBr+epoxyethaneH2O−−→A
View SolutionThe standard reduction potential for the half cell :
NOc−3(aq)+2Hc−+e−→NO2(g)+H2O is 0.78V.
Calculate the reduction potential in 8MH⊕.
View SolutionThe decreasing basic order of the following is:
i. PhSOo−3, ii. C2H5SOo−3, iii. C2H5COOo−, iv. o−CN, v. o−OH
View SolutionIn the following reaction, trace the position of isotopic O18
CH3−O∣∣C−O−H+CH3CH2−18O−H⇔CH3−O∣∣C−O−CH2CH3
+H2O
View SolutionPh−C≡CHHg+,H+/H2O−−−−−−−−−→A
Aditon of H2O in the reaction is an example of
View Solution
