The reaction
2NO2→2NO+O2
has an activation energy of 110KJmol−. At 400∘C, the rate constant is 7.8mol−1Ls−1. What is the value of rate constant at 430∘C ?
To find the rate constant k2 at 430∘C for the reaction 2NO2→2NO+O2, we will use the Arrhenius equation in the logarithmic form:
logk2k1=Ea2.303R⋅T2−T1T1T2
Step 1: Convert temperatures to Kelvin
- T1=400∘C=400+273=673K
- T2=430∘C=430+273=703K
Step 2: Write down the given values
- Activation energy Ea=110kJ/mol=110×103J/mol
- Rate constant at T1 (k1)=7.8mol−1Ls−1
- Gas constant R=8.314J/(mol⋅K)
Step 3: Substitute the values into the equation
Now we substitute the values into the logarithmic form of the Arrhenius equation:
logk27.8=110×1032.303×8.314⋅703−673673×703
Step 4: Calculate the temperature difference and the denominator
- T2−T1=703−673=30K
- T1T2=673×703
Calculating T1T2:
673×703=472,219K2
Step 5: Calculate the right-hand side
Now we calculate the right-hand side of the equation:
110×1032.303×8.314⋅30472219
Calculating 2.303×8.314:
2.303×8.314≈19.189
Now substituting back:
11000019.189⋅30472219≈5725.5⋅30472219≈5725.5⋅0.0000635≈0.3643
Step 6: Solve for k2
Now we have:
logk27.8=0.3643
Taking the antilog:
k27.8=100.3643≈2.31
Now, multiplying both sides by 7.8:
k2=2.31×7.8≈18.018mol−1Ls−1
Final Answer
Thus, the rate constant at 430∘C is approximately:
k2≈18mol−1Ls−1
---
- T1=400∘C=400+273=673K
- T2=430∘C=430+273=703K
- Activation energy Ea=110kJ/mol=110×103J/mol
- Rate constant at T1 (k1)=7.8mol−1Ls−1
- Gas constant R=8.314J/(mol⋅K)
Now we substitute the values into the logarithmic form of the Arrhenius equation:
- T2−T1=703−673=30K
- T1T2=673×703
Now we calculate the right-hand side of the equation:
Now we have:
Thus, the rate constant at 430∘C is approximately:
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Solved Example52videos
CHEMICAL KINETICS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex 4.1 (Objective)9videos
CHEMICAL KINETICS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)34videos
CARBOXYLIC ACIDS AND THEIR DERIVATIVES
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective18videos
COORDINATION COMPOUNDS
Similar Questions
The activation energy of a reaction is 50 kJ mol-1 and the value of rate constant at 300 K is 2.5×10−5sec−1. What is the value of the frequency factor, A?
View SolutionThe activation energy of the reaction: A+B→ Products is 105.73kJmol−1. At 40∘C, the Products are found at the rate of 0.133molL−1−1min. What will be the rate of formation of Products at 80∘C?
View Solution
Knowledge Check
- Question 1 - Select One
Consider the reaction, 2N2O5→4NO2+O2 In the reaction NO2 is being formed at the rate of 0.0125 mol L−1s−1. What is the rate of reaction at this time?
A0.0018 mol L−1s−1B0.0031 mol L−1s−1C0.0041 mol L−1s−1D0.050 mol L−1s−1
An exothermic reaction, A→B, has an activation energy of 15 kcal mol−1 and the energy of reaction is 5 kcal mol−1. The activation energy in kcal mol−1 for the reaction, B→A is
View SolutionRate constant of a reaction (k) is 175litre2mol−2sec−1. What is the order of reaction ?
View SolutionIn an exothermic reaction X→Y, the activation energy is 100kJmol−1 of X. The enthalphy of the reaction is −140kJmol−1. The activation energy of the reverse reaction Y→X is
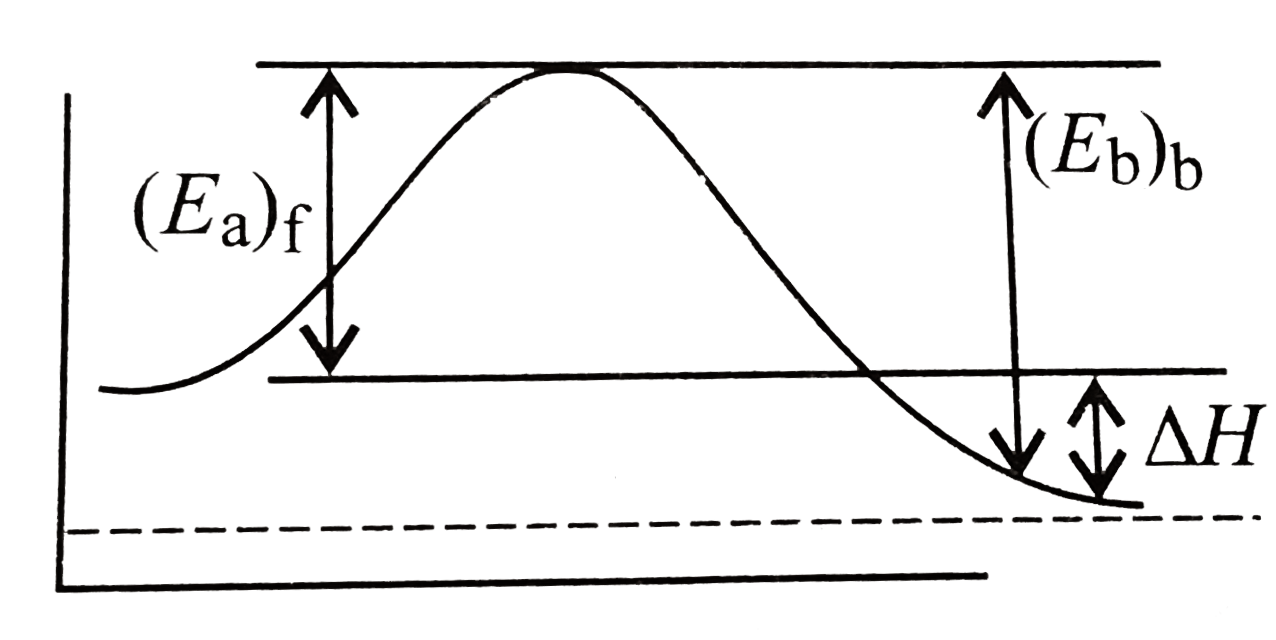 View Solution
View SolutionThe activation energy of a reaction is 94.14kJmol−1 and the value of rate constant at 313K is 18×10−5s−1. Calculate the frequency factor or pre-exponential factor, A.
View SolutionThe decomposition of phosphine 4PH3(g)→P4(g)+6H2(g) has the rate law, Rate=k[PH3]
The rate constant is6.0×10−4s−1 at 300 K and activation energy is 3.05×105Jmol−1 . What is the value of rate constant at 310 K? [R = 8.314JK−1mol−1]View SolutionThe decomposition of phosphine 4PH3(g)→P4(g)+6H2(g) has the rate law, Rate=k[PH3]
The rate constant is6.0×10−4s−1 at 300 K and activation energy is 3.05×105Jmol−1 . What is the value of rate constant at 310 K? [R = 8.314JK−1mol−1]View SolutionThe decomposition of phosphine 4PH3(g)→P4(g)+6H2(g) has the rate law, Rate=k[PH3]
The rate constant is6.0×10−4s−1 at 300 K and activation energy is 3.05×105Jmol−1 . What is the value of rate constant at 310 K? [R = 8.314JK−1mol−1]View Solution

