From the concetration of (butyl chloride) at different times given below, calculate the average rate of reaction:
during different intervals of time.
To calculate the average rate of the reaction of butyl chloride (C₄H₉Cl) with water over different time intervals, we will follow these steps:
1. Identify the Reaction:
The reaction is:
C4H9Cl+H2O→C4H9OH+HCl
The average rate of reaction can be calculated based on the disappearance of butyl chloride.
2. Average Rate Formula:
The average rate of the reaction can be expressed as:
Average Rate=−Δ(C4H9Cl)Δt
where Δ(C4H9Cl) is the change in concentration of butyl chloride and Δt is the change in time.
3. Data Given:
The concentrations of butyl chloride at different times are:
- At t=0 s, (C4H9Cl)=0.100 mol/L
- At t=50 s, (C4H9Cl)=0.0905 mol/L
- At t=100 s, (C4H9Cl)=0.0820 mol/L
- At t=150 s, (C4H9Cl)=0.0741 mol/L
- At t=200 s, (C4H9Cl)=0.0671 mol/L
- At t=300 s, (C4H9Cl)=0.0549 mol/L
- At t=400 s, (C4H9Cl)=0.0439 mol/L
- At t=700 s, (C4H9Cl)=0.0210 mol/L
- At t=800 s, (C4H9Cl)=0.0170 mol/L
4. Calculate Average Rates for Each Interval:
We will calculate the average rate for each time interval using the formula provided.
- Interval 0 to 50 s:
Average Rate=−0.0905−0.10050−0=−−0.009550=1.9×10−4mol L−1s−1
- Interval 50 to 100 s:
Average Rate=−0.0820−0.0905100−50=−−0.008550=1.7×10−4mol L−1s−1
- Interval 100 to 150 s:
Average Rate=−0.0741−0.0820150−100=−−0.007950=1.58×10−4mol L−1s−1
- Interval 150 to 200 s:
Average Rate=−0.0671−0.0741200−150=−−0.007050=1.40×10−4mol L−1s−1
- Interval 200 to 300 s:
Average Rate=−0.0549−0.0671300−200=−−0.0122100=1.22×10−4mol L−1s−1
- Interval 300 to 400 s:
Average Rate=−0.0439−0.0549400−300=−−0.0110100=1.10×10−4mol L−1s−1
- Interval 400 to 700 s:
Average Rate=−0.0210−0.0439700−400=−−0.0229300=7.63×10−5mol L−1s−1
- Interval 700 to 800 s:
Average Rate=−0.0170−0.0210800−700=−−0.0040100=4.00×10−5mol L−1s−1
5. Summary of Average Rates:
- From 0 to 50 s: 1.9×10−4mol L−1s−1
- From 50 to 100 s: 1.7×10−4mol L−1s−1
- From 100 to 150 s: 1.58×10−4mol L−1s−1
- From 150 to 200 s: 1.40×10−4mol L−1s−1
- From 200 to 300 s: 1.22×10−4mol L−1s−1
- From 300 to 400 s: 1.10×10−4mol L−1s−1
- From 400 to 700 s: 7.63×10−5mol L−1s−1
- From 700 to 800 s: 4.00×10−5mol L−1s−1
The reaction is:
C4H9Cl+H2O→C4H9OH+HCl
The average rate of reaction can be calculated based on the disappearance of butyl chloride.
The average rate of the reaction can be expressed as:
Average Rate=−Δ(C4H9Cl)Δt
where Δ(C4H9Cl) is the change in concentration of butyl chloride and Δt is the change in time.
The concentrations of butyl chloride at different times are:
- At t=0 s, (C4H9Cl)=0.100 mol/L
- At t=50 s, (C4H9Cl)=0.0905 mol/L
- At t=100 s, (C4H9Cl)=0.0820 mol/L
- At t=150 s, (C4H9Cl)=0.0741 mol/L
- At t=200 s, (C4H9Cl)=0.0671 mol/L
- At t=300 s, (C4H9Cl)=0.0549 mol/L
- At t=400 s, (C4H9Cl)=0.0439 mol/L
- At t=700 s, (C4H9Cl)=0.0210 mol/L
- At t=800 s, (C4H9Cl)=0.0170 mol/L
We will calculate the average rate for each time interval using the formula provided.
Average Rate=−0.0905−0.10050−0=−−0.009550=1.9×10−4mol L−1s−1
Average Rate=−0.0820−0.0905100−50=−−0.008550=1.7×10−4mol L−1s−1
Average Rate=−0.0741−0.0820150−100=−−0.007950=1.58×10−4mol L−1s−1
Average Rate=−0.0671−0.0741200−150=−−0.007050=1.40×10−4mol L−1s−1
Average Rate=−0.0549−0.0671300−200=−−0.0122100=1.22×10−4mol L−1s−1
Average Rate=−0.0439−0.0549400−300=−−0.0110100=1.10×10−4mol L−1s−1
Average Rate=−0.0210−0.0439700−400=−−0.0229300=7.63×10−5mol L−1s−1
Average Rate=−0.0170−0.0210800−700=−−0.0040100=4.00×10−5mol L−1s−1
- From 0 to 50 s: 1.9×10−4mol L−1s−1
- From 50 to 100 s: 1.7×10−4mol L−1s−1
- From 100 to 150 s: 1.58×10−4mol L−1s−1
- From 150 to 200 s: 1.40×10−4mol L−1s−1
- From 200 to 300 s: 1.22×10−4mol L−1s−1
- From 300 to 400 s: 1.10×10−4mol L−1s−1
- From 400 to 700 s: 7.63×10−5mol L−1s−1
- From 700 to 800 s: 4.00×10−5mol L−1s−1
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Solved Example
52 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex 4.1 (Objective)
9 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
Knowledge Check
If the concentration of glucose (C6H12O6) in blood is 0.9 g L−1, what will be the molarity of glucose in blood?
A5 MB50 MC0.005 MD0.5 M
From the data given below, calculate the average rate of the reaction :
C4H9Cl+H2O→C4H9OH+HCl during different intervals of time.
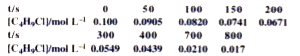
View SolutionIf the concentration of glucose (C6H12O6) in blood is 0.9 g L−1, what will be the molarity of glucose in blood?
View SolutionFrom the equation 4HCl+O2→2H2O+2Cl2, compute the moles of HCl needed to form 0.35 mol Cl2.
View SolutionWhat is the pH of a 0.10 M C6H5O− solution? The Ka of C6H5OH is1.0×10−10
View SolutionSO2Cl2 (sulphuryl chloride ) reacts with water to given a mixture of H2SO4 and HCL. What volume of 0.2 M Ba(OH)2 is needed to completely neutralize 25 mL of 0.2 MSO2Cl2 solution:
View SolutionConisder the reaction represented by the equation:
CH3Cl(g)+H2O(g)→CH3OH(g)+HCl(g)
These kinetics data were obtained for the given reaction concentrations:
Initial conc (M)Initial rate of disappearance[CH3Cl][H2O]of CH3Cl(Ms−1)0.20.210.40.220.40.48
Unit of rate constant will be
View SolutionCalculate ΔH at 85∘C for the reaction:
Fe2O3(s)+3H2(g)→2Fe(s)+3H2O(l)
The data: ΔHΘ298=−33.0kJmol−1 and SubstanceFe2O3(s)Fe(s)H2O(l)H2(g)C∘P(JK−1mol)103.025.075.028.0
View Solution
