Assertion (A) : For a first order, the concentration of a reaction decreases exponentially with time.
Reason (R ): The rate of reaction at any time depends upon the concentration of the reactant at that time.
If both (A) and (R) are correct, and (R) is the correct explnation of (A).
If both (A) and (R) are correct, but (R) is noth the correct explanation of (A).
If (A) is correct, but (R) is incorrect.
If (A) is incorrect, but (R) is correct.
The correct Answer is:B
To solve the given question, we need to analyze both the Assertion (A) and Reason (R) provided in the question.
Step 1: Understand the Assertion
The assertion states that "For a first order reaction, the concentration of a reaction decreases exponentially with time."
- For a first-order reaction, the rate of reaction is proportional to the concentration of the reactant. The mathematical representation of a first-order reaction can be expressed as:
Rate=k(A)
where k is the rate constant and (A) is the concentration of the reactant.
- The integrated rate law for a first-order reaction is given by:
(A)=(A0)e−kt
where (A0) is the initial concentration and t is time. This equation shows that the concentration decreases exponentially over time.
Step 2: Understand the Reason
The reason states that "The rate of reaction at any time depends upon the concentration of the reactant at that time."
- This statement is also true. In a first-order reaction, the rate of reaction at any given time is indeed dependent on the concentration of the reactant at that time. As the concentration decreases, the rate of reaction also decreases.
Step 3: Determine the Relationship Between Assertion and Reason
Now, we need to evaluate the relationship between the assertion and the reason:
- Both the assertion and the reason are correct statements.
- However, the reason provided does not serve as a direct explanation for the assertion. The assertion describes the behavior of concentration over time, while the reason describes the dependency of the rate on concentration, which is a separate concept.
Conclusion
Based on the analysis:
- Assertion (A) is correct.
- Reason (R) is correct.
- However, Reason (R) is not the correct explanation for Assertion (A).
Thus, the correct answer is that both the assertion and reason are correct, but the reason is not the correct explanation for the assertion.
Final Answer
The answer is option (B): Both Assertion and Reason are correct, but Reason is not the correct explanation for Assertion.
The assertion states that "For a first order reaction, the concentration of a reaction decreases exponentially with time."
Rate=k(A)
The reason states that "The rate of reaction at any time depends upon the concentration of the reactant at that time."
Now, we need to evaluate the relationship between the assertion and the reason:
- However, the reason provided does not serve as a direct explanation for the assertion. The assertion describes the behavior of concentration over time, while the reason describes the dependency of the rate on concentration, which is a separate concept.
Based on the analysis:
- Reason (R) is correct.
- However, Reason (R) is not the correct explanation for Assertion (A).
The answer is option (B): Both Assertion and Reason are correct, but Reason is not the correct explanation for Assertion.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Integer
15 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Fill In The Blanks
37 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Single Correct
177 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
Knowledge Check
Consider the reaction A→B. The concentration of both the reactants and the products varies exponentially with time. Which of the following figure correctly describes the change in concentration of reactants and products with time ?
A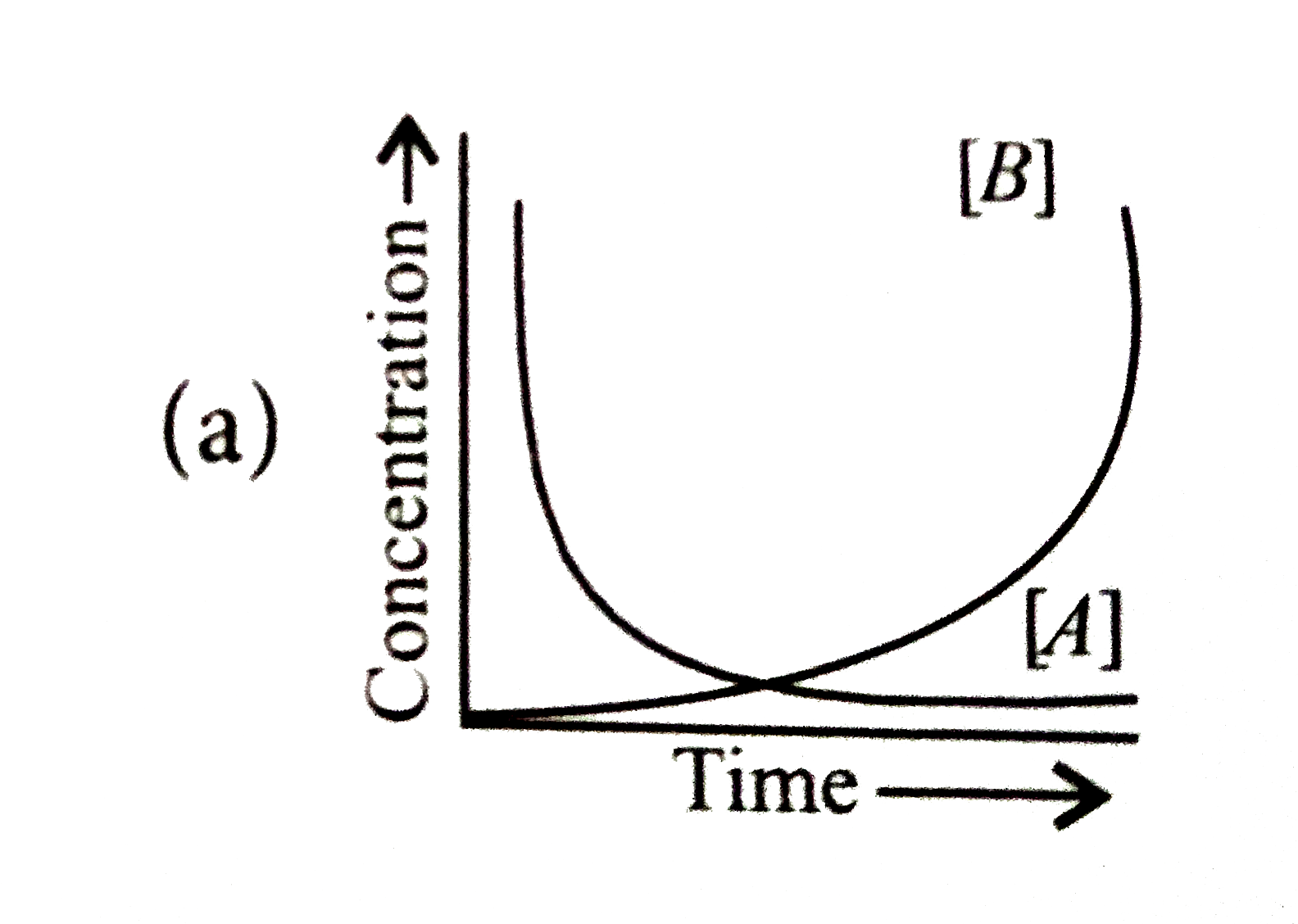 B
B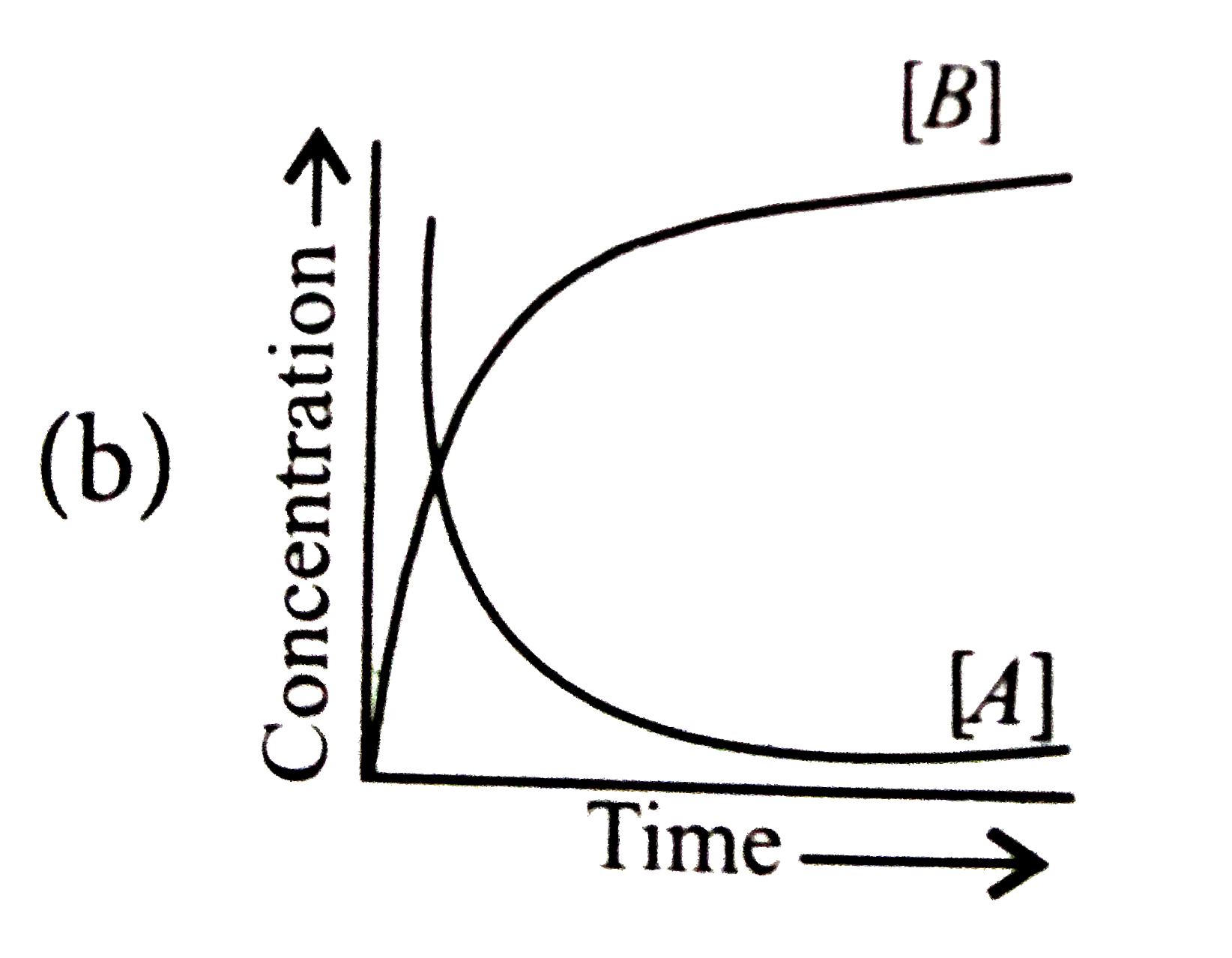 C
C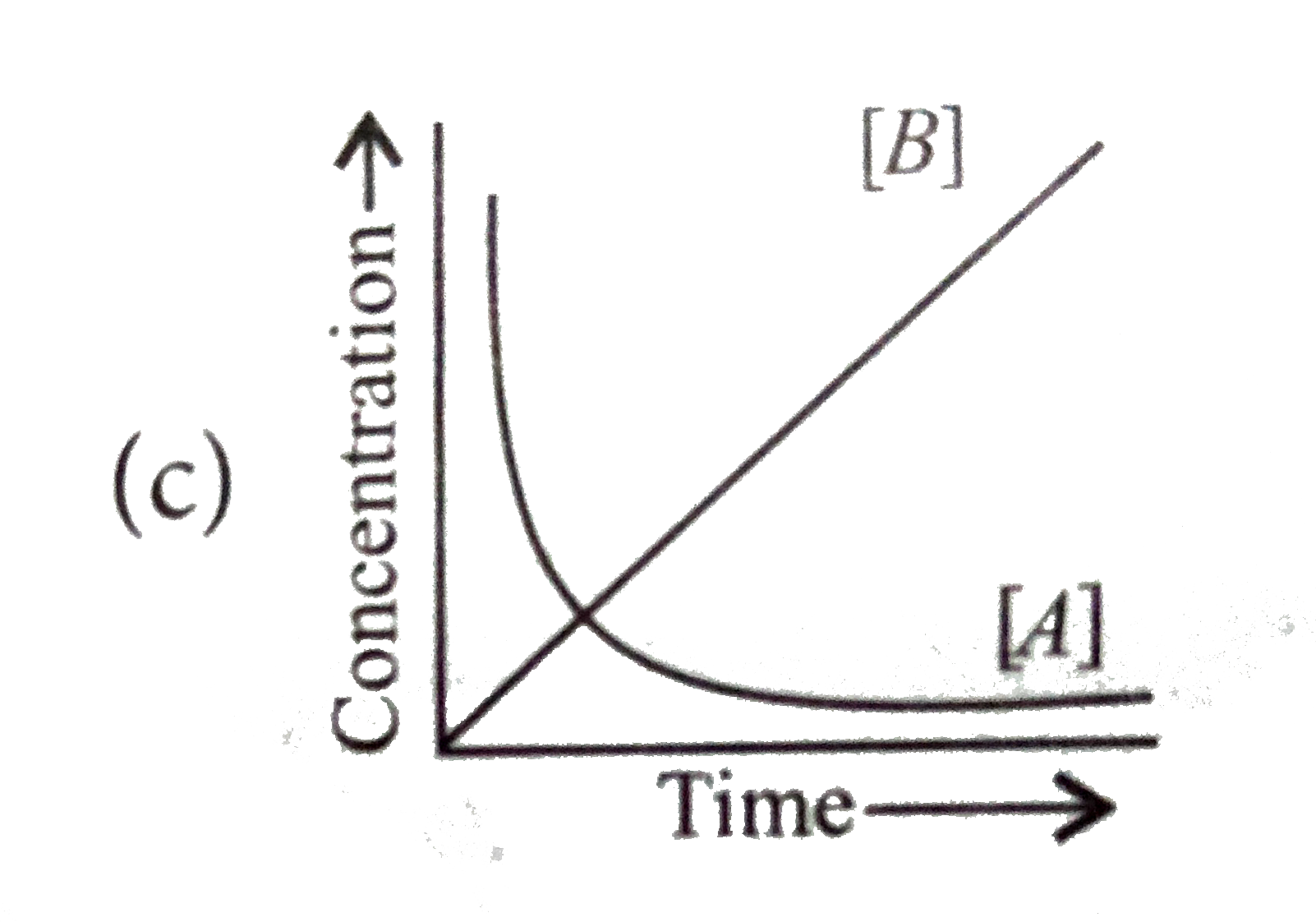 D
D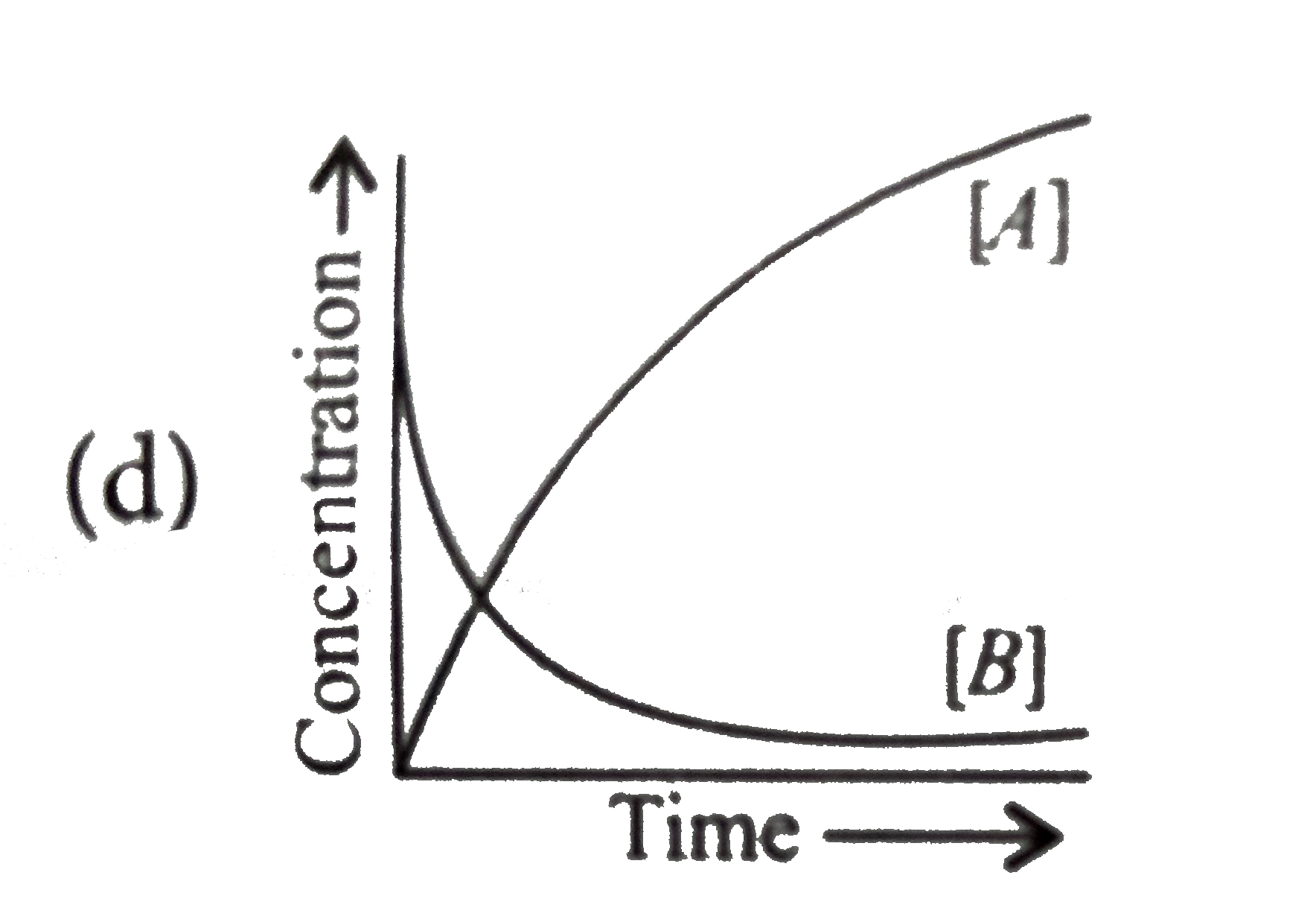
The rate constant of any reaction is proportional to the concentration of the reactants.
View SolutionFor a first order reaction, the rate of the reaction doubled as the concentration of the reactant is doubled.
View SolutionThe rate constant of a first order reaction is proportional to the concentration of the reactant.
View SolutionThe rate constant of a first order reaction is proportional to the concentration of the reactant.
View SolutionThe rate constant of a first order reaction is proportional to the concentration of the reactant.
View SolutionConsider the reaction A→B. The concentration of both the reactants and the products varies exponentially with time. Which of the following figure correctly describes the change in concentration of reactants and products with time ?
View SolutionIf the concentration of the reactants is increased, the rate of reaction for first order-
View Solution