10dm3 of a liquid A was mixed with 10dm3 of liquid B . The volume of the resulting solutino was found to be 19dm3. What do you conclude ?
To solve the problem, we need to analyze the information given about the mixing of two liquids, A and B.
1. Identify the volumes of liquids A and B:
- Volume of liquid A (V_A) = 10 dm³
- Volume of liquid B (V_B) = 10 dm³
2. Calculate the total volume if there were no volume change upon mixing:
- If there were no volume change, the total volume (V_A + V_B) would be:
VA+VB=10dm3+10dm3=20dm3
3. Determine the actual volume of the mixture:
- The volume of the resulting solution after mixing (V_mix) is given as:
Vmix=19dm3
4. Compare the actual volume with the expected volume:
- We observe that:
V_{mix} < V_A + V_B \quad \text{(19 dm³ < 20 dm³)}
5. Conclusion based on the comparison:
- Since the volume of the mixture (19 dm³) is less than the sum of the individual volumes (20 dm³), this indicates that the solution exhibits a negative deviation from Raoult's law.
6. Understanding the reason for negative deviation:
- In a non-ideal solution that shows negative deviation, the intermolecular forces between the molecules of different components (A and B) are stronger than the forces between the molecules of the same component. This leads to a decrease in volume upon mixing.
Final Conclusion:
The mixing of 10 dm³ of liquid A and 10 dm³ of liquid B resulting in a total volume of 19 dm³ indicates that the solution is a non-ideal solution showing negative deviation from Raoult's law.
---
- Volume of liquid A (V_A) = 10 dm³
- Volume of liquid B (V_B) = 10 dm³
- If there were no volume change, the total volume (V_A + V_B) would be:
VA+VB=10dm3+10dm3=20dm3
- The volume of the resulting solution after mixing (V_mix) is given as:
Vmix=19dm3
- We observe that:
V_{mix} < V_A + V_B \quad \text{(19 dm³ < 20 dm³)}
- Since the volume of the mixture (19 dm³) is less than the sum of the individual volumes (20 dm³), this indicates that the solution exhibits a negative deviation from Raoult's law.
- In a non-ideal solution that shows negative deviation, the intermolecular forces between the molecules of different components (A and B) are stronger than the forces between the molecules of the same component. This leads to a decrease in volume upon mixing.
The mixing of 10 dm³ of liquid A and 10 dm³ of liquid B resulting in a total volume of 19 dm³ indicates that the solution is a non-ideal solution showing negative deviation from Raoult's law.
Topper's Solved these Questions
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - NCERT Exercise
104 Videos
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Short Answer Type Questions(ElectroCHMmical Cell)
4 Videos
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - NCERT Exerise
25 Videos
- GRIGNARD REAGENTS AND ORGANOMETALLIC REAGENTSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - GRIGNARD REAGENTS AND ORGANOMETALLIC REAGENTSEXERCISE - Exercises Archives (Linked Comprehension)
1 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Archives Subjective
13 Videos
Similar Questions
11ml of liquid A was mixed with 10ml of liquid B. The volume of the resulting solution was found to be 19.9ml what do you conclude?
View Solution10 mL of a liquid A were mixed with 10 mL of liquid B. The volume of resulting solution was found to be 19.9 mL. What do you conclude?
View SolutionTwo liquids A and B are mixed and the resulting solution is found to be cooler. What do you conclude about the deviation from ideal behaviour?
View SolutionMolar volume of an Ideal gas is 0.45dm3/mol. The molar volume of air (assumming as real gas) under the same condition is 0.9dm3/mol. The point which corresponds to air the given graph is :
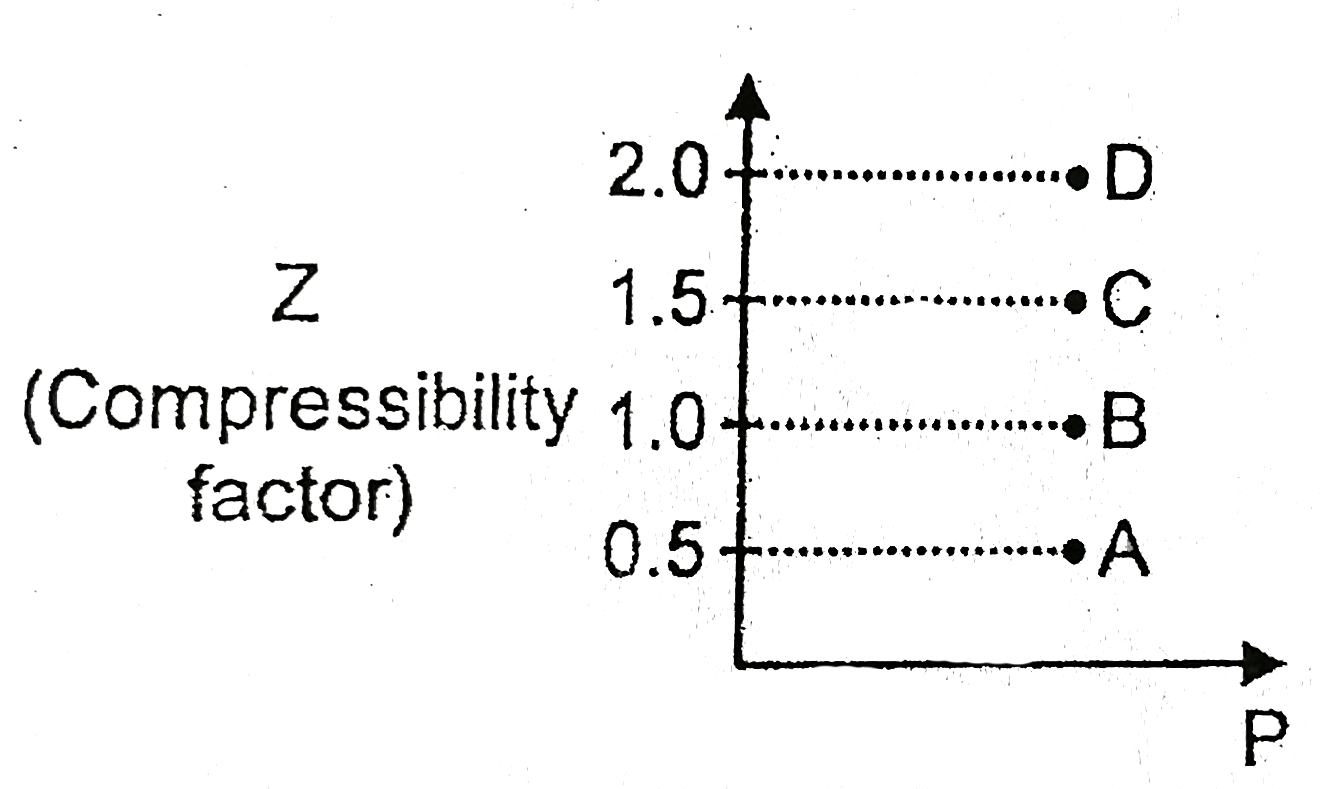
View SolutionThe volume of 2N H2SO4 solution is 0.1dm3. The volume of its decinormal solution (in dm3) will be
View SolutionWhat is the volume (measured in dm3 or litres) occupied by one mole of at at S.T.P.?
View Solution10dm3 of N2 gas and 10dm3 of gas X contain the same number of molecules at the same temperature, the gas (X) may be
View SolutionA sample of a gas occupies 100dm3 at 1 bar pressure and at 25oC.If the volume of the gas is reduced to 5 dm3 at the same temperature, what additional pressure must be be applied?
View SolutionA sample of a gas occupies 100 dm3 at 1 bar pressure and at 27oC.If the volume of the gas is reduced to 5dm3 at the same temperature, what additional pressure must be be applied?
View SolutionA sample of a gas occupies 100 dm3 at 1 bar pressure and at 27oC.If the volume of the gas is reduced to 25dm3 at the same temperature, what additional pressure must be be applied?
View Solution
