Assertion The total number of isomers shown by [Co(en)2CI2]⊕ complex ion is three
[Co(en)2CI2]2+ complex ion has an octahedral geometry .
If both (A) and (R) are correct and (R) is the correct explanation of (A) .
If both (A) and (R) are correct and (R) is the correct explanation of (A) .
If(A) is correct, but (R) is incorrect .
Both (A) and (R) are incorrect .
The correct Answer is:B
To solve the question regarding the assertion and reason about the coordination compound (Co(en)2Cl2)+, we will follow these steps:
Step 1: Determine the Coordination Number
The coordination number of a complex is determined by the number of donor atoms from the ligands attached to the central metal ion. In this case, we have:
- Ethylenediamine (en) is a bidentate ligand, meaning it donates 2 donor atoms.
- Chloride ions (Cl) are monodentate ligands, meaning each donates 1 donor atom.
Calculating the coordination number:
- From 2 ethylenediamine ligands: 2×2=4
- From 2 chloride ions: 2×1=2
Thus, the total coordination number is:
4+2=6
Step 2: Identify the Geometry
With a coordination number of 6, the geometry of the complex is octahedral. This can be confirmed as follows:
- For coordination number 6, the possible geometries are octahedral.
Step 3: Determine the Isomers
In an octahedral complex with the formula (Co(en)2Cl2)+, we can have different types of isomers:
1. Cis Isomer: Both chloride ions are adjacent to each other.
2. Trans Isomer: The chloride ions are opposite each other.
Additionally, the cis isomer can exist in two optically active forms (D and L forms) due to the presence of chiral centers created by the bidentate ligands. Therefore, we can summarize the isomers as follows:
- 1 Trans Isomer
- 1 Cis Isomer (which is optically active)
- 1 Mirror Image of the Cis Isomer (which is also optically active)
Thus, the total number of isomers is:
1 (trans)+1 (cis)+1 (mirror image of cis)=3
Step 4: Conclusion on Assertion and Reason
- The assertion states that the total number of isomers is three, which we have confirmed to be true.
- The reason states that the geometry is octahedral, which is also true.
However, the reason does not correctly explain the assertion because the number of isomers cannot be solely determined by the geometry; it also depends on the arrangement of the ligands and their symmetry. Therefore, while both the assertion and reason are correct, the reason is not the correct explanation for the assertion.
Final Answer
The answer to the question is option B: The assertion is correct, but the reason is not the correct explanation of the assertion.
---
The coordination number of a complex is determined by the number of donor atoms from the ligands attached to the central metal ion. In this case, we have:
- Ethylenediamine (en) is a bidentate ligand, meaning it donates 2 donor atoms.
- Chloride ions (Cl) are monodentate ligands, meaning each donates 1 donor atom.
- From 2 ethylenediamine ligands: 2×2=4
- From 2 chloride ions: 2×1=2
4+2=6
With a coordination number of 6, the geometry of the complex is octahedral. This can be confirmed as follows:
- For coordination number 6, the possible geometries are octahedral.
In an octahedral complex with the formula (Co(en)2Cl2)+, we can have different types of isomers:
1. Cis Isomer: Both chloride ions are adjacent to each other.
2. Trans Isomer: The chloride ions are opposite each other.
- 1 Trans Isomer
- 1 Cis Isomer (which is optically active)
- 1 Mirror Image of the Cis Isomer (which is also optically active)
1 (trans)+1 (cis)+1 (mirror image of cis)=3
- The assertion states that the total number of isomers is three, which we have confirmed to be true.
- The reason states that the geometry is octahedral, which is also true.
The answer to the question is option B: The assertion is correct, but the reason is not the correct explanation of the assertion.
Topper's Solved these Questions
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Exercises Integer (Naming And Terminology)
16 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Exercises Integer (Isomerism)
15 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Exercises Single Correct (Application Of Coordination Compounds And Miscellaneous)
28 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Archives Subjective
23 Videos
- D AND F BLOCK ELEMENTSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - D AND F BLOCK ELEMENTSEXERCISE - Archives Subjective
29 Videos
Similar Questions
Total number of stereoisomers in [Co(en)2Br2]+ complex are
View SolutionThe possible number of the optical isomers in [Cr(en)2CI2]⊕ is .
View SolutionWhich complex ion has cis and trans isomer ?
View SolutionGive the number of possibel isomers of
[CrCl2en2]⊕ .
View SolutionFind the number of geometrical isomers in [Co(en)(Pn)(NO2)2]
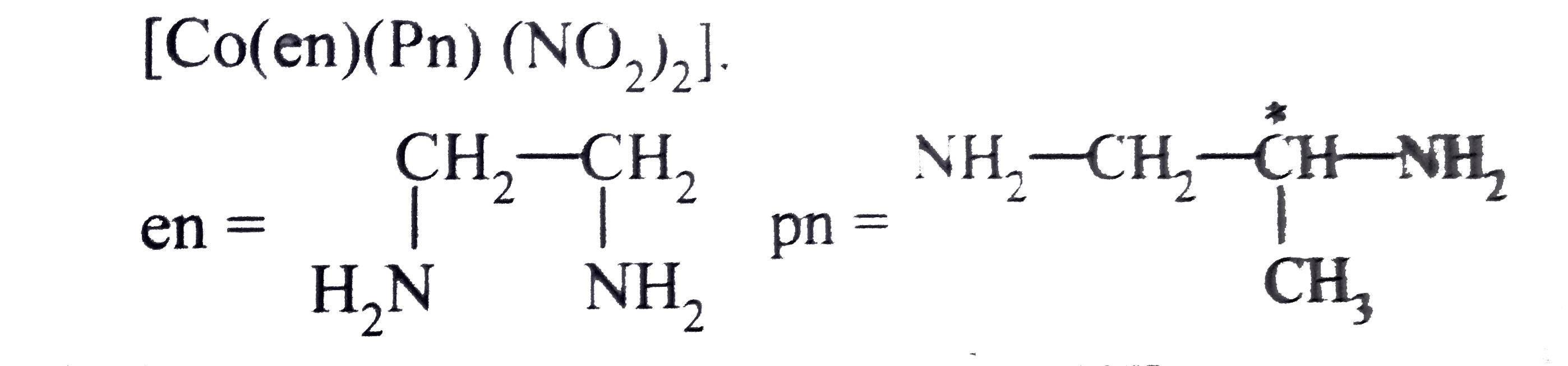 .
.
View SolutionGive the total number of possible isomers of
[Co12(CN)(NH3)en] .
View SolutionThe type of isomerism shown by the complex [CoCl2(en)2] is
View SolutionThe number of geometrical isomers for [Pt(NH3)2CI2] is
View SolutionThe total No. of isomers possible for the complex [Co(en)2Cl2] is :-
View SolutionTotal number of ionisation isomers of [Co(en)2(Br)(Cl)]NO3 are (including the given compound)
View Solution
