एक द्विधात्विक पट्टीका दो पट्टीयों A और B से बनी हैं जिनके रेखीय प्रसार गुणांक क्रमशः αAऔर αB हैI यदि αA<αB हो, तो गर्म करने पर पट्टी :
बाहरी तरफ A के साथ मुड़ेगी
बाहरी तरफ B के साथ मुड़ेगी
बिलकुल नहीं मुड़ेगी
इनमें से कोई भी नहीं
The correct Answer is:B
चूंकि, aB>aA इसलिए, बाहरी तरफ पट्टी B दिखाई देगी
Topper's Solved these Questions
Similar Questions
गतिज ऊर्जा 1 MeV के साथ गतिमान एक पॉजिट्रान और एक इलेक्ट्रॉन दोनों एक दूसरे को नष्ट करके दो गामा फोटॉन उत्सर्जित करते हैं। यदि एक इलेक्ट्रॉन की विराम द्रव्यमान ऊर्जा 0.51 MeV है, तो प्रत्येक फोटॉन की तरंग दैर्ध्य है:
04:17View Solutionएक हाइड्रोजन परमाणु जो प्रारंभ में मूल अवस्था में है, एक फोटॉन को अवशोषित करता है जो इसे n = 4 अवस्था में उत्तेजित करता है। फोटॉन की आवृत्ति है:
03:51View SolutionIf α1,,α2,,αn are the roots of equation xn+nax−b=0, show that (α1−α2)(α1−α2)(α1−αn)=n(α1n−1+a)
06:39View SolutionIf α1,α2,α3,α4 are the roos of x4+2x3+bx2+cx+d=0 such that α1−α3=α4−α2, then b−c is equal to__________
02:02View SolutionIf the roots of equation x3+ax2+b=0areα1,α2,and
α3(a,b≠0). Then find the equation whose roots are
α1α2+α2α3α1α2α3,α2α3+α3α1α1α2α3,α1α3+α1α2α1α2α3.05:04View SolutionA bimetallic is made of two strips A and B having coefficients of linear expansion alpha_(4) and alpha_(B) . If alpha_(A) lt alpha_(B) , then on heating, the strip will
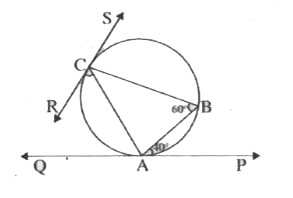
03:34View Solutionआकृति में PQ तथा RS एक वृत्त पर क्रमशः बिन्दु A और C पर स्पर्श रेखाएं है। यदि ∠ABC=60∘ और ∠BAP=40∘ हो तो ∠BCR ज्ञात कीजिए।
 02:16View Solution
02:16View Solutionयदि A और B दो वर्ग आव्यूह इस प्रकार हैं कि AB = A और BA = B तो सिद्ध करो कि A और B समशम होंगे |
04:26View Solutionयदि A और B किसी परीक्षण के प्रतिदर्श समष्टि S कि दो घटनाएँ हो , तो P(SA)=………
02:28View SolutionA bimetalic strip is made of two strips A and B, having co-efficeint of linear expansion as αA and αB. If αA>αB which of the following describes the behavior fo the metallic strip when heated?
05:12View Solutionयदि ω(≠1) इकाई का घनमूल है और (1+ω)7=A+Bω हो, तो A और B क्रमशः होंगे
View Solutionप्रतिबंध 0≤a1,a2,.....an≤π2 तथा (cota1)(cota2).....(cotan)=1, के अन्तर्गत (cosa1)(cosa2)...(cosan) का महत्तम मान है
02:43View SolutionIf the normals drawn to the hyperbola xy = 4 at (αi,βi)(i=1,2,3,4) are concurrent at the point (a, b), then
(α1+α2+α3+α4)(β1+β2+β3+β4)(α1α2α3α4)=07:22View SolutionIf the roots of equation x3+ax2+b=0areα1,α2,and
α3(a,b≠0). Then find the equation whose roots are
α1α2+α2α3α1α2α3,α2α3+α3α1α1α2α3,α1α3+α1α2α1α2α3.07:38View Solutionयदि α1<α2<α3<α4<α5<α6 तो समीकरण (x−α1)(x−α3)(x−α5)+3(x−α2)(x−α4)(x−α6)=0 रखती है-
View Solution

