Which of the following statements about the instantaneous axis (passing through the centre of mass) is correct
The correct Answer is:A
Topper's Solved these Questions
Similar Questions
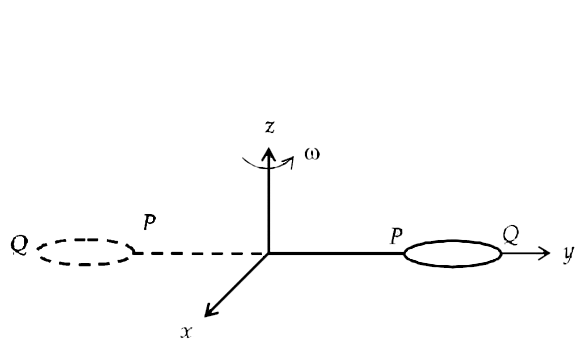
The general motion of a rigid body can be considered to be a combination of (i) a motion of its centre of mass about an axis, and (ii) its motion about an instantaneous exis passing through the centre of mass. These axes need not be stationary. Consider, for example, a thin uniform disc welded (rigidly fixed) horizontally at its rim to a massless, stick as shown in the figure. When the disc-stick system is rotated about the origin on a horizontal frictionless plane with angular speed ω the motion at any instant can be taken as a combination of (i) a rotation of the disc through an instantaneous vertical axis passing through its centre of mass (as is seen from the changed orientation of points P and Q). Both these motions have the same angular speed ω in this case
Now consider two similar system as shown in the figure: Case (a) the disc with its face vertical and parallel to x-z plane, Case (b) the disc with its face making an angle of 45∘ with x-y plane and its horizontal diameter parallel to x-axis. In both the cases, the disc is welded at point P, and the systems are rotated with constant angular speed ω about the z-axis.
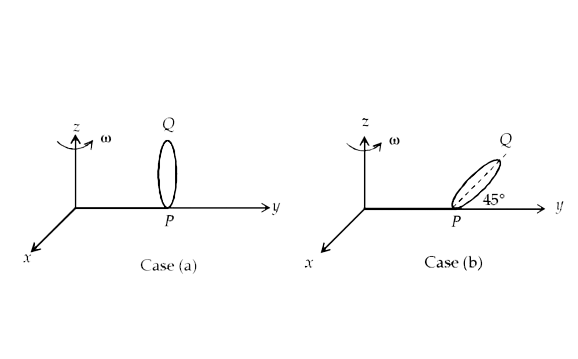
Which of the following statements regarding the angular speed about the instantaneous axis (passing through the centre of mass) is correct?
View SolutionWhich of the following statements are correct for instantaneous axis of rotation?
View Solution
Knowledge Check
The general motion of a rigid body can be considered to be a combination of (i) a motion of its centre of mass about an axis, and (ii) its motion about an instantaneous exis passing through the centre of mass. These axes need not be stationary. Consider, for example, a thin uniform disc welded (rigidly fixed) horizontally at its rim to a massless, stick as shown in the figure. When the disc-stick system is rotated about the origin on a horizontal frictionless plane with angular speed ω the motion at any instant can be taken as a combination of (i) a rotation of the disc through an instantaneous vertical axis passing through its centre of mass (as is seen from the changed orientation of points P and Q). Both these motions have the same angular speed ω in this case

Now consider two similar system as shown in the figure: Case (a) the disc with its face vertical and parallel to x-z plane, Case (b) the disc with its face making an angle of 45∘ with x-y plane and its horizontal diameter parallel to x-axis. In both the cases, the disc is welded at point P, and the systems are rotated with constant angular speed ω about the z-axis.

Which of the following statements regarding the angular speed about the instantaneous axis (passing through the centre of mass) is correct?Ait is √2ω for both the casesBit is ω for cases (a), and ω/√2 for case (b)Cit is ω for case (a), and √2ω for case (b)Dit is ω for both the cases.Which of the following statements are correct for instantaneous axis of rotation?
AAcceleration of every point lying on the axis must be equal to zeroBVelocity of a point distance r from the axis is equal to rωCif moment of inertia of a body about the axis is I and angular velocity is (ω), then kinetic energy of the body is equal to Iω2/2DMoment of inertia of a body is least about nstantaneous axis of rotation among all the parallel axes.Which of the following statement is absolutely correct about mass -
AMore the mass of a body connected with spring balance more will be elongation in spring baanceBMore the mass of body kept in one pan of beam balance more the mass has to be kept on the other pan to keep beam-horizontalCMore the mass of a body, lesser will be its acceleration for a given forceDAll
For the same total mass, which of the following will have the largest moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the body
View SolutionA solid sphere of mass M, radius R and having moment of inertia about as axis passing through the centre of mass as I, is recast into a disc of thickness t, whose moment of inertia about an axis passing through its edge and perpendicular to its plance remains I. Then, radius of the disc will be.
View SolutionFor the same total mass, which of the following will have the largest moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the body
View SolutionFor the same total mass which of the following will have the largest moment of inertia about an axis passing through its centre of mass and perpendicular to the plane of the body.
View SolutionFor the same total mass, which of the following will have the largest moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the body
View Solution
