A block of mass M is attached with a string of mass m and length /as shown in figure. The whole system is placed on a plant whose and radius is three times the mass and radius of earth. Find

Ratio of maximum and minimum velocity of wave pulse (g=acceleration due to gravity on earth).
VmaxVmin=√1+mM
Topper's Solved these Questions
Similar Questions
A block of mass M is attached with a string of mass m and length l as shown in figure. The whole system is placed on a planet whose mass and radius is three times the mass and radius of earth. Find the ratio of maximum and minimum velocity of wave pulse. Assume the acceleration due to gravity on the earth to be g.
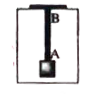
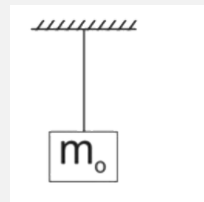
View SolutionThe block of mass m0=μL is attached to a uniform string of mass M=μL and length L as shown in the figure. If a wave pulse is produced near the block, then the time it taken to reach the ceiling is
View Solution
Knowledge Check
Consider a planet whose mass and radius are one-third the mass and radius of the earth. If g is the value of acceleration due to gravity on the earth, then its value on the planet will be ___
A9gB3gC19gD13gIf g1 and g2 denote acceleration due to gravity on the surface of the earth and on a planet whose mass and radius is thrice that of earth, then
Ag1=9g2Bg2=9g1Cg1=3g2Dg2=3g1The binding energy of an object of mass m placed on the surface of the earth r is (R = radius of earth, g = acceleration due to gravity)
AmgR/2Bmg RCmgR4DmgR8
The block of mass m0=μL is attached to a uniform string of mass M=μL and length L as shown in the figure. If a wave pulse is produced near the block, then the time it taken to reach the ceiling is

View SolutionFind the binding energy of a satellite of mass m in orbit of radius r, (R = radius of earth, g = acceleration due to gravity)
View SolutionFind the binding energy of a satellite of mass m in orbit of radius r, (R = radius of earth, g = acceleration due to gravity)
View SolutionWhat will be acceleration due to gravity on a planet whose mass is 4 times and radius is 2 times that of earth ?
View SolutionIf g1 and g2 denote acceleration due to gravity on the surface of the earth and on a planet whose mass and radius is thrice that of earth, then
View Solution
