The magnetic field intensity at the centre of a cubical case of identical wires of length 'a' due to A current I flowing as shown in the figure is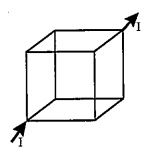
A
(2I/a)
B
√2Ia
C
0
D
I/2a
Text Solution
The correct Answer is:C
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The magnetic field at the origin due to the current flowing in the wire is
 A−μ0I8πa(ˆi+ˆk)Bμ0I2πa(ˆi+ˆk)Cμ0I8πa(−ˆi+ˆk)D−μ0I4πa√2(ˆi−ˆk)
A−μ0I8πa(ˆi+ˆk)Bμ0I2πa(ˆi+ˆk)Cμ0I8πa(−ˆi+ˆk)D−μ0I4πa√2(ˆi−ˆk) - Question 2 - Select One
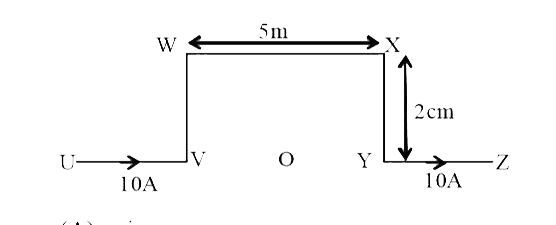
The resulting magnetic field at the point O due to the current carrying wire shown in the figure:
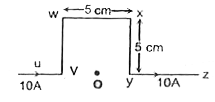 APoints out of the pageBpoints into the pageCis zeroDis the same as due to the segment WX along.
APoints out of the pageBpoints into the pageCis zeroDis the same as due to the segment WX along. - Question 3 - Select One
The magnetic field intensity due to a thin wire carrying current I shown in figure, is
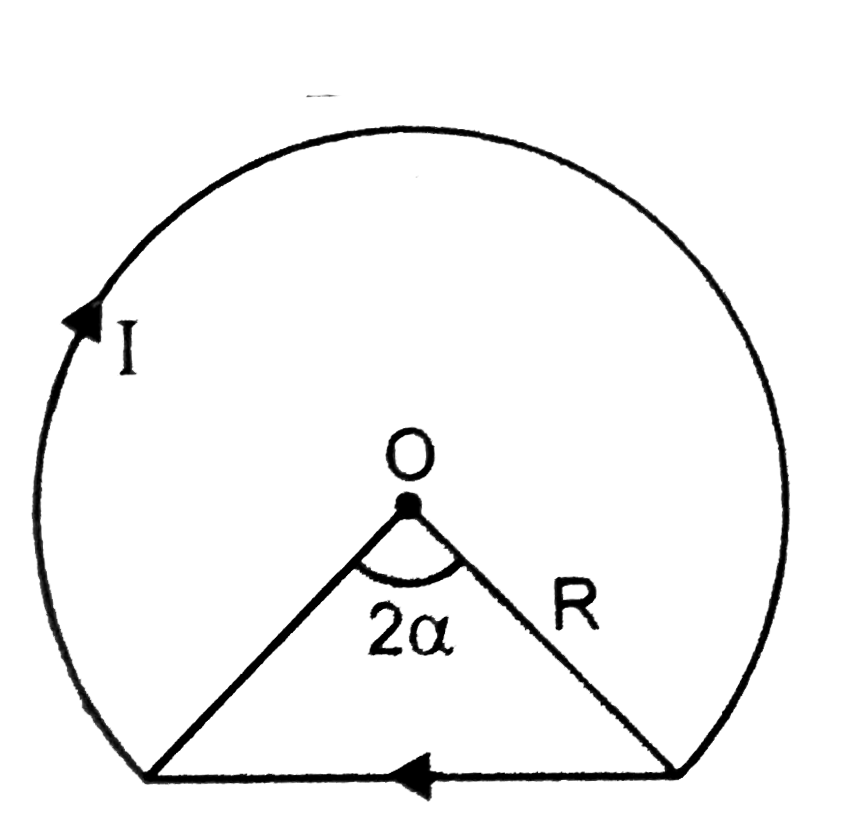 Aμ0I2πR[π−α+tanα]Bμ0I2πR[π−α]Cμ0I2πR[π+α]Dμ0I2πR[π+α−tanα]
Aμ0I2πR[π−α+tanα]Bμ0I2πR[π−α]Cμ0I2πR[π+α]Dμ0I2πR[π+α−tanα]
What is the magnetic field ∣∣→B∣∣ at the point P due to a current carrying wire of length AB (having a current of 2A) shown in figure ?
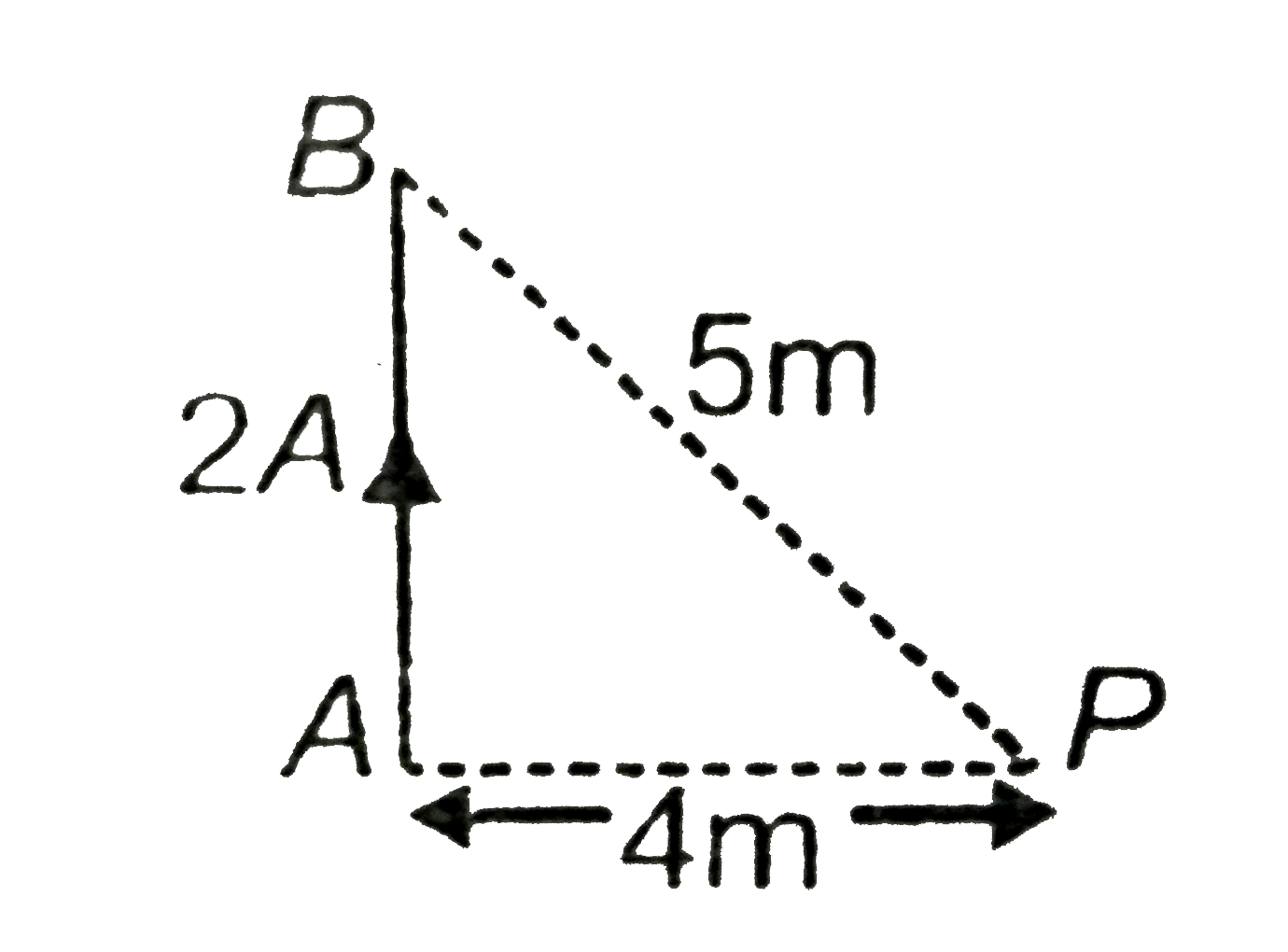 View Solution
View SolutionWhat is the magnetic field ∣∣→B∣∣ at the point P due to a current carrying wire of length AB (having a current of 2A) shown in figure ?
 View Solution
View SolutionThe resulting magnetic field at the point O due to the current carrying wire shown in the figure:
 View Solution
View SolutionThe resulting magnetic field at the point O due to the current carrying wire shown in the figure-
 View Solution
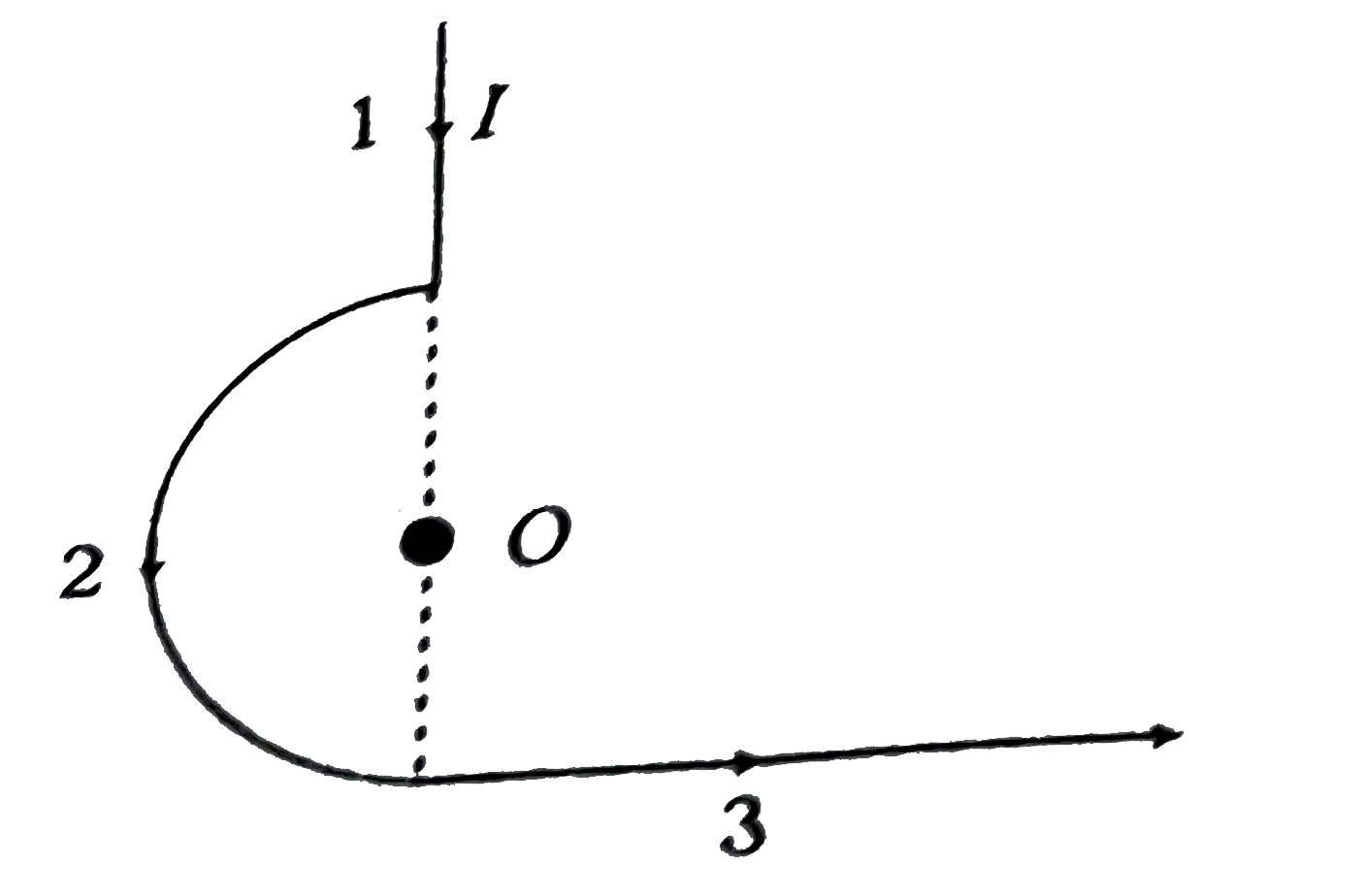
View SolutionMagnetic field produced at the point O due to current flowing in an infinite wire shaped as shown in the figure is
 View Solution
View Solution
