A rigid equilateral triangular plate ABC of side 2m is in motion in the x−y plane. At the instant shown in the figure, the point B has velocity →vB=(3ˆi+8ˆj)ms and the plate has angular velocity →ω=2ˆkrads. Find the speed (in ms ) of point A.
'(##CEN_KSR_PHY_JEE_C07_031_Q20##)'
The correct Answer is:5
Topper's Solved these Questions
Similar Questions
A particle moves in the x−y plane under acceleration →a=3ˆi+4ˆjm/s2. If the initial velocities is →u=6ˆi+8ˆjm/s. Find the velocity and the position vector of a particle after 2s.
View SolutionA particle is moving with velocity ˆi+2ˆj+3ˆk)m/s plane mirror exists in the x-y plane.The mirror has a velocity (ˆi+2ˆj)m/s] of the image (in m/s)
View Solution
Knowledge Check
A particle is moving with a constant acceleration →a=ˆi−2ˆjm/s2 and instantaneous velocity →v=ˆi+2ˆj+2ˆkm/s then rate of change of speed of particle 1 second after this instant will be :
A√5m/s2B√3m/s2C√10m/s2DNone of theseA particle is moving with speed 6m/s along the direction of →A=2ˆi+2ˆj−ˆk, then its velocity is :
A(4ˆi+2ˆj)unitsB(4ˆi+4ˆj−2ˆk) unitsC(4ˆi+4ˆj−4ˆk) unitsD(2ˆi+4ˆj−2ˆk) unitsAn electron is moving with a velocity (2ˆi+2ˆj)m/s is an electric field of intensity →E=ˆi+2ˆj−8ˆkV/m and a magnetic field of →B=(2ˆj+3ˆk) tesla. The magnitude of force on the electron is
A14.4×10−19NB9×10−19NC11.2×10−19ND6.4×10−19N
A particle is moving with a constant acceleration →a=ˆi−2ˆjm/s2 and instantaneous velocity →v=ˆi+2ˆj+2ˆkm/s then rate of change of speed of particle 1 second after this instant will be :
View SolutionIf →a=ˆi+ˆj+2ˆk,→b=2ˆj+ˆkand→c=ˆi+ˆj+ˆk . Find a unit vector perpendicular to the plane containing →a,→b,→c
View SolutionFind the projection of →b+→c on →a where →a=7ˆi−→j+8ˆk, →b= ˆi+2ˆj+3ˆk and →c=ˆj+4ˆk
View SolutionFind the projection of →b+→c on →a where →a=2ˆi− 2ˆj+ ˆk, →b= ˆi+ 2ˆj− 2ˆk and →c=2ˆi− ˆj+ 4ˆk
View SolutionThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s.Its velocity (in m//s) at point B is
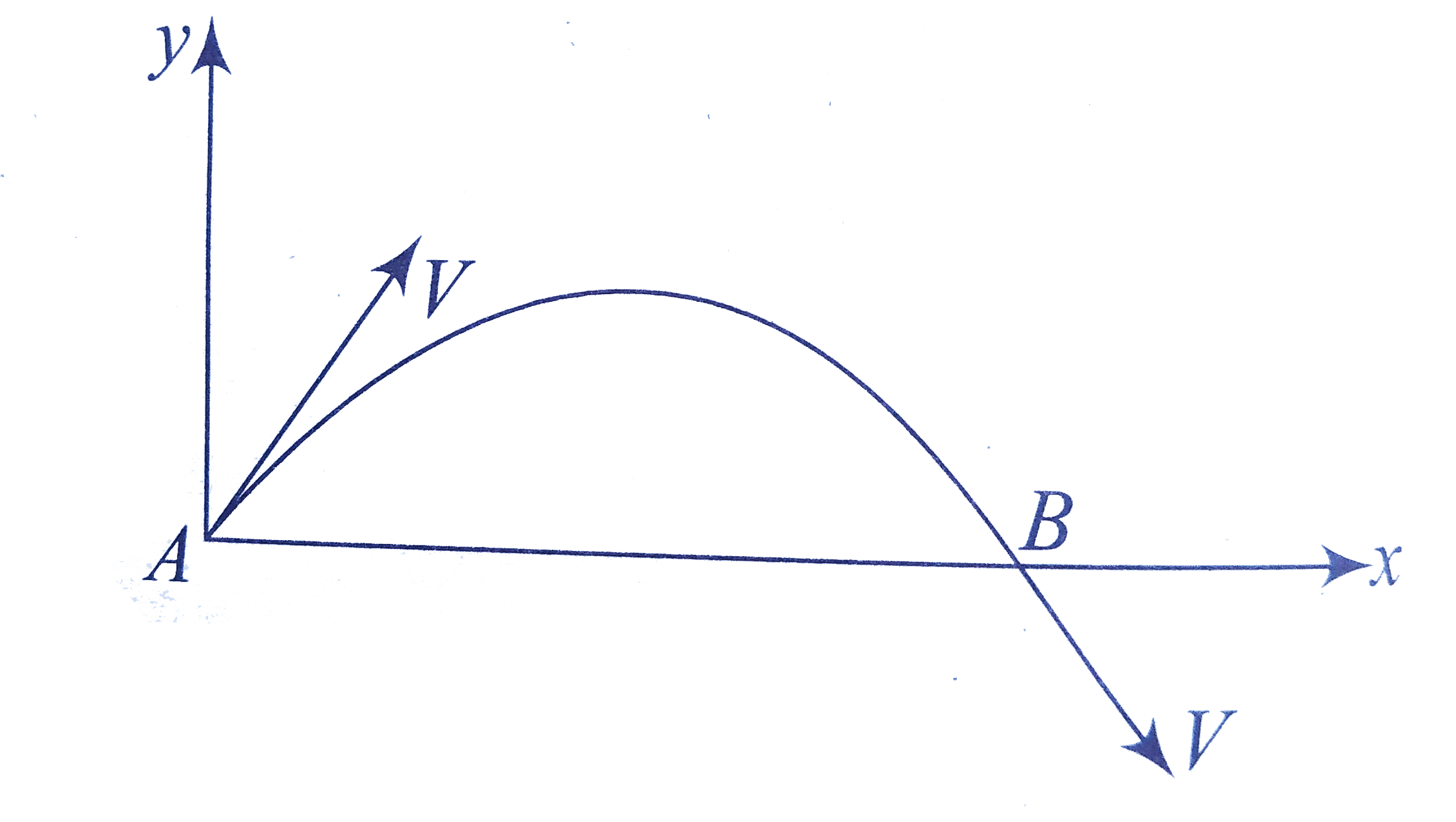
View Solution
