A capacitor is filled with an insulator and a certain potential difference is applied to its pltaes. The energy stored in the capacitor is U. Now the capacitor is disconnected from the source and the insulator is pulled out of the capacitor. The work performed against the forces of electric field in pulling out the insulator is 4U. Then dielectric constant of the insulator is.
The correct Answer is:5
Step by step video, text & image solution for A capacitor is filled with an insulator and a certain potential difference is applied to its pltaes. The energy stored in the capacitor is U. Now the capacitor is disconnected from the source and the insulator is pulled out of the capacitor. The work performed against the forces of electric field in pulling out the insulator is 4 U. Then dielectric constant of the insulator is. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A capacitor is filled with an insulator and a certain potential difference is applied to its pltaes. The energy stored in the capacitor is U. Now the capacitor is disconnected from the source and the insulator is pulled out of the capacitor. The work performed against the forces of electric field in pulling out the insulator is 4U. Then dielectric constant of the insulator is.
View SolutionA capacitor of capacity C0 is charged to certain potential and enrgy stored in it is U0. Now this capacitor is connected in parrallel to uncharged capacitor of capacitance. C. Find the loss of energy.
View Solution
Knowledge Check
A capacitor is charged until its stored energy is 3J and then the charging battery is removed. Now another uncharged capacitor is connected across it and it is found that charge is distributed equally in the two capacitors. The final value of total energy stored in the electric fields is :
A1.5JB3JC2.5JD2JFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dielectric constant K is placed in between the plates of the capacitor as shown.
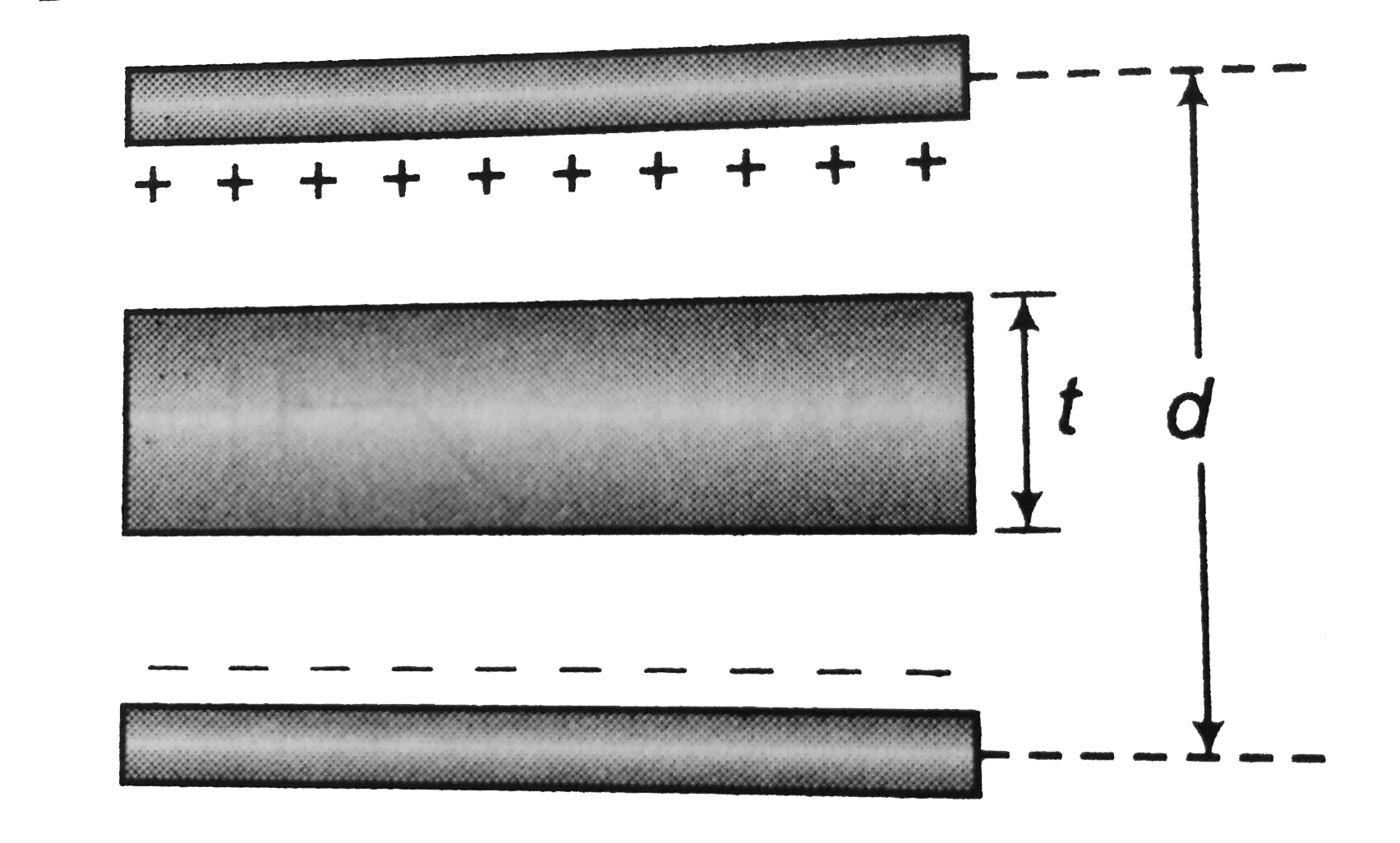
The electric field in the dielectric slab isAVKdBKVdCVdDKVtFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
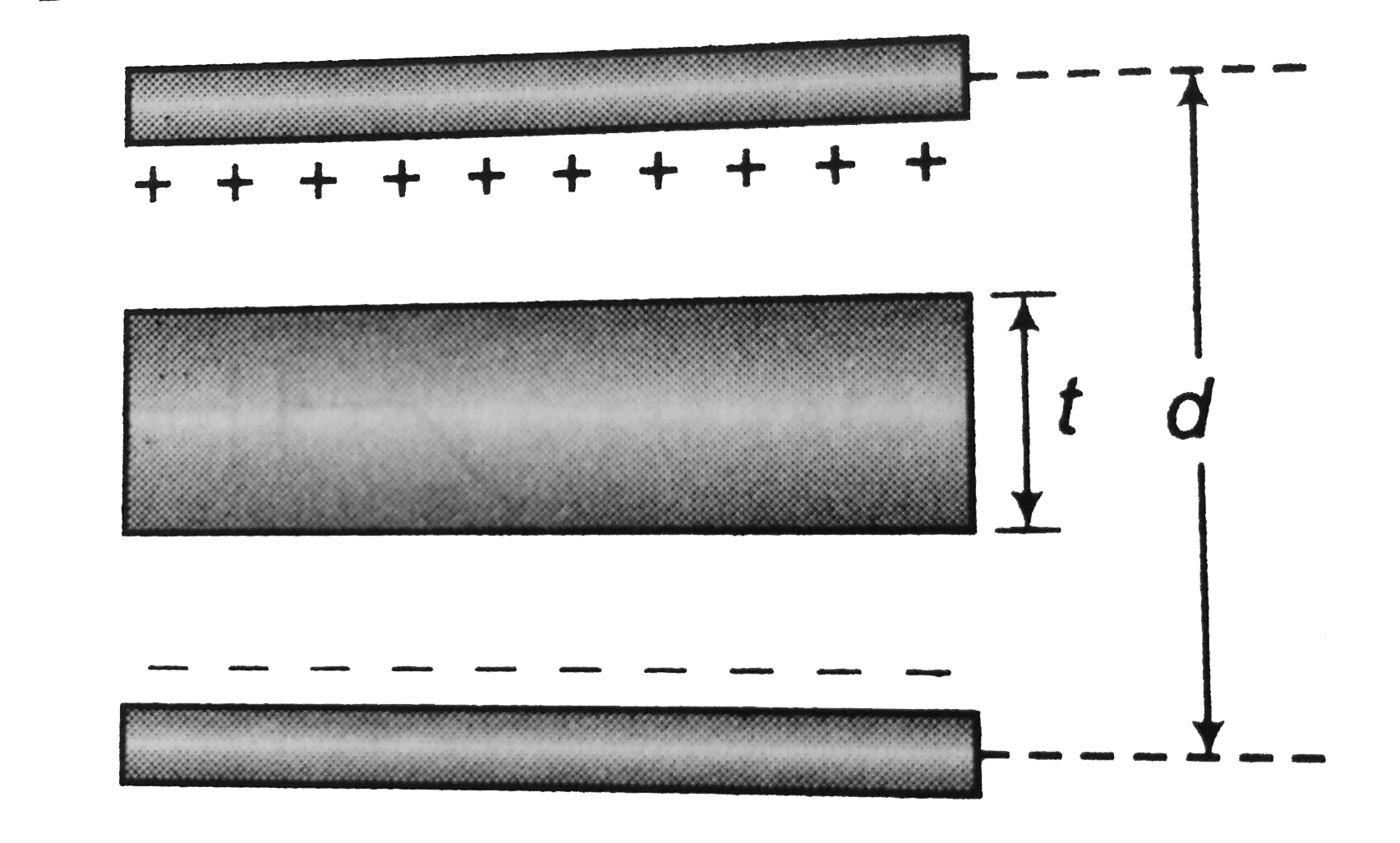
The electric field in the gaps between the plates and the electric slab will beAε0AVdBVdCKVdDVd−t
Three capacitors having capacitances of 8.4μF,8.2μF and 4.21μF are connected in series across a 36 potential difference.
(a) What is the charge on 4.2μF capacitor?
(b) What is the total energy stored in all three capacitors?
(c) The capacitors are disconnected from the potential difference without allowing them to discharge. They are then reconnected in parallel with each other, with the positively charged plates connected together. What is the voltage across each capacitor in the parallel combination?
(d) What is the total energy now stored in the capacitors?
View SolutionThe terminals of a battery of emf V are connected to the two plates of a parallel plate capacitor. If the space between the plates of the capacitor is filled with an insulator of dielectric constant K, then :
View SolutionA capacitor of capacitance C is charged to potential difference V and then disconnected from the battery. The air dielectric of capacitor is replaced by another dielectric of dielectric constant K. The fractional decrease in energy of the capacitor is
View SolutionIdentical dielectric slabs are inserted into two identical capacitors A and B. These capacitors and a battery are connected as shown in figure. Now the slab of capacitor B is pulled out with battery remaining connected
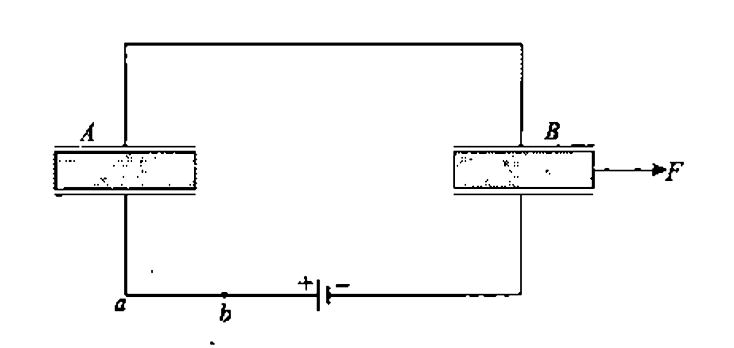
View SolutionA capacitor is charged to store an energy U. The charging battery is disconnected. An Identical capacitor is now connected to the first capacitor in parallel. The energy in each capacitor is now.
View Solution
