निम्न में आप कौन सा सर्वांगसमता प्रतिबंध का प्रयोग करेंगे ?
दिया हैं : AC=DF,AB=DE,BC=EF इसलिए ΔABC≅ΔDEF
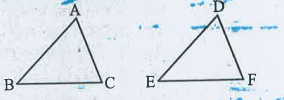
SSS सर्वांगसमता प्रतिबन्ध
SAS सर्वांगसमता प्रतिबन्ध
ASA सर्वांगसमता प्रतिबन्ध
RHS सर्वांगसमता प्रतिबन्ध
The correct Answer is:A
Step by step video & image solution for निम्न में आप कौन सा सर्वांगसमता प्रतिबंध का प्रयोग करेंगे ? दिया हैं : AC=DF , AB=DE, BC=EF इसलिए Delta ABC cong Delta DEF by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
निम्न कथनो को पूरा कीजिये : दो रेखाखण्ड सर्वांगसम होते हैं यदि …………...।
View Solutionदी गयी आकृति में ∠P की माप ज्ञात कीजिये।
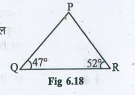
View Solutionएक आयताकार भूखंड की लम्बाई और चौड़ाई क्रमशः 500m तथा 300m हैं। ज्ञात कीजिये:
View SolutionDelta ABC=Delta DEF, Prove that (AB)/(DE)=(AB+BC+AC)/(DE+EF+DF)
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionIn the following figure, AB and BC are equidistant from the centre 'O' of the Show that
(a) ABC is an isosceles triangle.
(b) OB bisects angle ABC.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_X_C13_E02_006_Q01.png" width="80%"gt
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View SolutionIn the given figure, DEF is a triangle. If DF is the longest side and EF is the shortest side, then which of the following is true?
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_E04_028_Q01.png" width="80%"gt
View SolutionFind out area of triangle OAB and BCD shown in figure :- ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/ALN_PHY_R05_E01_014_Q01.png" width="80%"gt
View SolutionIn ΔABCandΔDEF, we have ABDE=BCEF=ACDF=57, then ar(ΔABC):ar(ΔDFR)=?
View SolutionIn two triangle ABC and DEF, AB= DE ,BC=DF and AC =Ef then
View Solution
