वृत्त S1≡x2+y2−4x−6y−12=0 तथा S2≡x2+y2+6x+4y−12=0 व रेखा L=x+y=0 कि
S1 तथा S2 की उभयनिष्ट स्पा रेखार है।
S1 तथा S2 की उभयनिष्ट जीवा है
S1 तथा S2 की मुलाक्ष है
S1 तथा S2 के केंद्र को मिलाने वाली रेखा L के लंबवत है
Step by step video & image solution for वृत्त S_(1)-= x^(2)+y^(2)-4x-6y-12=0" तथा "S_(2)-=x^(2)+y^(2)+6x+4y-12=0 व रेखा L=x+y=0 कि by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ALLEN HINDICHAPTER - वृत्तEXERCISE - Exercise -0223videos
वृत्त
- BOOK - ALLEN HINDICHAPTER - वृत्तEXERCISE - Exercise -0313videos
वृत्त
- BOOK - ALLEN HINDICHAPTER - वृत्तEXERCISE - Exercise -04 [B]8videos
वृत्त
- BOOK - ALLEN HINDICHAPTER - लघुगणक EXERCISE - DO YOURSELF -62videos
लघुगणक
- BOOK - ALLEN HINDICHAPTER - समुच्चयEXERCISE - EXERCISE-I ( CHECK YOUR GRASP)20videos
समुच्चय
Similar Questions
यदि (a,1a),(b,1b),(c,1c)तथा (d,1d)
चार भिन्न - भिन्न बिंदु 4 इकाई त्रिज्या के वृत पर स्थित है तो abcd का मान है09:05View Solutionस्तम्भ-I के चित्रों को स्तम्भ-II के समीकरणों से सुमेलित कीजिये
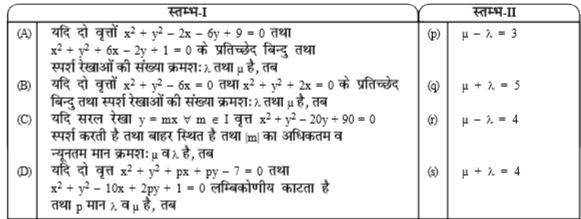 05:32View Solution
05:32View Solutionरेखाओं 3x+ 4y -12 = 0, 5x-12y-20 = 0 तथा 24y -7x-72 = 0 से बने त्रिभुज का अंतःकेंद्र निकालें।
06:04View Solutionहल कीजिए :|x+3|=2(5−x)
04:32View Solutionयदि A और B दो समुच्चय है तो A∩(A∪B) बराबर है
03:11View SolutionConsider the circles
S1:x2+y2−4x−6y−12=0
and S2:x2+y2+6x+18y+26=0
then which of the following is (are) correct?04:34View SolutionThe number of common tangents to the circles x2+y2−4x−6y−12=0 and x2+y2+6x+18y+26=0, is
03:27View Solutionसिद्ध कीजिए कि वृत्त x2+y2=1,x2−2x−4y−11=0 तथा वृत्त x2+y2−4x−6y−243=0 कि त्रिज्याएँ गुणोत्तर श्रेढी में हैं।
01:31View SolutionThe number of common tangents to the circle x2+y2−4x−6y−12=0andx2y2+6x+18y+26=0 is
03:41View SolutionIf the circles x2+y2−6x−8y+12=0,x2+y2−4x+6y+k=0 cut orthogonally, then k =
View Solutionवृतों x2+y2−4x−6y−12=0 तथा x2+y2+6x+18y+26=0 को उभयनिष्ट स्पर्श रेखाओं की संख्या है :
04:34View SolutionThe number of common tangents to the circles x2+y2−4x−6y−12=0 and
x2+y2+6x+18y+26=0, is -07:08View SolutionThe number of common tangents to the circles x2+y2−4x−6y−12=0 and x2+y2+6x+18y+26=0 , is :
07:44View SolutionThe circles x2+y2−6x−8y+12=0,x2+y2−4x+6y+k=0 , cut orthogonally then k=
05:44View SolutionThe circle x2+y2−4x−6y−12=0,x2+y2+6x−8y+21=0 are
06:20View Solution

