The reliability of a COVID PCR test is specified as follows
Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive. From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive.
Based on the above information, answer the following
What is the probability that the ‘person selected will be diagnosed as COVID positive’?
0.1089
0.01089
0.0189
0.189
The correct Answer is:B
Step by step video & image solution for The reliability of a COVID PCR test is specified as follows Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive. From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive. Based on the above information, answer the following What is the probability that the ‘person selected will be diagnosed as COVID positive’? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- QUESTION BANK 2021BOOK - CBSE MODEL PAPERCHAPTER - QUESTION BANK 2021EXERCISE - QUESTIONS
9 Videos
- Additional Practice QuestionsBOOK - CBSE MODEL PAPERCHAPTER - Additional Practice QuestionsEXERCISE - Question
47 Videos
- SAMPLE QUESTION PAPER ( MATHEMATICS )BOOK - CBSE MODEL PAPERCHAPTER - SAMPLE QUESTION PAPER ( MATHEMATICS )EXERCISE - QUESTION
60 Videos
Similar Questions
The reliability of a COVID PCR test is specified as follows
Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive. From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive.
Based on the above information, answer the following
What is the probability that the ‘person is actually not having COVID?
View SolutionThe reliability of a COVID PCR test is specified as follows
Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive. From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive.
Based on the above information, answer the following
What is the probability of the ‘person to be tested as COVID positive’ given that ‘he is actually not having COVID’?
View SolutionThe reliability of a COVID PCR test is specified as follows
Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive. From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive.
Based on the above information, answer the following
What is the probability of the ‘person to be tested as COVID positive’ given that ‘he is actually having COVID?
View SolutionThe reliability of a COVID PCR test is specified as follows
Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive. From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive.

Based on the above information, answer the following
What is the probability that the ‘person is actually having COVID given that ‘he is tested as COVID positive’?
View SolutionSuppose that the reliability of a HIV test is specified as follows: Of people having HIV. 90% of the test detects the disease but 10% go undetected. Of people free of HIV, 99% of the test are judged HIV-ive but 1% are diagnosed as showing HIV+ive. From a large population of which only 0.1% have HIV one person is selected at random, given the HIV test, and the pathologist reports him/her as HIV+ive. What is the probability that the person actually has HIV?
View SolutionA test for detection of a particular disease is not fool proof. The test will correctly detect the disease 90% of the time, but will incorrectly detect the disease 1% of the time. For a large population of which an estimated 0.2% have the disease, a person is selected at random, given the test, and told that he has the disease. What are the chances that the person actually have the disease?
View SolutionIn a group of equal number of men and women 10% men and 45% women are unemployed. What is the probability that a person selected at random is employed ?
View SolutionA can solve 90% of the problems given in a book and B can solve 70%. What is the probability that at least one of them will solve the problem, selected at random from the book?
View SolutionSuppose that 90% of people are right-handed. What is the probability that at most 6 of a random sample of 10 people are right-handed?
View SolutionThe percentage of marks obtained by 10 students in the monthly unit tests are given below:
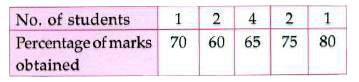
Based on this data, a student is selected at random. Find the probability that the selected student obtains more than 70% marks in a unit test.
View Solution
