For the first row transition metals the Eɵ(M+2/M)) value are:
V−1.18Cr−0.91Mn−1.18Fe−0.44Co−0.28Ni−0.25Cu+0.34
Explain the irregularity in the above values.
The E^(Ɵ)(M^(2+)//M) values are not regular which can be explained from the irregular variation of ionisation enthalpies (ΔiH1+ΔiH2) and also the sublimation enthalpies which are relatively much less for manganese and vanadium.
Topper's Solved these Questions
- THE D AND F-BLOCK ELEMENTS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THE D AND F-BLOCK ELEMENTS EXERCISE - Try Yourself
15 Videos
- THE D AND F-BLOCK ELEMENTS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THE D AND F-BLOCK ELEMENTS EXERCISE - Assingnment(Section -A ( Objective Type Questions)(One option is correct))
50 Videos
- Test-12BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - Test-12EXERCISE - Exercise
58 Videos
- THE P-BLOCK ELEMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THE P-BLOCK ELEMENTSEXERCISE - Assignment Section-J)
16 Videos
Similar Questions
For the first row transition metals the Eɵ value are:
V−1.18Cr−0.91Mn−1.18Fe−0.44Co−0.28Ni−0.25Cu+0.34
Explain the irregularity in the above values.
View Solution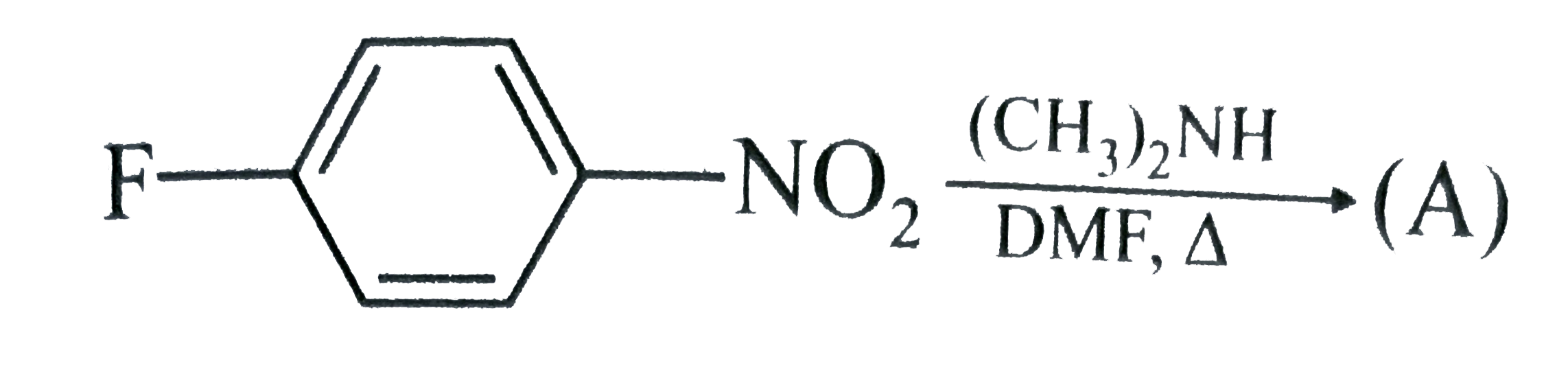 (A) overset(NaNO_(2)//HCl)underset((ii)H_(2)//Ni)underset(0-5^(@))(rarr)(B) , is:
(A) overset(NaNO_(2)//HCl)underset((ii)H_(2)//Ni)underset(0-5^(@))(rarr)(B) , is:
View SolutionEvaluate: π/4∫0sinxdx
View SolutionThe value of cot(23∑n=1cot−1(1+n∑k=12k)) is
View SolutionIf 4∫−1f(x)=4 and 4∫2(3−f(x))dx=7, then the value of −1∫2f(x)dx, is
View SolutionLet a∫0f(x)dx=λ and a∫0f(2a−x)dx=μ. Then,
2a∫0f(x)dx equal to
View SolutionEvaluate: limx→0(1−x)n−1x
View SolutionIf I=1∫011+xπ/2dxthen
View SolutionStudent I,II,andIII perform an experiment for measuring the acceleration due to gravity (g) usinf a simple pendulum. They use lengths of the pendulum and // or record time for different number of oscillations . The observations are shown in the following table . Least count for length =0.1cm
StudentIIIIIILength of Pendulam(cm)64.064.020.0Number ofn Oscillation(n)844TimePeriod(s)16.016.09.0
Least count for time =0.1s.
If EI,EII , and EIII are the percentage errors in g , i.,e., (Δgg×100) for students I,II , and III, respectively , then
View SolutionThe value of 16π/3∫0|sinx|dx is
View Solution
