The work function for Ag metal is 7.5×10−19J. As metal is being exposed to the light of frequency 1220 Å . Which is / are correct statements?
Threshold frequency of metal is 1135×1015s−1
Threshold frequency of metal is 8.33×1015s−1
Stopping potential is 5.46 volt
If light of wavelength 3600 Å is used then photo -electric effect take place
The correct Answer is:1,3
To solve the problem, we need to determine whether the light of frequency corresponding to a wavelength of 1220 Å (angstrom) can cause the photoelectric effect in silver (Ag) metal, given its work function. We will follow these steps:
Step 1: Convert Wavelength to Meters
The wavelength is given in angstroms, and we need to convert it to meters for our calculations.
Wavelength(λ)=1220Å=1220×10−10m
Step 2: Calculate the Energy of the Incident Light
We use the formula for the energy of a photon:
E=hcλ
Where:
- h=6.626×10−34J s (Planck's constant)
- c=3×108m/s (speed of light)
Substituting the values:
E=(6.626×10−34J s)×(3×108m/s)1220×10−10m
Calculating the energy:
E=1.9878×10−251.22×10−7≈1.63×10−18J
Step 3: Compare Energy with Work Function
The work function (ϕ) for silver is given as:
ϕ=7.5×10−19J
Now we compare the energy of the incident light with the work function:
E=1.63×10−18J>ϕ=7.5×10−19J
Since the energy of the incident light is greater than the work function, the photoelectric effect will occur.
Step 4: Calculate Stopping Potential
The stopping potential (V0) can be calculated using the formula:
E−ϕ=eV0
Where e is the charge of the electron (1.6×10−19C).
Substituting the values:
1.63×10−18J−7.5×10−19J=(1.6×10−19C)V0
Calculating the left side:
0.88×10−18J=(1.6×10−19C)V0
Now, solving for V0:
V0=0.88×10−181.6×10−19≈5.5V
Conclusion
1. The photoelectric effect occurs because the energy of the incident light is greater than the work function.
2. The stopping potential is approximately 5.5 V.
The wavelength is given in angstroms, and we need to convert it to meters for our calculations.
We use the formula for the energy of a photon:
- h=6.626×10−34J s (Planck's constant)
- c=3×108m/s (speed of light)
The work function (ϕ) for silver is given as:
The stopping potential (V0) can be calculated using the formula:
1. The photoelectric effect occurs because the energy of the incident light is greater than the work function.
2. The stopping potential is approximately 5.5 V.
Topper's Solved these Questions
- STRUCTURE OF ATOM Book:AAKASH INSTITUTE ENGLISHChapter:STRUCTURE OF ATOM Exercise:ASSIGNMENT ( SECTION -D) Linked Comprehension Type Questions)
Explore 9 Videos
- STRUCTURE OF ATOM Book:AAKASH INSTITUTE ENGLISHChapter:STRUCTURE OF ATOM Exercise:ASSIGNMENT ( SECTION -E) Assertion - Reason Type Questions
Explore 10 Videos
- STRUCTURE OF ATOM Book:AAKASH INSTITUTE ENGLISHChapter:STRUCTURE OF ATOM Exercise:ASSIGNMENT ( SECTION -B) Objective Type Questions (One option is correct)
Explore 38 Videos
- STRUCTURE OF ATOMBook:AAKASH INSTITUTE ENGLISHChapter:STRUCTURE OF ATOMExercise:ASSIGNMENT (SECTION -D) Assertion-Reason Type Questions
Explore 15 Videos
- SURFACE CHEMISTRY Book:AAKASH INSTITUTE ENGLISHChapter:SURFACE CHEMISTRY Exercise:Assignment Section - C (Assertion - Reason type questions)
Explore 10 Videos
Similar Questions
If the work function for a certain metal is 3.2×10−19 joule and it is illuminated with light of frequency 8×1014Hz. The maximum kinetic energy of the photo-electrons would be
(h=6.63×10−34Js)
View SolutionThe work function of a metal is 2.5×10−19J. (a) Find the threshold frequency for photoelectric emission. (b) If the metal is exposed to a light beam of frequency 6.0×1014 Hz, what will be the stopping potential ?
View SolutionA metal surface having a work function ϕ=2.2×10−19J, is illuminated by the light of wavelengh 1320Å. What is the maximum kinetic energy ( in eV ) of the emitted photoelectron ? [Take h=6.6×10−34Js]
View SolutionA metal surface having a work function ϕ=2.2×10−19J, is illuminated by the light of wavelengh 1320Å. What is the maximum kinetic energy ( in eV ) of the emitted photoelectron ? [Take h=6.6×10−34Js]
View SolutionThe work function of a metallic surface is 5.01eV. The photo - electrons are emitted when light of wavelength 2000Å falls on it . The potential difference applied to stop the fastest photo - electrons is [h=4.14×10−15eVsec]
View SolutionThe work function of a certain metal is 3.31×10−19jthen, the maximum kinetic energy of photoelectrons emitted by incident radiation of wavelength 5000Å IS(GIVEN,h=6.62XX10−34J−s,c=3×108ms−1,e=1.6×10−19C)
View SolutionThe work function of a metal is 3.0 V. It is illuminated by a light of wave length 3×10−7m. Calculate i) threshold frequency, ii) the maximum energy of photoelectrons, iii) the stopping potential.
(h=6.63×10−34Jsandc=3×108ms−).
View SolutionWork function of a metal is 3.0 eV. It is illuminated by a light of wavelength 3×10−7 m. Calculate the maximum energy of the electron.
View SolutionThe work function (ϕ0), of a metal X, equals 3×10−19J. Calculate the number (N) of photons, of light of wavelength 26.52nm, whose total energy equal W. Plank's constant =6.63×10−34Js.
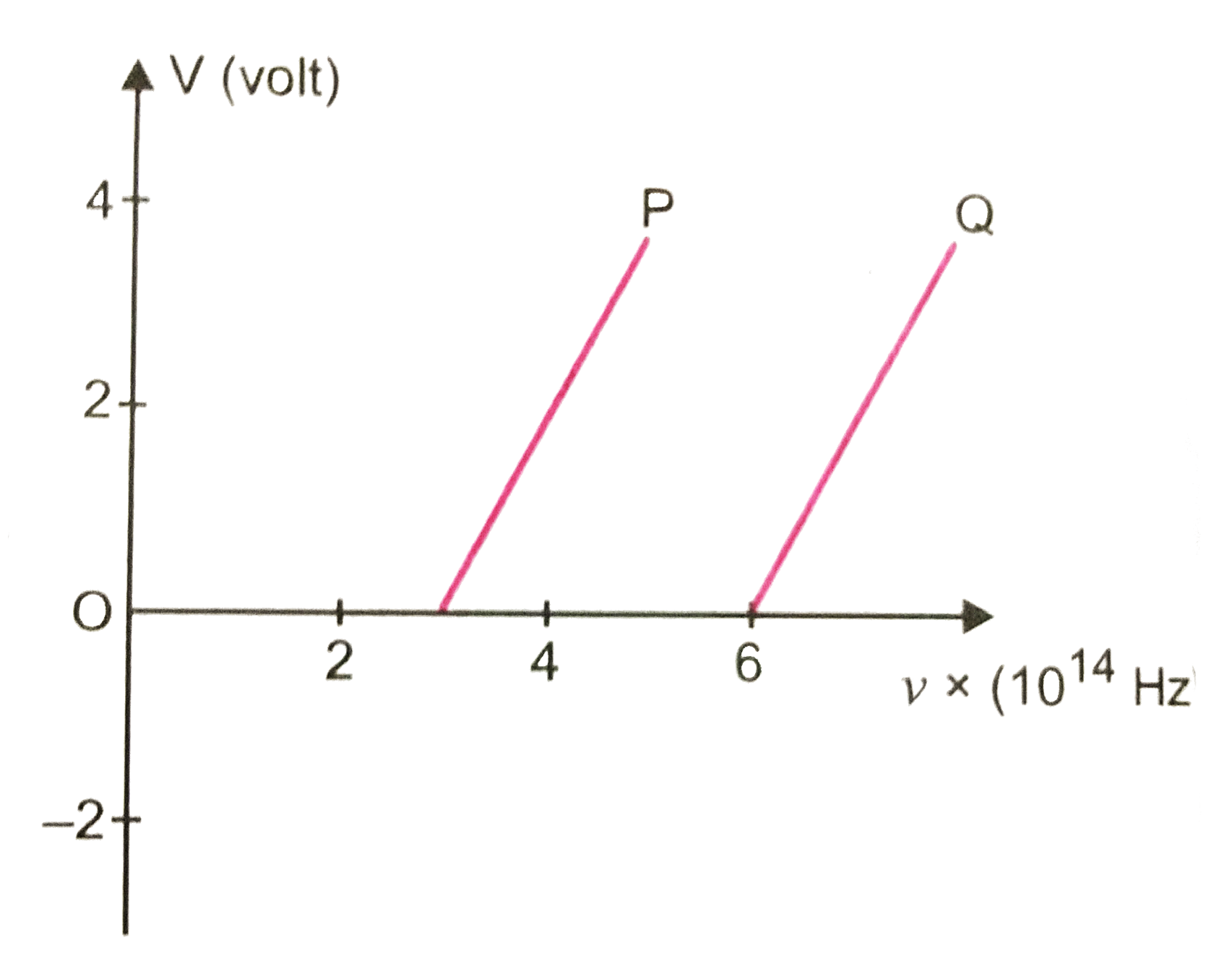
View SolutionIn the study of a photoelectric effect the graph between the stopping potential V and frequency ν of the incident radiation on two different metals P and Q is shown in fig.
(i) which one of two metals have higher threshold frequency
(ii) Determine the work function of the metal which has greater value
(iii) Find the maximum kinetic energy of electron emitted by light of frequency 8×1014Hz for this metal.
View Solution