In photoelectric effect light of certain frequency (> threshold frequency ) is incident on a metal surfave whereby , an e− ( with certain K.E.) moves towards the collector plate and a flow of current is initiated . In order to stop the current flow, an opposite potential , on the two metal plates, is applied
The work function of a metal is 4eV. If light of frequency 2.3×1015Hz is incident on metal surface, then
No photoelectron will be ejected
2 photoelectron of zero kinetic energy are ejected
1 photoelectron of zero kinetic energy is ejected
1 photoelectron is ejected , which required the stopping potential of 5.52 volt
The correct Answer is:4
To solve the problem related to the photoelectric effect, we will follow these steps:
Step 1: Understand the given data
- Work function of the metal (Φ) = 4 eV
- Frequency of the incident light (ν) = 2.3 × 10¹⁵ Hz
- Planck's constant (h) = 6.626 × 10⁻³⁴ J·s
- 1 eV = 1.6 × 10⁻¹⁹ J
Step 2: Calculate the energy of the incident light
The energy of the incident light can be calculated using the formula:
E=hν
Substituting the values:
E=6.626×10−34J⋅s×2.3×1015Hz
Calculating this gives:
E=1.52×10−18J
Step 3: Convert the energy from joules to electron volts
To convert the energy from joules to electron volts, we use the conversion factor:
E(in eV)=E(in J)1.6×10−19J/eV
Calculating this:
E(in eV)=1.52×10−181.6×10−19≈9.5eV
Step 4: Apply the photoelectric equation
The photoelectric equation is given by:
E=Φ+KE
Where:
- KE is the kinetic energy of the emitted electron.
Rearranging gives:
KE=E−Φ
Substituting the values:
KE=9.5eV−4eV=5.5eV
Step 5: Calculate the stopping potential
The stopping potential (Vs) can be calculated using the relationship:
KE=eVs
Where:
- e is the charge of an electron (1 eV corresponds to 1 V for a single electron).
Thus:
Vs=KE
Substituting the value:
Vs=5.5V
Conclusion
From the calculations, we find that:
- One photoelectron is ejected which requires a stopping potential of approximately 5.5 V.
Final Answer
The correct statement is: 1 photoelectron is ejected which requires the stopping potential of 5.52 V.
---
- Work function of the metal (Φ) = 4 eV
- Frequency of the incident light (ν) = 2.3 × 10¹⁵ Hz
- Planck's constant (h) = 6.626 × 10⁻³⁴ J·s
- 1 eV = 1.6 × 10⁻¹⁹ J
The energy of the incident light can be calculated using the formula:
E=hν
E=6.626×10−34J⋅s×2.3×1015Hz
E=1.52×10−18J
To convert the energy from joules to electron volts, we use the conversion factor:
E(in eV)=E(in J)1.6×10−19J/eV
E(in eV)=1.52×10−181.6×10−19≈9.5eV
The photoelectric equation is given by:
E=Φ+KE
Where:
- KE is the kinetic energy of the emitted electron.
KE=E−Φ
KE=9.5eV−4eV=5.5eV
The stopping potential (Vs) can be calculated using the relationship:
KE=eVs
- e is the charge of an electron (1 eV corresponds to 1 V for a single electron).
Vs=KE
Vs=5.5V
From the calculations, we find that:
- One photoelectron is ejected which requires a stopping potential of approximately 5.5 V.
The correct statement is: 1 photoelectron is ejected which requires the stopping potential of 5.52 V.
Topper's Solved these Questions
- STRUCTURE OF ATOM BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - ASSIGNMENT ( SECTION -E) Assertion - Reason Type Questions
10 Videos
- STRUCTURE OF ATOM BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - ASSIGNMENT ( SECTION -F) Matrix - Match Type Questions
4 Videos
- STRUCTURE OF ATOM BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - ASSIGNMENT ( SECTION -C) Objective Type Questions (More than one options are correct)
15 Videos
- STRUCTURE OF ATOMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - ASSIGNMENT (SECTION -D) Assertion-Reason Type Questions
15 Videos
- SURFACE CHEMISTRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SURFACE CHEMISTRY EXERCISE - Assignment Section - C (Assertion - Reason type questions)
10 Videos
Similar Questions
In photoelectric effect light of certain frequency (> threshold frequency ) is incident on a metal surfave whereby , an e− ( with certain K.E.) moves towards the collector plate and a flow of current is initiated . In order to stop the current flow, an opposite potential , on the two metal plates, is applied
The work function of metal is 6eV . If light of frequency 1×1015Hz is incident on the metal , intensity of light is increased 4 times, then
View SolutionIn photoelectric effect light of certain frequency (> threshold frequency ) is incident on a metal surfave whereby , an e− ( with certain K.E.) moves towards the collector plate and a flow of current is initiated . In order to stop the current flow, an opposite potential , on the two metal plates, is applied
A light of frequency 2.5×1015Hz is incident on a metal surface having work function 4 eV. The velocity of photoelectron is ( in cm s−1)
View SolutionIn photoelectric effect, what is meant by the term .threshold frequency.?
View SolutionIn photoelectric effect the slope of stop of stopping potential versus frequency of incident light for a given surface will be
View SolutionGraph between stopping potential and frequency of light as shown find the work function
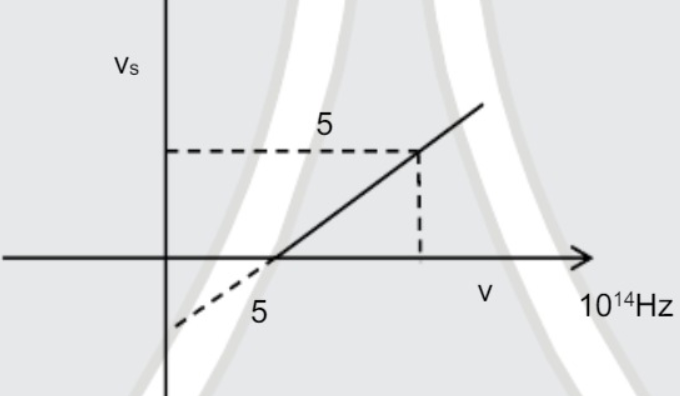
View SolutionThe photoelectric threshold of a certain metal is 3000A. If the radiation of 2000A is incident on the metal
View SolutionThe work function of a metal is hv0. Light of frequency v falls on this metal. The photoelectric effect will take place only if
View SolutionIf the threshold wavelength for a certain metal is 2000Å, then the work function of the metal is
View SolutionGraph between stopping potential and frequency of photon is given in figure
_2019_CBT7_E01_021_Q01.png)
Find out work function of metal:
View SolutionThe photoelectric threshold frequency of a metal is v. When light of frequency 4v is incident on the metal . The maximum kinetic energy of the emitted photoelectrons is
View Solution
