Two trains each of length 100 m are moving with speeds 54 km/h (for Train - 1) and 90 km/h (for Train-2) on two parallel tracks. Match the entries given in column I with the entries given in column II
Column I Column II(A) Time taken in passing (in s)(p) 125(B) Distance travelled by 1st train during passing (in m)(q) 500(C) Distance travelled by 2nd train during passing (in m)(r) 20 if both trains are moving in same direction(D) Displacement of 1st train w.r.t. 2nd train in 2 s (in m)(s) 300(t) 5
The correct Answer is:A(r, t), B(s), C(q), D(r)
To solve the problem of matching the entries in Column I with those in Column II, we will follow these steps:
Step 1: Convert the speeds from km/h to m/s
To convert the speeds of the trains from kilometers per hour to meters per second, we use the conversion factor 518.
- For Train 1 (speed = 54 km/h):
Speed of Train 1=54×518=15 m/s
- For Train 2 (speed = 90 km/h):
Speed of Train 2=90×518=25 m/s
Step 2: Calculate the time taken for the trains to pass each other when moving in the same direction
When two objects are moving in the same direction, the relative velocity is the difference of their speeds.
- Relative velocity Vr when moving in the same direction:
Vr=25−15=10 m/s
- The distance to be covered when passing each other is the sum of their lengths:
Distance=100+100=200 m
- Time taken to pass each other:
Time=DistanceVr=20010=20 seconds
Step 3: Calculate the time taken for the trains to pass each other when moving in opposite directions
When moving in opposite directions, the relative velocity is the sum of their speeds.
- Relative velocity Vr when moving in opposite directions:
Vr=25+15=40 m/s
- Time taken to pass each other:
Time=DistanceVr=20040=5 seconds
Step 4: Calculate the distance traveled by Train 1 during passing
When the trains are moving in the same direction:
- Time = 20 seconds, Speed of Train 1 = 15 m/s
Distance=Speed×Time=15×20=300 m
When the trains are moving in opposite directions:
- Time = 5 seconds, Speed of Train 1 = 15 m/s
Distance=15×5=75 m
Step 5: Calculate the distance traveled by Train 2 during passing
When the trains are moving in the same direction:
- Time = 20 seconds, Speed of Train 2 = 25 m/s
Distance=25×20=500 m
When the trains are moving in opposite directions:
- Time = 5 seconds, Speed of Train 2 = 25 m/s
Distance=25×5=125 m
Step 6: Calculate the displacement of Train 1 with respect to Train 2 in 2 seconds
When moving in the same direction:
- Relative velocity Vr=10 m/s
Displacement=Vr×Time=10×2=20 m
When moving in opposite directions:
- Relative velocity Vr=40 m/s
Displacement=Vr×Time=40×2=80 m
Final Matching of Columns
Now we can match the results with Column II:
- (A) Time taken in passing (in s) → (p) 20 seconds (same direction) and (t) 5 seconds (opposite direction)
- (B) Distance traveled by 1st train during passing (in m) → (s) 300 m (same direction) and (r) 75 m (opposite direction)
- (C) Distance traveled by 2nd train during passing (in m) → (q) 500 m (same direction) and (p) 125 m (opposite direction)
- (D) Displacement of 1st train w.r.t. 2nd train in 2 s (in m) → (r) 20 m (same direction) and (s) 80 m (opposite direction)
To convert the speeds of the trains from kilometers per hour to meters per second, we use the conversion factor 518.
Speed of Train 1=54×518=15 m/s
Speed of Train 2=90×518=25 m/s
When two objects are moving in the same direction, the relative velocity is the difference of their speeds.
Vr=25−15=10 m/s
Distance=100+100=200 m
Time=DistanceVr=20010=20 seconds
When moving in opposite directions, the relative velocity is the sum of their speeds.
Vr=25+15=40 m/s
Time=DistanceVr=20040=5 seconds
When the trains are moving in the same direction:
- Time = 20 seconds, Speed of Train 1 = 15 m/s
Distance=Speed×Time=15×20=300 m
- Time = 5 seconds, Speed of Train 1 = 15 m/s
Distance=15×5=75 m
When the trains are moving in the same direction:
- Time = 20 seconds, Speed of Train 2 = 25 m/s
Distance=25×20=500 m
- Time = 5 seconds, Speed of Train 2 = 25 m/s
Distance=25×5=125 m
When moving in the same direction:
- Relative velocity Vr=10 m/s
Displacement=Vr×Time=10×2=20 m
- Relative velocity Vr=40 m/s
Displacement=Vr×Time=40×2=80 m
Now we can match the results with Column II:
- (A) Time taken in passing (in s) → (p) 20 seconds (same direction) and (t) 5 seconds (opposite direction)
- (B) Distance traveled by 1st train during passing (in m) → (s) 300 m (same direction) and (r) 75 m (opposite direction)
- (C) Distance traveled by 2nd train during passing (in m) → (q) 500 m (same direction) and (p) 125 m (opposite direction)
- (D) Displacement of 1st train w.r.t. 2nd train in 2 s (in m) → (r) 20 m (same direction) and (s) 80 m (opposite direction)
Topper's Solved these Questions
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - G)
4 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - H)
9 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - E)
8 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
Similar Questions
Match the entries in column II with the statements given in column I.

View SolutionMatch all the entries in column-I to all the entries in column-II
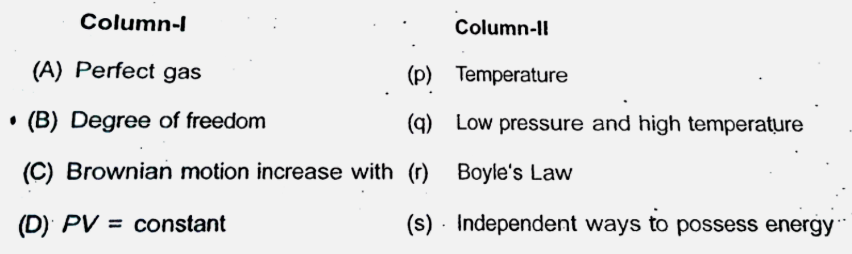
View SolutionMatch the entry in colum-I with the entry in column-II
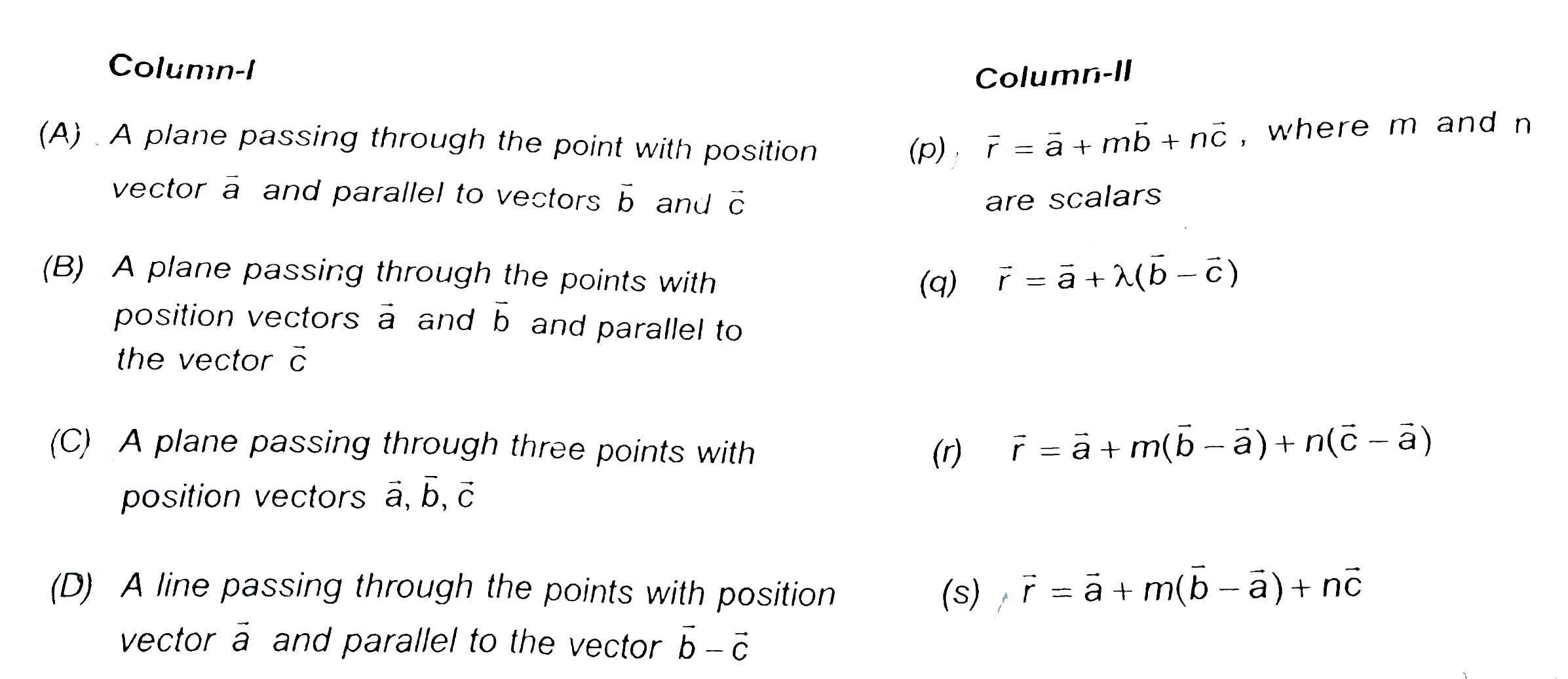
View SolutionMatch the entries of Column I with those of Column-II

View SolutionMatch the entries of Column I with those of Column-II

View SolutionMatch the entries in column I and column II with same dimensional formula

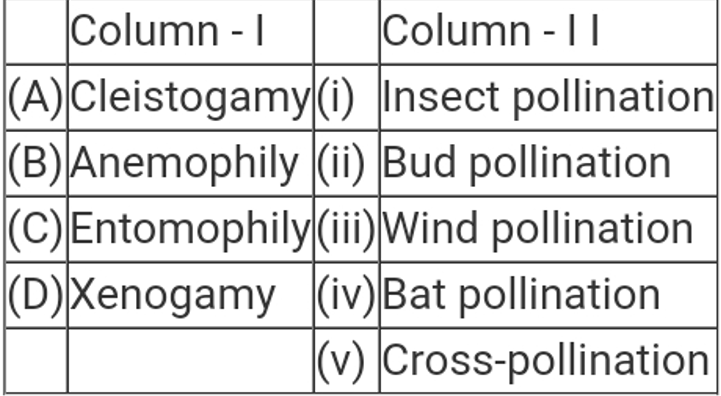
View SolutionMatch the entries in Column - I with those of Column - II and chosse the correct answer
View SolutionRegarding the spectrum of hydrogen, match the entries in column-I with all the entries in column-II. Match the following :
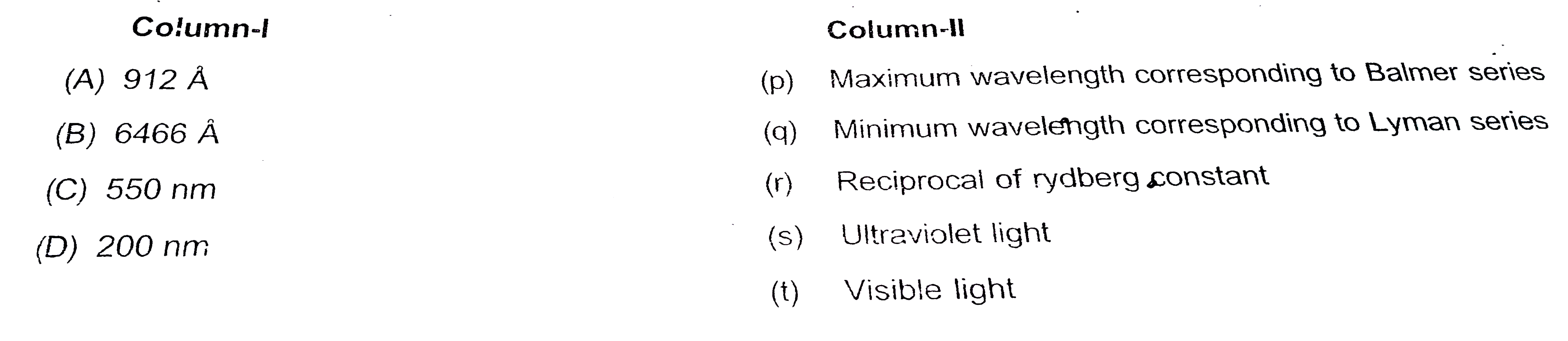
View SolutionTwo trains each of length 100 m moving parallel towards each other at speed 72km/h and 36km/h respectively. In how much time will they cross each other?
View SolutionThe correct match between the entries in column I and column II are :
View Solution
