A listener is at rest and a police siren is moving away from the listerner at 60m/s. what frequency does the listerner hear given that the velocity of sound in air is 340m/s and frequency os siren is 500Hz?
To solve the problem of finding the frequency heard by a listener when a police siren is moving away, we will use the Doppler effect formula. Here’s a step-by-step solution:
Step 1: Understand the Doppler Effect Formula
The Doppler effect formula for sound is given by:
f′=f(v+vov−vs)
Where:
- f′ = apparent frequency heard by the observer
- f = actual frequency of the source (siren)
- v = speed of sound in air
- vo = speed of the observer (listener)
- vs = speed of the source (siren)
Step 2: Identify the Given Values
From the problem, we have:
- f=500Hz (frequency of the siren)
- v=340m/s (speed of sound in air)
- vo=0m/s (the listener is at rest)
- vs=60m/s (the siren is moving away from the listener)
Step 3: Substitute the Values into the Formula
Since the listener is at rest, vo=0. The siren is moving away, so we will use a plus sign for vs:
f′=500(340+0340−60)
Step 4: Simplify the Equation
Now, simplify the equation:
f′=500(340280)
Step 5: Calculate the Apparent Frequency
Now, calculate the value:
f′=500×340280
Calculating 340280:
340280=1714≈1.2143
Now multiply by 500:
f′≈500×1.2143≈607.15Hz
Step 6: Final Result
The frequency that the listener hears is approximately:
f′≈607.15Hz
The Doppler effect formula for sound is given by:
- f′ = apparent frequency heard by the observer
- f = actual frequency of the source (siren)
- v = speed of sound in air
- vo = speed of the observer (listener)
- vs = speed of the source (siren)
From the problem, we have:
- f=500Hz (frequency of the siren)
- v=340m/s (speed of sound in air)
- vo=0m/s (the listener is at rest)
- vs=60m/s (the siren is moving away from the listener)
Since the listener is at rest, vo=0. The siren is moving away, so we will use a plus sign for vs:
Now, simplify the equation:
Now, calculate the value:
The frequency that the listener hears is approximately:
Topper's Solved these Questions
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Try Yourself
65 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-A)
55 Videos
- WAVE OPTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))
1 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - D)
13 Videos
Similar Questions
If a listener L is at rest and the siren in previous illustrantion is moving away from L at a speed of 30ms, what frequency does the listener hear?
View SolutionWhat is the fundamental frequency of an open organ pipe of length 42.5 cm, if the velocity of sound in air is 340 m/s?
View SolutionA stationary sound sound 's' of frequency 334 Hz and a stationary ovserver 'O' are placed near a reflecting suface moving away from the source with velocity 2 m/s as shown in the figure. If the velocity of the sound waves is air is v= 330 m/s the apparent frequency of the echo is
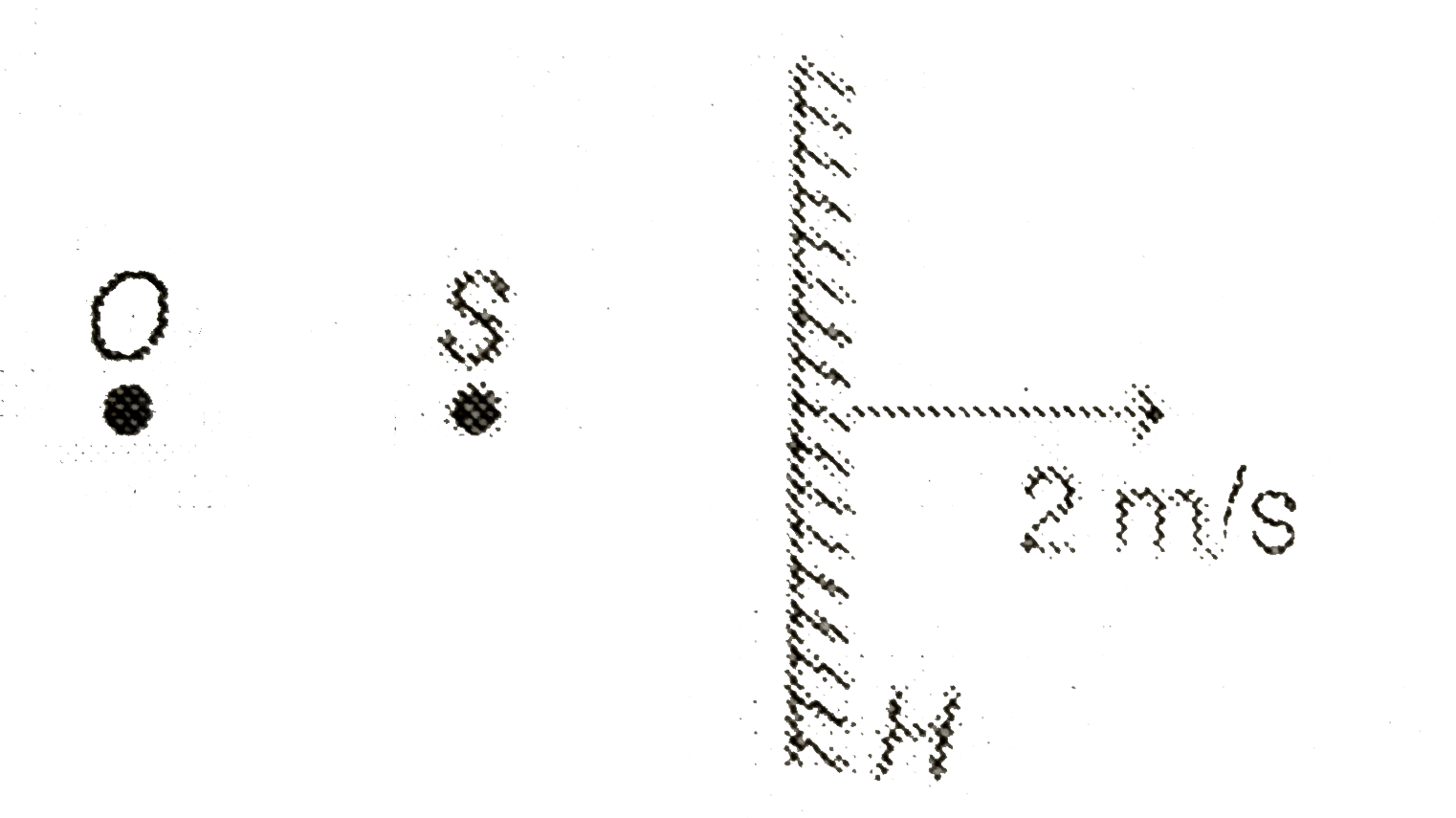
View SolutionA siren emitting sound of frequency 800Hz is going away from a static listener with a speed of 30 m/s. Frequency of the sound to be heard by the listener is ( Take velocity of sound as 300 m/s )
View SolutionA plice siren emits a sound wave of frequency 440 Hz. The speed of sound is 332 m/s. if the siren is moving away from the listerner with a speed of 18 m/s relative to the air and the listerner is moving towards the siren with a speed of 68 m/s relative to the air, then what frequency does the listern hear?
View SolutionFind out frequency at which maximum intensity detected by observer 0. If velocity of sound =340m/s−1 and frequency range of source 2000 Hz to 5000 Hz
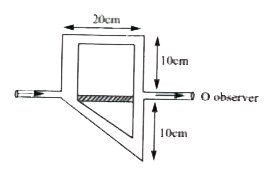
View SolutionA police car with a siren of frequency 8KHz is moving with uniform velocity 36Km/hr towards a ball building which reflects the sound waves. The speed of sound in air is 320m/s. The frequency of the siren heard by the car driver is
View SolutionA police car with a siren of frequency 8KHz is moving with uniform velocity 36Km/hr towards a ball building which reflects the sound waves. The speed of sound in air is 320m/s. The frequency of the siren heard by the car driver is
View SolutionCalculate the frequency of 2nd harmonic in an open organ pipe of length 34 cm if the velocity of sound is 340m/s.
View SolutionA man standing on a platform observes that the frequency of the sound of a whistle emitted by a train drops by 140 Hz. If the velocity of sound in air is 330 m/s and the speed of the train is 70 m/s, the frequency of the whistle is
View Solution
