The equation of a transverse wave travelling along a string is y(xt)=0.3sin(200x−0.5t)
what is the equation of the reflected wave when it is reflected by
(i) A rigid boundary?
(ii) A free end?
To solve the problem, we need to analyze the given wave equation and determine how it changes when reflected from different types of boundaries.
The original wave equation is given as:
y(x,t)=0.3sin(200x−0.5t)
Step 1: Identify the properties of the wave
The wave travels in the positive x-direction with:
- Amplitude A=0.3
- Wave number k=200
- Angular frequency ω=0.5
Step 2: Reflection at a Rigid Boundary
When a wave reflects off a rigid boundary:
1. The wave direction reverses (from positive x-direction to negative x-direction).
2. There is a phase change of π (or 180 degrees).
The reflected wave equation will have the form:
y(x,t)=Asin(kx+ωt+π)
Substituting the values:
- Amplitude remains 0.3
- Wave number remains 200
- Angular frequency remains 0.5
Thus, the equation becomes:
y(x,t)=0.3sin(200x+0.5t+π)
Using the property of sine, sin(θ+π)=−sin(θ), we can simplify this to:
y(x,t)=−0.3sin(200x+0.5t)
Step 3: Reflection at a Free End
When a wave reflects off a free end:
1. The wave direction also reverses (from positive x-direction to negative x-direction).
2. There is no phase change.
The reflected wave equation will have the form:
y(x,t)=Asin(kx+ωt)
Substituting the values:
- Amplitude remains 0.3
- Wave number remains 200
- Angular frequency remains 0.5
Thus, the equation becomes:
y(x,t)=0.3sin(200x+0.5t)
Final Answers
(i) The equation of the reflected wave at a rigid boundary is:
y(x,t)=−0.3sin(200x+0.5t)
(ii) The equation of the reflected wave at a free end is:
y(x,t)=0.3sin(200x+0.5t)
y(x,t)=0.3sin(200x−0.5t)
The wave travels in the positive x-direction with:
- Amplitude A=0.3
- Wave number k=200
- Angular frequency ω=0.5
When a wave reflects off a rigid boundary:
1. The wave direction reverses (from positive x-direction to negative x-direction).
2. There is a phase change of π (or 180 degrees).
y(x,t)=Asin(kx+ωt+π)
- Amplitude remains 0.3
- Wave number remains 200
- Angular frequency remains 0.5
y(x,t)=0.3sin(200x+0.5t+π)
y(x,t)=−0.3sin(200x+0.5t)
When a wave reflects off a free end:
1. The wave direction also reverses (from positive x-direction to negative x-direction).
2. There is no phase change.
y(x,t)=Asin(kx+ωt)
- Amplitude remains 0.3
- Wave number remains 200
- Angular frequency remains 0.5
y(x,t)=0.3sin(200x+0.5t)
(i) The equation of the reflected wave at a rigid boundary is:
y(x,t)=−0.3sin(200x+0.5t)
y(x,t)=0.3sin(200x+0.5t)
Topper's Solved these Questions
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-A)
55 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-B)
35 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
- WAVE OPTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))
1 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - D)
13 Videos
Similar Questions
Knowledge Check
The equation of a wave travelling in a string can be written as y=3cosπ(10t−x). Its wavelength is
A100 cmB2 cmC5 cmDnone of the above
The equation of a travelling wave on a string is y = (0.10 mm )sin[31.4m−1x+(314s−1)t]
View SolutionWhich of the following equations represent a transverse wave travelling along- y axis ?
View SolutionThe equation of a transverse wave travelling along a string is given by
y=0.4sin[π(0.5x−200t)] where y and x are measured in cm and t in seconds. Suppose that you clamp the string at two points L cm apart and you observe a standing wave of the same wavelength as above transverse wave. For what values of L less than 10cm is this possible?
View SolutionThe equation of a transverse wave travelling along x-axis is given by y = 10 sin π= (0.01 x- 2.0t) where y and x are represented in centimeters and, in seconds. Find the amplitude, frequency velocity and wavelength of the wave.
View Solutionthe equation of a wave travelling along the positive x - axis ,as shown in figure at t = 0 is given by
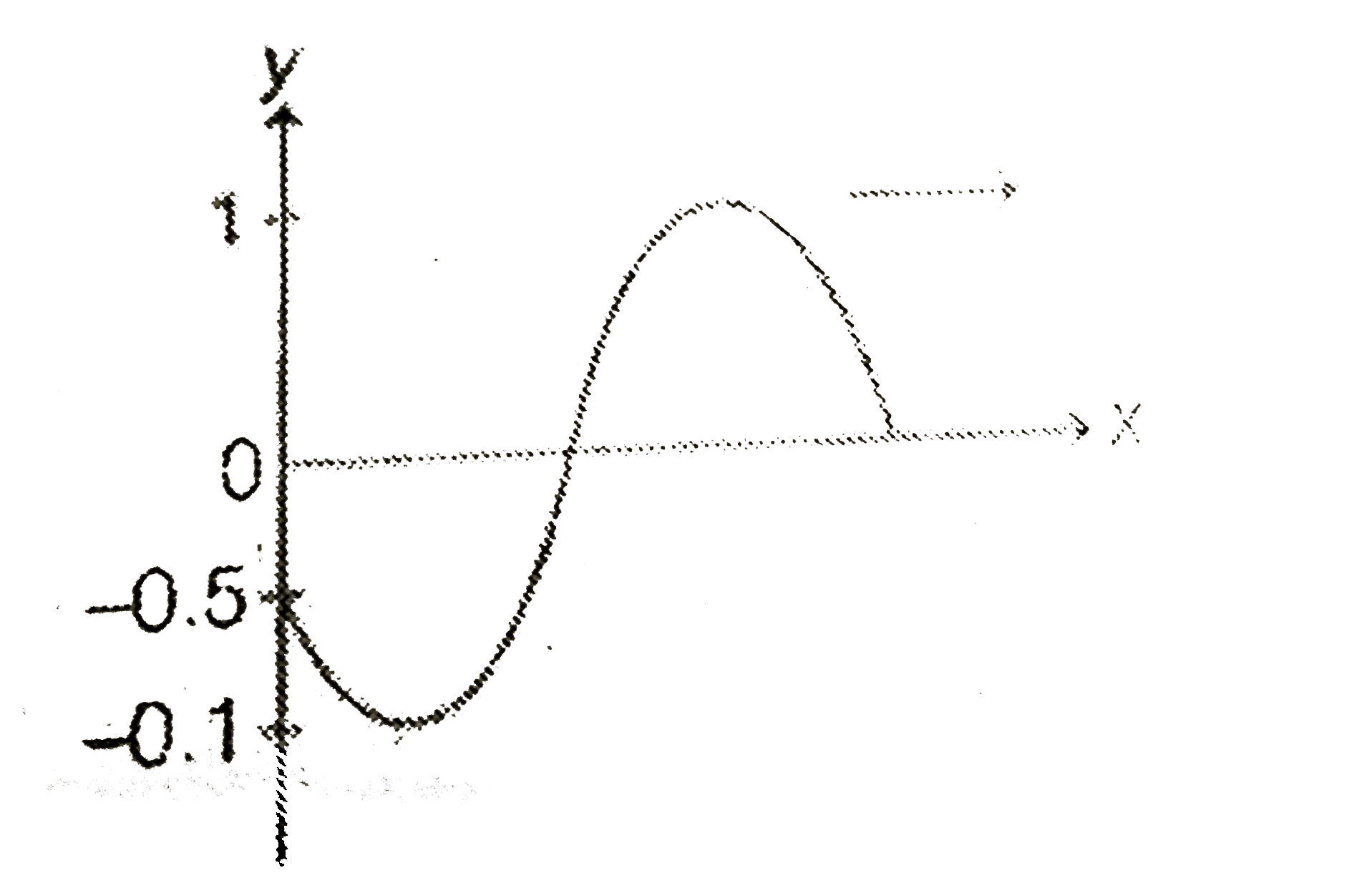
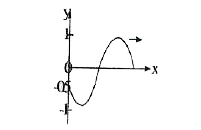
View SolutionThe equation of a wave travelling along the positive x-axis, as shown in figure at t = 0 is given by
View SolutionA transverse wave travelling on a taut string is represented by:
Y=0.01sin2π(10t−x)
Y and x are in meters and t in seconds. Then,
View Solution
