If your height is h and you want to see your complete image in a plane mirror, what should be the minimum size of the mirror ?
Strategy : You can see your complete image if light rays from the top of your head and light rays from your feet reach your eyes after reflection.
To determine the minimum size of a plane mirror required for a person of height h to see their complete image, we can follow these steps:
1. Understanding the Reflection:
- A plane mirror reflects light such that the angle of incidence equals the angle of reflection. This means that the rays coming from the top of the head and the feet of the person must reach the eyes after reflecting off the mirror.
2. Drawing the Diagram:
- Imagine a person standing in front of a plane mirror. Let’s denote the height of the person as h. The top of the head is at height h and the feet are at height 0.
3. Identifying Eye Level:
- Let’s assume the eye level of the person is at a height x (where 0<x<h).
4. Ray Diagram:
- Draw two rays: one from the top of the head to the mirror and then to the eyes, and another from the feet to the mirror and then to the eyes.
- The ray from the head will reflect downwards and the ray from the feet will reflect upwards.
5. Calculating the Required Mirror Height:
- For the person to see their entire height in the mirror, the mirror must be tall enough to reflect both rays to the eyes.
- The height of the mirror needs to cover half the distance from the eyes to the top of the head and half the distance from the eyes to the feet.
- The distance from the eyes to the top of the head is h−x and the distance from the eyes to the feet is x.
6. Minimum Height of the Mirror:
- The minimum height of the mirror H can be calculated as:
H=(h−x)+x2=h2
- Therefore, the minimum size of the mirror required is h2.
Conclusion:
The minimum size of the mirror required for a person of height h to see their complete image is h2.
- A plane mirror reflects light such that the angle of incidence equals the angle of reflection. This means that the rays coming from the top of the head and the feet of the person must reach the eyes after reflecting off the mirror.
- Imagine a person standing in front of a plane mirror. Let’s denote the height of the person as h. The top of the head is at height h and the feet are at height 0.
- Let’s assume the eye level of the person is at a height x (where 0<x<h).
- Draw two rays: one from the top of the head to the mirror and then to the eyes, and another from the feet to the mirror and then to the eyes.
- The ray from the head will reflect downwards and the ray from the feet will reflect upwards.
- For the person to see their entire height in the mirror, the mirror must be tall enough to reflect both rays to the eyes.
- The height of the mirror needs to cover half the distance from the eyes to the top of the head and half the distance from the eyes to the feet.
- The distance from the eyes to the top of the head is h−x and the distance from the eyes to the feet is x.
- The minimum height of the mirror H can be calculated as:
H=(h−x)+x2=h2
- Therefore, the minimum size of the mirror required is h2.
The minimum size of the mirror required for a person of height h to see their complete image is h2.
Similar Questions
You are standing at a distance 2 metre from a plane mirror.
What is the distance of your image from the mirror ?
View SolutionA man of height 170cmwants to see his complete image in a plane mirror(while standing).His eyes are at a height of 160cmfrom the ground.
View SolutionYou are standing at a distance 2 metre from a plane mirror.
What is the distance between you and your image ?
View SolutionIn the figure, you can see a light ray travelling from air to glass. Why is there no refraction?

View SolutionA person wants to see AB part of his image. His eye level is at 1.8m above the ground. If he uses minimum size of mirror required for this, find the height of the lowest point of mirror above the ground.
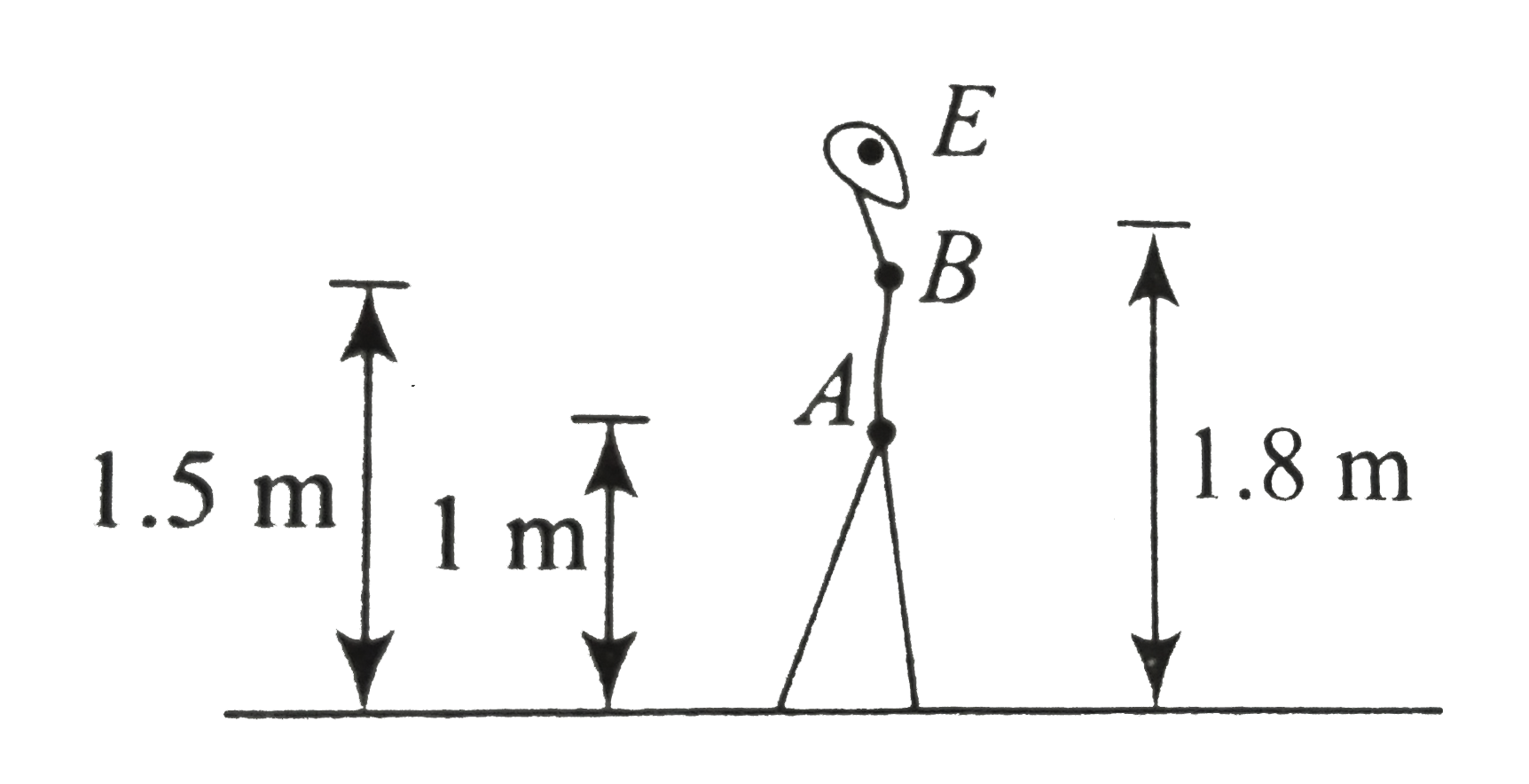
View SolutionYou want to wear your favourite shirt to a party, but the problem is that it is still wet after a wash. What steps would you take to dry it faster ?
View SolutionWhy does a driver use a convex mirror instead of a plane mirror as arear view mirror? Illustrate your answer with the help of a ray diagram.
View SolutionRays of light are incident on a plane mirror at 40∘. At what angle with the first should a second mirror be placed such that the rays emerge from the second mirror parallel to the first mirror.
View SolutionGive five examples of data that you can collect from your day-to-day life.
View SolutionA ray of light is moving from a rarer medium to a denser medium and strikes a plane mirror placed at 90∘ to the direction of the ray as shown in the diagram.
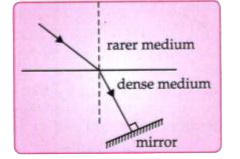
Name the principle you have used to mark the arrows to show the direction of the ray.
View Solution
