mean deviation about median for the following data 2,5,7,2,4,6,10,12,5
2.4
3
1.9
None of these
The correct Answer is:1
To find the mean deviation about the median for the given data set 2,5,7,2,4,6,10,12,5, we will follow these steps:
Step 1: Organize the Data
First, we need to arrange the data in ascending order.
Data in ascending order:
2,2,4,5,5,6,7,10,12
Step 2: Create a Frequency Distribution Table
Next, we will create a frequency distribution table.
| xi | Frequency (fi) |
|---------|---------------------|
| 2 | 2 |
| 4 | 1 |
| 5 | 2 |
| 6 | 1 |
| 7 | 1 |
| 10 | 1 |
| 12 | 1 |
Step 3: Calculate Cumulative Frequency
Now, we calculate the cumulative frequency.
| xi | Frequency (fi) | Cumulative Frequency (cf) |
|---------|---------------------|-------------------------------|
| 2 | 2 | 2 |
| 4 | 1 | 3 |
| 5 | 2 | 5 |
| 6 | 1 | 6 |
| 7 | 1 | 7 |
| 10 | 1 | 8 |
| 12 | 1 | 9 |
Step 4: Find the Median
To find the median, we need to find N2 where N is the total number of observations. Here, N=9, so N2=4.5.
Looking at the cumulative frequency, the median is the value corresponding to the cumulative frequency just greater than 4.5, which is 5. Thus, the median is:
Median = 5
Step 5: Calculate Deviations from the Median
Now, we calculate the absolute deviations from the median.
Deviation=|xi−Median|
| xi | Deviation (|xi−5|) |
|---------|---------------------------|
| 2 | 3 |
| 2 | 3 |
| 4 | 1 |
| 5 | 0 |
| 5 | 0 |
| 6 | 1 |
| 7 | 2 |
| 10 | 5 |
| 12 | 7 |
Step 6: Calculate fi⋅di
Now, we multiply each deviation by its corresponding frequency.
| xi | Frequency (fi) | Deviation (|xi−5|) | fi⋅di |
|---------|---------------------|---------------------------|--------------------|
| 2 | 2 | 3 | 6 |
| 4 | 1 | 1 | 1 |
| 5 | 2 | 0 | 0 |
| 6 | 1 | 1 | 1 |
| 7 | 1 | 2 | 2 |
| 10 | 1 | 5 | 5 |
| 12 | 1 | 7 | 7 |
Step 7: Sum of fi⋅di and fi
Now, we calculate the total of fi⋅di and fi.
∑fi⋅di=6+1+0+1+2+5+7=22
∑fi=9
Step 8: Calculate Mean Deviation
Finally, we calculate the mean deviation about the median using the formula:
Mean Deviation=∑fi⋅di∑fi=229≈2.44
Final Answer
The mean deviation about the median is approximately 2.44.
---
First, we need to arrange the data in ascending order.
2,2,4,5,5,6,7,10,12
Next, we will create a frequency distribution table.
|---------|---------------------|
| 2 | 2 |
| 4 | 1 |
| 5 | 2 |
| 6 | 1 |
| 7 | 1 |
| 10 | 1 |
| 12 | 1 |
Now, we calculate the cumulative frequency.
|---------|---------------------|-------------------------------|
| 2 | 2 | 2 |
| 4 | 1 | 3 |
| 5 | 2 | 5 |
| 6 | 1 | 6 |
| 7 | 1 | 7 |
| 10 | 1 | 8 |
| 12 | 1 | 9 |
To find the median, we need to find N2 where N is the total number of observations. Here, N=9, so N2=4.5.
Now, we calculate the absolute deviations from the median.
|---------|---------------------------|
| 2 | 3 |
| 2 | 3 |
| 4 | 1 |
| 5 | 0 |
| 5 | 0 |
| 6 | 1 |
| 7 | 2 |
| 10 | 5 |
| 12 | 7 |
Now, we multiply each deviation by its corresponding frequency.
|---------|---------------------|---------------------------|--------------------|
| 2 | 2 | 3 | 6 |
| 4 | 1 | 1 | 1 |
| 5 | 2 | 0 | 0 |
| 6 | 1 | 1 | 1 |
| 7 | 1 | 2 | 2 |
| 10 | 1 | 5 | 5 |
| 12 | 1 | 7 | 7 |
Now, we calculate the total of fi⋅di and fi.
∑fi=9
Finally, we calculate the mean deviation about the median using the formula:
The mean deviation about the median is approximately 2.44.
Topper's Solved these Questions
- STATISTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATISTICSEXERCISE - Section-B Linked Comprehension
5 Videos
- STATISTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATISTICSEXERCISE - Section-C Assertion-Reason
15 Videos
- STATISTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATISTICSEXERCISE - Section-C Assertion-Reason
15 Videos
- SETS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-I(Aakash Challengers Questions)
4 Videos
- STRAIGHT LINESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - SECTION-J (AAKASH CHALLENGERS QUESTIONS)
5 Videos
Similar Questions
Find the mean deviation about the median for the following data
13,17,16,11,13,10,16,11,18,12,17
View SolutionFind the mean deviation about the median for the following data:4,6,9,3,10,13,2
View SolutionFind the mean deviation about the mean for the following data:6,7,10,12,13,4,8,12
View SolutionFind the mean deviation about median for the following data:

View SolutionFind the mean deviation about mean for the following data:
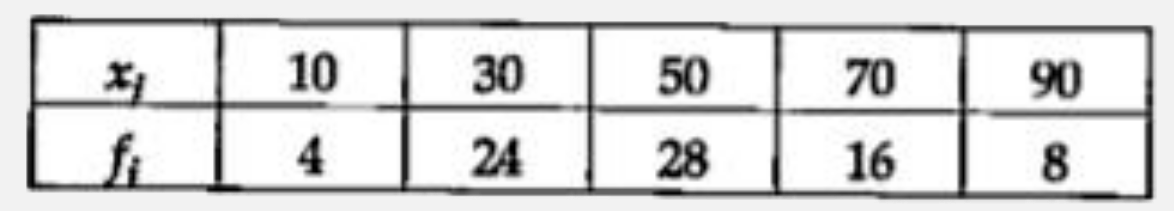
View SolutionCalculate the mean deviation about median from the following data: 340, 150, 210, 240, 300, 310, 320.
View SolutionCalculate the mean deviation about median from the following data: 340, 150, 210, 240, 300, 310, 320.
View SolutionFind the mean deviation about the median for the following data:3,9,5,3,12,10,18,4,7,19,21.
View SolutionFind the mean deviation about the median for the following data:
11, 3, 8, 7, 5, 14, 10, 2, 9
View SolutionCalculate the mean deviation about mean of the following data.(i) 1, 4, 7, 8, 9,10, 12, 13, 17
View Solution
