There are 3 apartments A, B and C for rent in a building . Each apartment will accept either 3 or 4 occupants. The number of ways of renting the apartments to 10 students
12600
10800
13500
15000
The correct Answer is:A
To solve the problem of renting 3 apartments (A, B, and C) to 10 students, where each apartment can accommodate either 3 or 4 occupants, we need to determine the different ways to distribute the students among the apartments.
1. Identify the possible distributions of students:
Since we have 10 students and each apartment can take either 3 or 4 students, the only possible distribution that satisfies the total of 10 students is:
- Apartment A: 3 students
- Apartment B: 3 students
- Apartment C: 4 students
2. Choose the group of 4 students:
We first need to choose which 4 students will occupy Apartment C. The number of ways to choose 4 students from 10 is given by the combination formula:
Number of ways=(104)
Calculating this:
(104)=10!4!(10−4)!=10×9×8×74×3×2×1=210
3. Distribute the remaining 6 students:
After selecting 4 students for Apartment C, we have 6 students left. We need to choose 3 of these 6 students to occupy Apartment A. The number of ways to choose 3 students from 6 is:
Number of ways=(63)
Calculating this:
(63)=6!3!(6−3)!=6×5×43×2×1=20
4. Assign students to apartments:
Now, we have assigned 4 students to Apartment C and 3 students to Apartment A. The remaining 3 students will automatically go to Apartment B.
5. Calculate the total arrangements:
Since the apartments are distinct, we can arrange the students in the apartments in different ways. The arrangement of students in each apartment can be calculated as follows:
- For Apartment C (4 students): 4! ways
- For Apartment A (3 students): 3! ways
- For Apartment B (3 students): 3! ways
Therefore, the total arrangements are:
Total arrangements=4!×3!×3!=24×6×6=864
6. Combine the selections and arrangements:
The total number of ways to rent the apartments is the product of the ways to choose the students and the arrangements:
Total ways=(104)×(63)×(4!×3!×3!)
Substituting the values:
Total ways=210×20×864
7. Calculate the final result:
Now we calculate:
210×20=4200
Then,
4200×864=3628800
Thus, the total number of ways to rent the apartments to 10 students is 3628800.
Since we have 10 students and each apartment can take either 3 or 4 students, the only possible distribution that satisfies the total of 10 students is:
- Apartment A: 3 students
- Apartment B: 3 students
- Apartment C: 4 students
We first need to choose which 4 students will occupy Apartment C. The number of ways to choose 4 students from 10 is given by the combination formula:
Number of ways=(104)
Calculating this:
(104)=10!4!(10−4)!=10×9×8×74×3×2×1=210
After selecting 4 students for Apartment C, we have 6 students left. We need to choose 3 of these 6 students to occupy Apartment A. The number of ways to choose 3 students from 6 is:
Number of ways=(63)
Calculating this:
(63)=6!3!(6−3)!=6×5×43×2×1=20
Now, we have assigned 4 students to Apartment C and 3 students to Apartment A. The remaining 3 students will automatically go to Apartment B.
Since the apartments are distinct, we can arrange the students in the apartments in different ways. The arrangement of students in each apartment can be calculated as follows:
- For Apartment C (4 students): 4! ways
- For Apartment A (3 students): 3! ways
- For Apartment B (3 students): 3! ways
Total arrangements=4!×3!×3!=24×6×6=864
The total number of ways to rent the apartments is the product of the ways to choose the students and the arrangements:
Total ways=(104)×(63)×(4!×3!×3!)
Substituting the values:
Total ways=210×20×864
Now we calculate:
210×20=4200
Then,
4200×864=3628800
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section C Objective type questions (One option is correct )
17 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section D Linked Comprehension Type Questions
12 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section A Objective type questions (One option is correct )
51 Videos
- MATRICESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MATRICESEXERCISE - Assignment (Section - J) Aakash Challengers Questions
3 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
11 Videos
Similar Questions
There are five points A,B,C,D and E. no three points are collinear and no four are concyclic. If the line AB intersects of the circles drawn through the five points. The number of points of intersection on the line apart from A and B is
View SolutionThere are some students in the two examination halls A and B. To make the number of students equal in each hall, 10 students are sent from A to B but, if 20 students are sent from B to A, the number of students in A becomes double the number of students in B, then find the number of students in the both halls.
View Solution
Knowledge Check
Mr. Martinson is building a concrete patio in his backyard and deciding where to buy the materials and rent the tools needed for the project. The table below shows the materials’ cost and daily rental costs for three different stores.
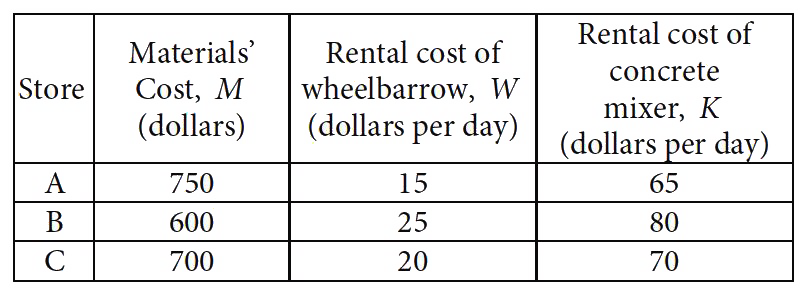
The total cost, y, for buying the materials and renting the tools in terms of the number of days, x, is given by y=M+(W+K)x .
If the relationship between the total cost, y, of buying the materials and renting the tools at Store C and the number of days, x, for which the tools are rented is graphed in the xy-plane, what does the slope of the line represent?AThe total cost of the projectBThe total cost of the materialsCThe total daily cost of the projectDThe total daily rental costs of the tools
Two plane mirrors are plaved parallel to each other and 40 cm apart. An a object is placed 10 cm from one mirror .What is the distance from the object to the image for each of the five images that are closest to the object?
View SolutionIn a large housing complex, there are 15 small buildings and 22 large building. Each of the large buildings has 10 floors with 2 apartments on each floor. Each of the small buildings has 12 floors with 3 apartments on each floor. How many apartments are there in all.
View SolutionTwo charged particles are placed at a distance 1.0 cm apart. What is the minimum possible magnitude of the electric force acting o each charge?
View SolutionTwo charged particles are placed at a distance 1.0 cm apart. What is the minimum possible magnitude of the electric force acting o each charge?
View SolutionTwo identical balls having like charges and placed at a certain distance apart repel each other with a certain force. They are brought in contact and then moved apart to a distance equal to half their initial separation. The force of repulsion between them increases 4.5 times in comparision with the initial value. The ratio of the initial charges of the balls is
View SolutionTwo point charges of 20μC and 80μC are 10 cm apart where will the electric field strength be zero on the line joining the charges from 20μC charge
View SolutionThe electric dipole moment of an electron and a proton 4.3 nm apart, is
View Solution
