Let a line intersects the x-axis at A and y-axis at B resapectively. A line parallel to it is drawn to intersect the axes in P and Q respectively. The extermities of the lines are joined transversely. If the locus of point of intersection of the line joining them is then c is equal to
The correct Answer is:1
To solve the problem step by step, we will analyze the given line and the parallel line, find their intersection points, and then derive the locus of the intersection point.
Step 1: Identify the given line
The equation of the given line is:
xa+yb=1
This line intersects the x-axis and y-axis at points A and B respectively.
Step 2: Find the coordinates of points A and B
- The x-intercept (point A) occurs when y=0:
xa+0b=1⟹x=a⟹A(a,0)
- The y-intercept (point B) occurs when x=0:
0a+yb=1⟹y=b⟹B(0,b)
Step 3: Write the equation of the parallel line
A line parallel to the given line can be expressed as:
xa+yb=k
for some constant k. This line intersects the axes at points P and Q.
Step 4: Find the coordinates of points P and Q
- The x-intercept (point P) occurs when y=0:
xa+0b=k⟹x=ak⟹P(ak,0)
- The y-intercept (point Q) occurs when x=0:
0a+yb=k⟹y=bk⟹Q(0,bk)
Step 5: Find the equations of lines AQ and BP
- The line AQ connects points A and Q:
- A is at A(a,0) and Q is at Q(0,bk).
- The slope of AQ is:
slope of AQ=bk−00−a=−bka
- The equation of line AQ using point-slope form:
y−0=−bka(x−a)⟹y=−bkax+bk
- The line BP connects points B and P:
- B is at B(0,b) and P is at P(ak,0).
- The slope of BP is:
slope of BP=0−bak−0=−bak
- The equation of line BP using point-slope form:
y−b=−bak(x−0)⟹y=−bakx+b
Step 6: Find the intersection point of lines AQ and BP
Set the equations of AQ and BP equal to find the intersection point:
−bkax+bk=−bakx+b
Multiply through by a to eliminate the denominators:
−bkx+abk=−bx+ab
Rearranging gives:
bx−bky=ab−abk
Factoring out b:
b(x−ky)=ab(1−k)
Thus, we have:
x−ky=a(1−k)
Step 7: Find the locus of the intersection point
From the equation x−ky=a(1−k), we can express it in the form:
xa=k⋅yb+(1−k)
This implies:
xa=c⋅yb
where c=1 when k=1.
Conclusion
Thus, the value of c is:
1
The equation of the given line is:
xa+yb=1
This line intersects the x-axis and y-axis at points A and B respectively.
- The x-intercept (point A) occurs when y=0:
xa+0b=1⟹x=a⟹A(a,0)
- The y-intercept (point B) occurs when x=0:
0a+yb=1⟹y=b⟹B(0,b)
A line parallel to the given line can be expressed as:
xa+yb=k
for some constant k. This line intersects the axes at points P and Q.
- The x-intercept (point P) occurs when y=0:
xa+0b=k⟹x=ak⟹P(ak,0)
- The y-intercept (point Q) occurs when x=0:
0a+yb=k⟹y=bk⟹Q(0,bk)
- The line AQ connects points A and Q:
- A is at A(a,0) and Q is at Q(0,bk).
- The slope of AQ is:
slope of AQ=bk−00−a=−bka
- The equation of line AQ using point-slope form:
y−0=−bka(x−a)⟹y=−bkax+bk
- B is at B(0,b) and P is at P(ak,0).
- The slope of BP is:
slope of BP=0−bak−0=−bak
- The equation of line BP using point-slope form:
y−b=−bak(x−0)⟹y=−bakx+b
Set the equations of AQ and BP equal to find the intersection point:
−bkax+bk=−bakx+b
Multiply through by a to eliminate the denominators:
−bkx+abk=−bx+ab
Rearranging gives:
bx−bky=ab−abk
Factoring out b:
b(x−ky)=ab(1−k)
Thus, we have:
x−ky=a(1−k)
From the equation x−ky=a(1−k), we can express it in the form:
xa=k⋅yb+(1−k)
This implies:
xa=c⋅yb
where c=1 when k=1.
Thus, the value of c is:
1
Topper's Solved these Questions
- STRAIGHT LINESBook:AAKASH INSTITUTE ENGLISHChapter:STRAIGHT LINESExercise:SECTION-H (MULTIPLE TRUE-FALSE TYPE QUESTIONS)
Explore 2 Videos
- STRAIGHT LINESBook:AAKASH INSTITUTE ENGLISHChapter:STRAIGHT LINESExercise:SECTION-I (SUBJECTIVE TYPE QUESTIONS)
Explore 5 Videos
- STRAIGHT LINESBook:AAKASH INSTITUTE ENGLISHChapter:STRAIGHT LINESExercise:SECTION-F (MATRIX-MATHC TYPE QUESTION)
Explore 3 Videos
- STATISTICSBook:AAKASH INSTITUTE ENGLISHChapter:STATISTICSExercise:Section-C Assertion-Reason
Explore 15 Videos
- THREE DIMENSIONAL GEOMETRY Book:AAKASH INSTITUTE ENGLISHChapter:THREE DIMENSIONAL GEOMETRY Exercise:ASSIGNMENT SECTION - J
Explore 10 Videos
Similar Questions
The point of intersection of the lines xa+yb=1 and xb+ya=1 lies on
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
View SolutionIn the figure, given, ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.
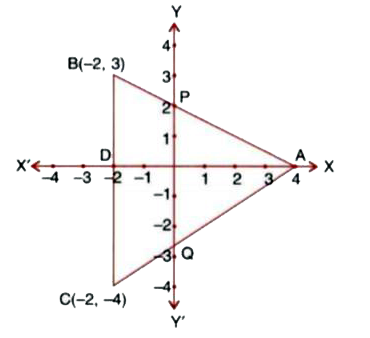
Find the equation of the line AC.
View SolutionThe equation of the line joining the origin to the point of intersection of the lines 2x2+xy−y2+5x−y+2=0 is
View SolutionA line intersects the Y- axis and X-axis at the points P and Q, respectively. If (2,-5) is the mid- point of PQ, then the coordinates of P and Q are, respectively.
View SolutionFind the equation of the line parallel to y-axis and drawn through the point of intersection of the lines x−7y+5=0 and 3x+y=0.
View SolutionFind the equation of the line parallel to y-axis and drawn through the point of intersection of the lines x−7y+5=0 and 3x+y=0.
View SolutionFind the equation of the line parallel to y-axis and drawn through the point of intersection of the lines x−7y+5=0 and 3x+y=0.
View SolutionLet a given line L1 intersect the X and Y axes at P and Q respectively. Let another line L2 perpendicular to L1 cut the X and Y-axes at Rand S, respectively. Show that the locus of the point of intersection of the line PS and QR is a circle passing through the origin
View SolutionA line intersects the y-axis and x-axis at the points P and Q respectively. If (2, 5) is the mid-point of PQ, then find the coordinates of P and Q.
View Solution