If ax+bx≥c,∀a>0anda,b,c are positive constant then
ab≥c24
ab≤c24
bc≥a24
ac≥b24
The correct Answer is:A
To solve the inequality ax+bx≥c where a,b,c are positive constants and x>0, we can follow these steps:
Step 1: Rearrange the Inequality
Start by rewriting the inequality:
ax+bx−c≥0
This can be expressed as:
ax+bx≥c
Step 2: Multiply through by x
Since x>0, we can multiply both sides by x without changing the direction of the inequality:
ax2+b−cx≥0
Rearranging gives us:
ax2−cx+b≥0
Step 3: Analyze the Quadratic
The expression ax2−cx+b is a quadratic in x. For this quadratic to be non-negative for all x>0, we need to check two conditions:
1. The coefficient of x2 (which is a) must be positive.
2. The discriminant of the quadratic must be less than or equal to zero.
Step 4: Calculate the Discriminant
The discriminant D of the quadratic ax2−cx+b is given by:
D=(−c)2−4ab=c2−4ab
For the quadratic to be non-negative for all x, we require:
D≤0⟹c2−4ab≤0
This simplifies to:
4ab≥c2
Step 5: Final Condition
From the above inequality, we can derive:
ab≥c24
Thus, we conclude that the condition for the inequality ax+bx≥c to hold for all x>0 is:
ab≥c24
Conclusion
Since a,b,c are positive constants, the correct option based on the derived condition is:
ab≥c4
Start by rewriting the inequality:
ax+bx−c≥0
This can be expressed as:
ax+bx≥c
Since x>0, we can multiply both sides by x without changing the direction of the inequality:
ax2+b−cx≥0
Rearranging gives us:
ax2−cx+b≥0
The expression ax2−cx+b is a quadratic in x. For this quadratic to be non-negative for all x>0, we need to check two conditions:
1. The coefficient of x2 (which is a) must be positive.
2. The discriminant of the quadratic must be less than or equal to zero.
The discriminant D of the quadratic ax2−cx+b is given by:
D=(−c)2−4ab=c2−4ab
For the quadratic to be non-negative for all x, we require:
D≤0⟹c2−4ab≤0
This simplifies to:
4ab≥c2
From the above inequality, we can derive:
ab≥c24
Thus, we conclude that the condition for the inequality ax+bx≥c to hold for all x>0 is:
ab≥c24
Since a,b,c are positive constants, the correct option based on the derived condition is:
ab≥c4
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION-C( Objective Type Questions ( More than one option are correct ))
1 Video
- APPLICATION OF DERIVATIVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION-C( Objective Type Questions ( More than one option is correct ))
5 Videos
- APPLICATION OF DERIVATIVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION-A (Competition Level Questions)
50 Videos
- APPLICATION OF INTEGRALS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF INTEGRALS EXERCISE - Assignment Section - I Aakash Challengers Questions
2 Videos
Similar Questions
Knowledge Check
Consider the inequality y≥ax2+bx+c, where a , b and c are all positive . Which of the following regions could be the solution set of the inequality ?
A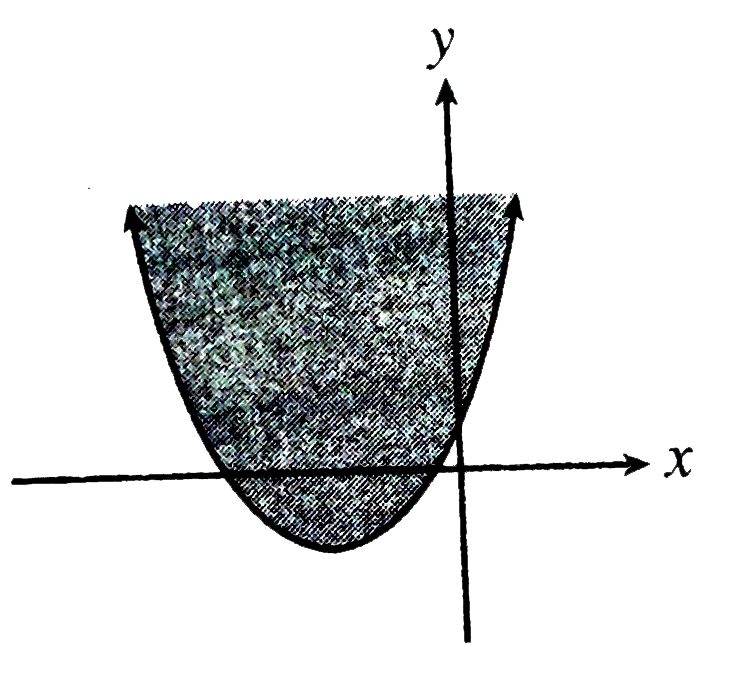 B
B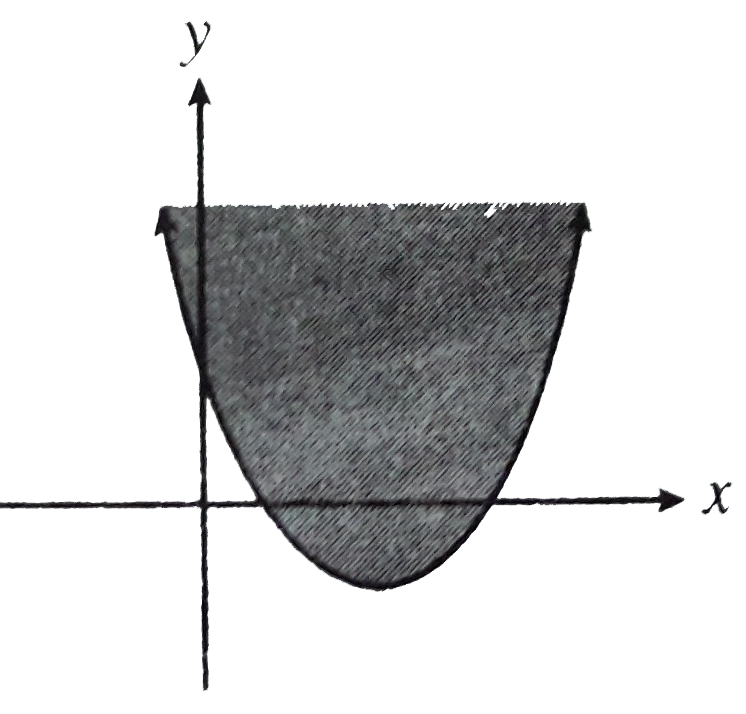 C
C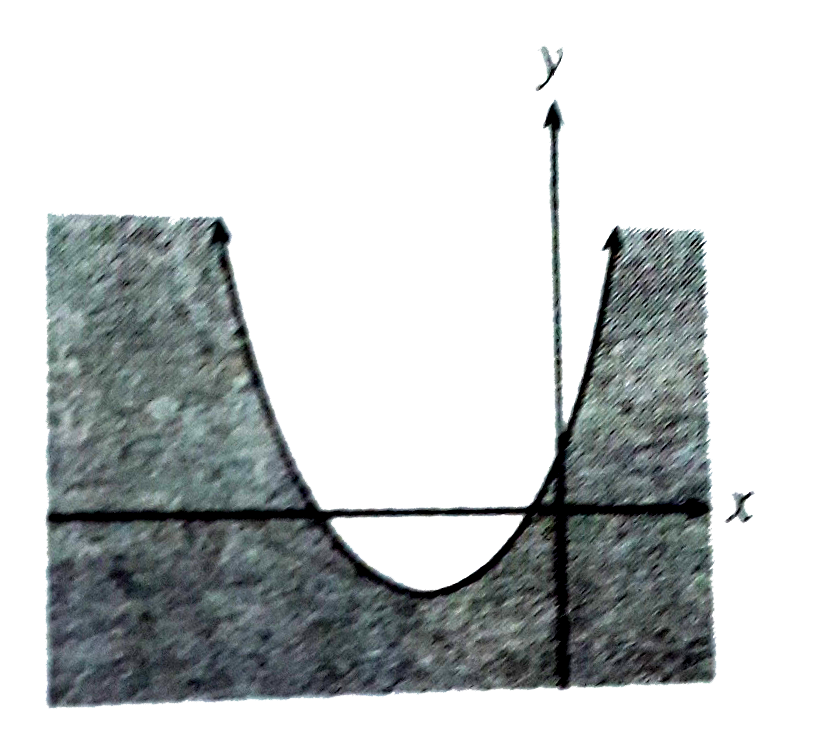 D
D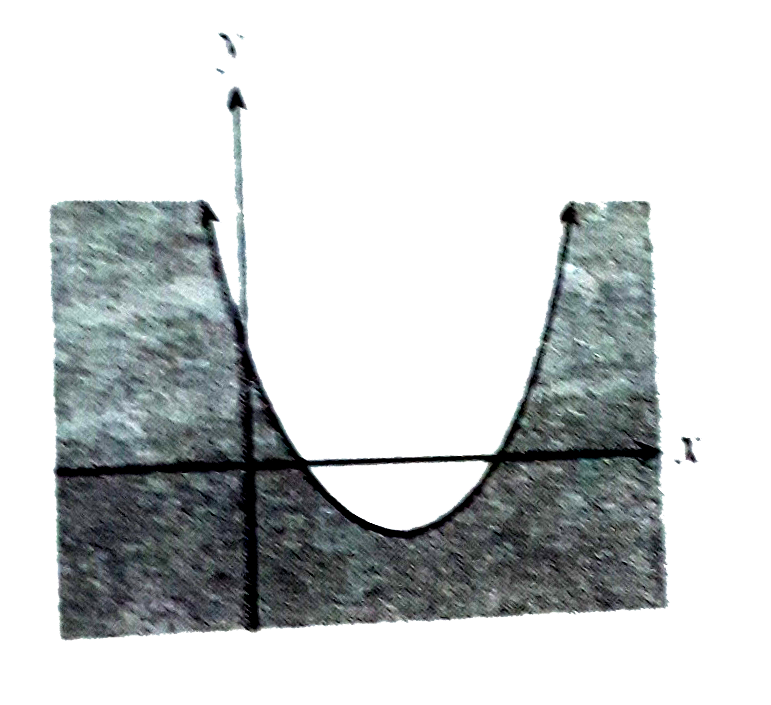
If f(x) is a function satisfying f(x+a)+f(x)=0 for all x∈R and positive constant a such that ∫c+bbf(x)dx is independent of b, then find the least positive value of c
View SolutionLet f(x) be a non-positive continuous function and F(x)=∫x0f(t)dt∀x≥0 and f(x)≥cF(x) where c<0 and let g:[0,∞)→R be a function such that dg(x)dx<g(x)∀x>0 and g(0)=0
The number of solution(s) of the equation
∣∣x2+x−6∣∣=f(x)+g(x) is/are
View SolutionIf f(x) is a function satisfying f(x+a)+f(x)=0 for all x∈R and positive constant a such that ∫c+bbf(x)dx is independent of b, then find the least positive value of ⋅
View SolutionIf a,c>0 and ax2+2bx+3c=0 does not have any real roots then prove that:
(i) 4a−4b+3c>0, (ii) a+6b+27c>0, (iii) a+2b+6c>0
View SolutionThe function f:C→C defined by f(x)=ax+bcx+d for x∈C where bd≠0 reduces to a constant function if
View SolutionLet f(x)=a+b|x|+c|x|4 , where a, b, and c are real constants. Then, f(x) is differentiable at x=0 , if
View SolutionIf a,b,c are in H.P, where a>c>0, then :
View Solution