Let the function defined in Colomn-I have domain (0,π2) then match the following
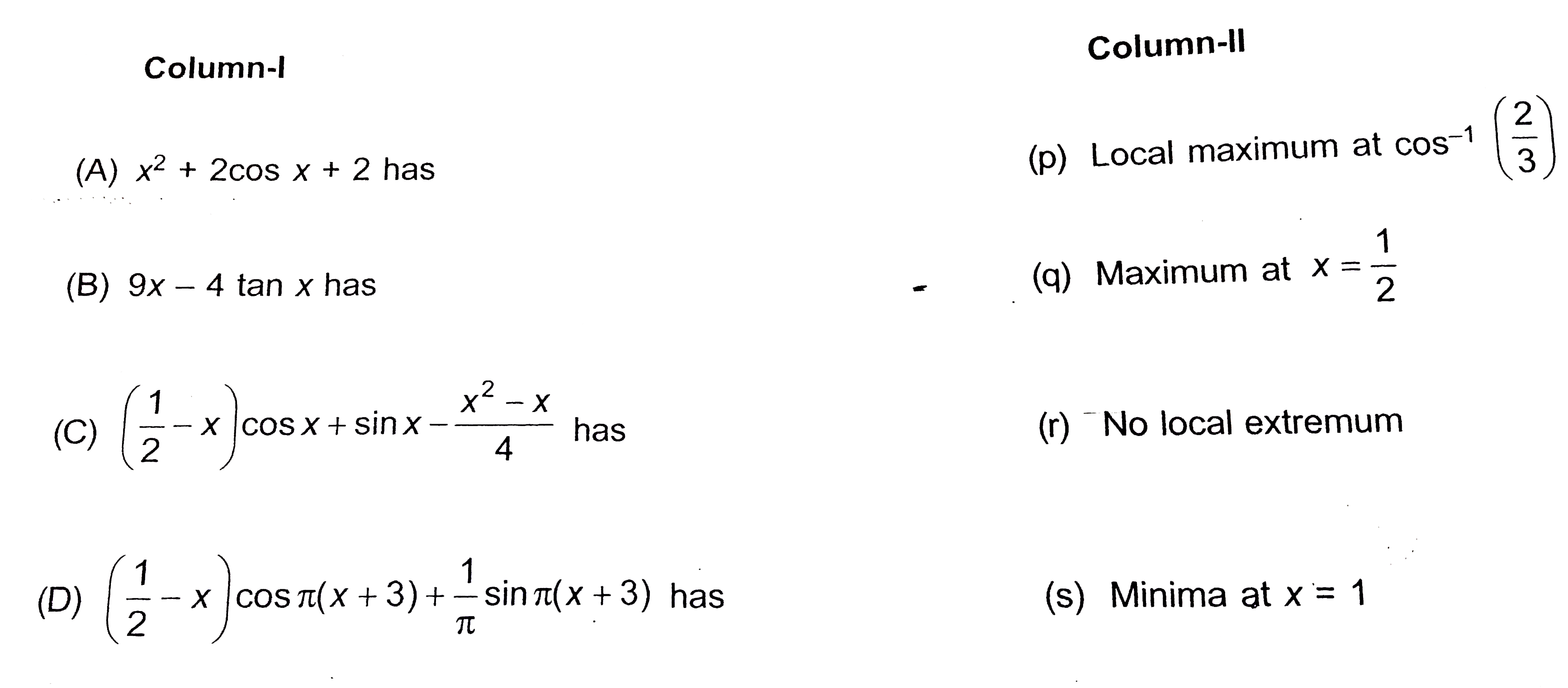
Text Solution
A(r), B(p), C(q), D(q,s)
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION- G ( Integer Answer Type Questions )2videos
APPLICATION OF DERIVATIVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION- H ( Multiple True-False Type Questions ( Identify the correct statement of true (T) and False (F) of the given three statements ).)1videos
APPLICATION OF DERIVATIVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION-E ( Assertion -Reason Type Questions )4videos
APPLICATION OF DERIVATIVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF INTEGRALS EXERCISE - Assignment Section - I Aakash Challengers Questions2videos
APPLICATION OF INTEGRALS
Similar Questions
Knowledge Check
- Question 1 - Select One
Let f be the function defined on R as follows
Af is continuous at 0Bf is discontinuous at 0Cf is nowhere at 0Df is everywhere discontinuous - Question 2 - Select One or More
Let the function f(x)=sinx+cosx, be defined in [0,2π], then f(x)
Aincreases in (π/4,π/2)Bdecreases in [π/4,5π/4]Cincreases in [0,π/4]∪[5π/4,2π]Ddecreases in [0,π/4)∪(π/2,2π] - Question 3 - Select One or More
Let f:I−{−1,0,1}→[−π,π] be defined as f(x)=2tan−1x−tan−1(2x1−x2), then which of the following statements (s) is (are) correct ?
Af(x) is bijectiveBf(x) is injective but not surjectiveCf(x) is neither injective nor surjectiveDf(x) is an odd function
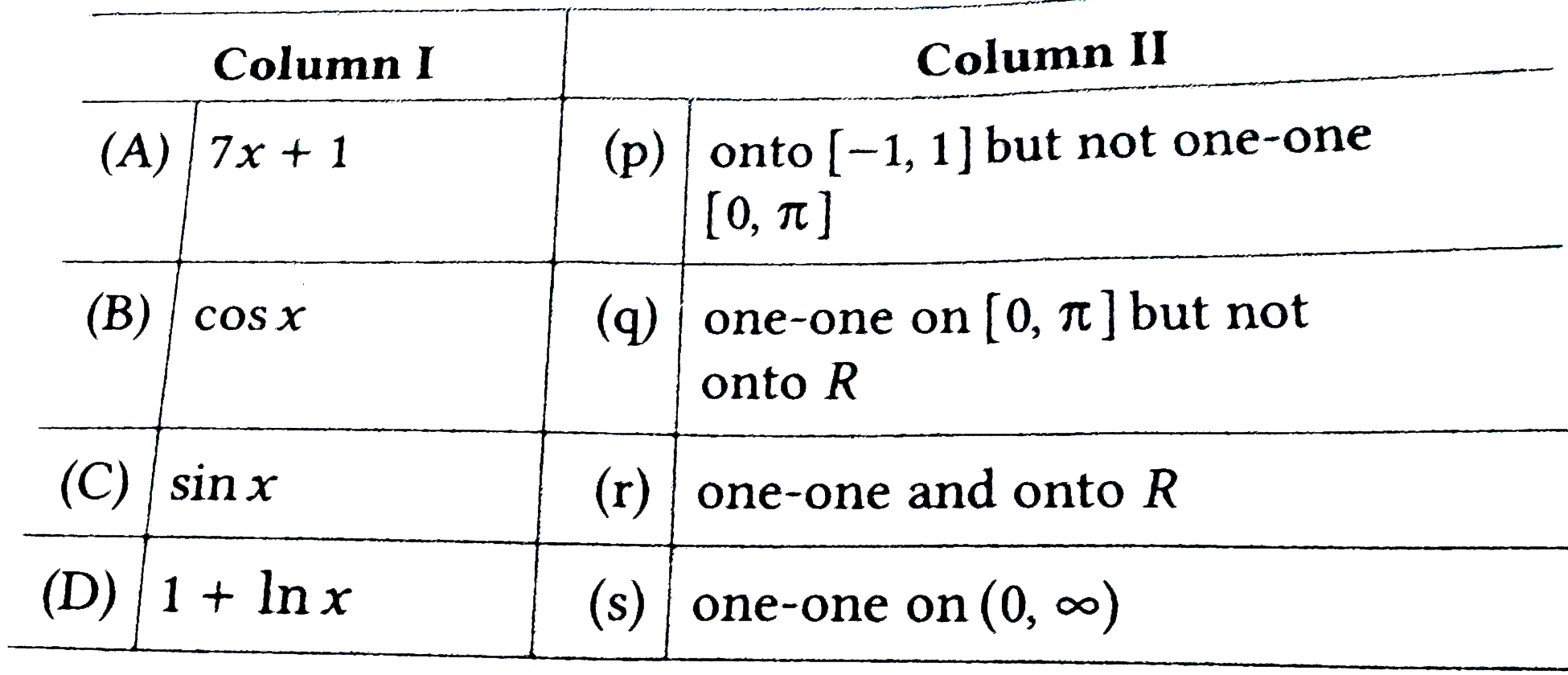
The functions defined have domain R
 View Solution
View SolutionMatch the conditions/ expressions in Column I with statements in Column II.
Let the functions defined in Column I have domain (−π/2,π/2).
(##41Y_SP_MATH_C10_E02_025_Q01.png" width="80%">View SolutionThe function f(x) is defined in [0,1]. Find the domain of f(tanx).
View SolutionThe function f(x) is defined in [0,1]. Find the domain of f(tanx).
View SolutionLet f(x) be an onto function defined from [0, 2] to [3, 5] . hich of the following is/are CORRECT ?
View Solution

