A projectile has the same range R for angles of projections. If T1andT2 be the times of fight in the two cases, then ( using θ as the angle of projection corresponding to T1 )
T1T2=2Rg
T1T2=R2
T1T2=tanθ
T1T2=1
The correct Answer is:A, C
To solve the problem step by step, we will derive the relationship between the times of flight T1 and T2 for a projectile launched at two complementary angles that yield the same range.
1. Understanding the Time of Flight:
The time of flight T for a projectile launched at an angle θ with an initial speed u is given by:
T=2usinθg
For our two angles, let T1 be the time of flight for angle θ and T2 for angle 90∘−θ.
2. Finding T2:
The time of flight for the angle 90∘−θ can be expressed as:
T2=2usin(90∘−θ)g=2ucosθg
3. Expressing the Range:
The range R of a projectile is given by:
R=u2sin2θg
Using the identity sin2θ=2sinθcosθ, we can rewrite the range as:
R=2u2sinθcosθg
4. Relating sinθ and cosθ to Time of Flight:
From the expressions for T1 and T2:
- From T1:
sinθ=gT12u
- From T2:
cosθ=gT22u
5. Substituting into the Range Equation:
Substitute sinθ and cosθ into the range equation:
R=2u2(gT12u)(gT22u)g
Simplifying this gives:
R=2u2⋅gT1T24u2g=T1T22
6. Final Relationship:
Rearranging the equation gives:
T1T2=2R
Conclusion:
Thus, we find that the relationship between the times of flight T1 and T2 for the two angles of projection that yield the same range R is:
T1T2=2R
The time of flight T for a projectile launched at an angle θ with an initial speed u is given by:
T=2usinθg
For our two angles, let T1 be the time of flight for angle θ and T2 for angle 90∘−θ.
The time of flight for the angle 90∘−θ can be expressed as:
T2=2usin(90∘−θ)g=2ucosθg
The range R of a projectile is given by:
R=u2sin2θg
Using the identity sin2θ=2sinθcosθ, we can rewrite the range as:
R=2u2sinθcosθg
From the expressions for T1 and T2:
- From T1:
sinθ=gT12u
- From T2:
cosθ=gT22u
Substitute sinθ and cosθ into the range equation:
R=2u2(gT12u)(gT22u)g
Simplifying this gives:
R=2u2⋅gT1T24u2g=T1T22
Rearranging the equation gives:
T1T2=2R
Thus, we find that the relationship between the times of flight T1 and T2 for the two angles of projection that yield the same range R is:
T1T2=2R
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -D (Linked Comprehension)
12 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -E (Assertion-Reason)
4 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -B Objective (one option is correct)
29 Videos
- MOCK_TEST_17BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOCK_TEST_17EXERCISE - Example
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
A projectile can have the same range R for two angles of projection. If t1 and t2 be the times of flight in the two cases:-
View SolutionFor a given velocity, a projectile has the same range R for two angles of projection. If t1andt2 are the times of flight in the two cases then:
View SolutionA projectile can have same range R for two angles of projection. It t1andt2 are the times of flight in the two cases, then what is the product of two times of flight ?
View SolutionFor a given velocity, a projectile has the same range R for two angles of projection. If t1 and t2 are the time of flight in the two cases, then t1⋅t2 is equal to
View SolutionA projectile can have the same range 'R' for two angles of projection . If 'T1' and 'T2' to be times of flights in the two cases, then the product of the two times of flights is directly proportional to .
View SolutionA projectile has same range for two angules of projection. If times of flight in two cases are t1andt2 then the range of the projectilie is
View SolutionIf T1andT2 are the times of flight for two complementary angles, then the range of projectile R is given by
View SolutionIf the time of flight of a bullet over a horizontal range R is T, then the angle of projection with horizontal is -
View SolutionA body is projected with a certain speed at angles of projection of θ and 90−θ The maximum height attained in the two cases are 20m and 10m respectively. The range for angle of projection θ is
View SolutionTwo stones are projected from level ground. Trajectory of two stones are shown in figure. Both stones have same maximum heights above level ground as shown. Let T1 and T2 be their time of flights and u1 and u2 be their speeds of projection respectively (neglect air resistance). then
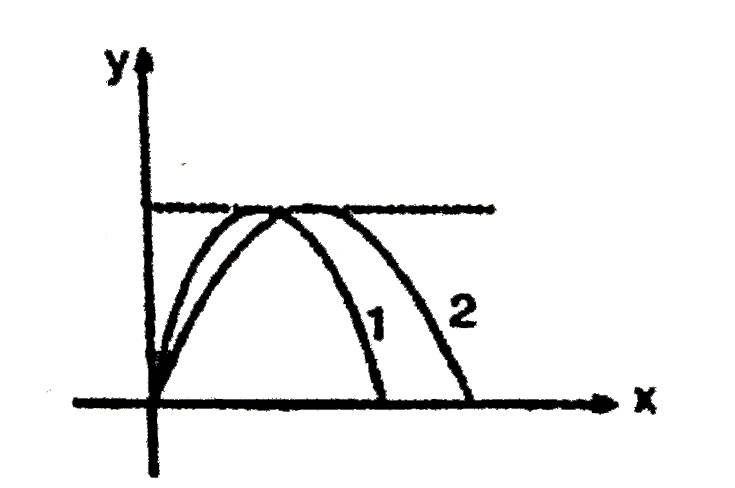
View Solution
