While solving logarithmic equations or logarithmic
inequalities care must be taken to ensure that the value
of the variable obtained do indeed satisfy the given
equation . Often the solution consists in transforming the original equation to form which can be solved with ease .
But in bargain the process the transformations carried
out are not always equivalent .In what follows one must
verify that the values of variables obtained indeed satisfy
original equation or inequation.
How many solutions in real numbers does the
equation 3x8xx−2=6 have ?
i) None
ii) Exactly one
iii) Exactly two
iv) Infinite
None
Exactly one
Exactly two
Infinite
The correct Answer is:3
To solve the equation 3x⋅8xx−2=6, we will follow these steps:
Step 1: Rewrite the equation
We start with the original equation:
3x⋅8xx−2=6
We can express 8 as 23:
3x⋅(23)xx−2=6
This simplifies to:
3x⋅23xx−2=6
Step 2: Rewrite the right side
Next, we can express 6 as 31⋅21:
3x⋅23xx−2=31⋅21
Step 3: Compare the bases
Now we can compare the powers of the bases 3 and 2:
1. For the base 3:
x=1
2. For the base 2:
3xx−2=1
Step 4: Solve for x from the second equation
From the equation 3xx−2=1, we can cross-multiply:
3x=x−2
Rearranging gives:
3x−x=−2⟹2x=−2⟹x=−1
Step 5: Verify the solutions
We found two potential solutions: x=1 and x=−1. We need to check if both satisfy the original equation.
1. Check x=1:
31⋅811−2=3⋅8−1=3⋅18=38(not equal to 6)
2. Check x=−1:
3−1⋅8−1−1−2=13⋅8−1−3=13⋅813=13⋅2=23(not equal to 6)
Conclusion
Neither x=1 nor x=−1 satisfies the original equation. Therefore, the equation has no solutions in real numbers.
Final Answer
The correct option is:
i) None
We start with the original equation:
3x⋅8xx−2=6
We can express 8 as 23:
3x⋅(23)xx−2=6
This simplifies to:
3x⋅23xx−2=6
Next, we can express 6 as 31⋅21:
3x⋅23xx−2=31⋅21
Now we can compare the powers of the bases 3 and 2:
1. For the base 3:
x=1
2. For the base 2:
3xx−2=1
From the equation 3xx−2=1, we can cross-multiply:
3x=x−2
Rearranging gives:
3x−x=−2⟹2x=−2⟹x=−1
We found two potential solutions: x=1 and x=−1. We need to check if both satisfy the original equation.
31⋅811−2=3⋅8−1=3⋅18=38(not equal to 6)
3−1⋅8−1−1−2=13⋅8−1−3=13⋅813=13⋅2=23(not equal to 6)
Neither x=1 nor x=−1 satisfies the original equation. Therefore, the equation has no solutions in real numbers.
The correct option is:
i) None
Topper's Solved these Questions
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - E) Assertion-Reason
12 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - G) Integer
6 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - C) More than one option are correct
11 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions
8 Videos
- SETS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-I(Aakash Challengers Questions)
4 Videos
Similar Questions
White solving logarithmic equations or logarithmic
inequalities care must be taken to ensure that the value
of the variable obtained do indeed satisfy the given
equation . Often the solution consists in transforming the original equation to form which can be solved with ease .
But in bargain the process the transformations carried
out are hot always equivalent .In what follows one must
verify that the values of veriables obtained indeed satisfy
original equation or inequation.
Let S be the set of all solutions x in real numbers of
the equation (log5x)2+log5x(5)x=1 . Then ∑x∈Sx
View SolutionHow is the following transformation best carried out?
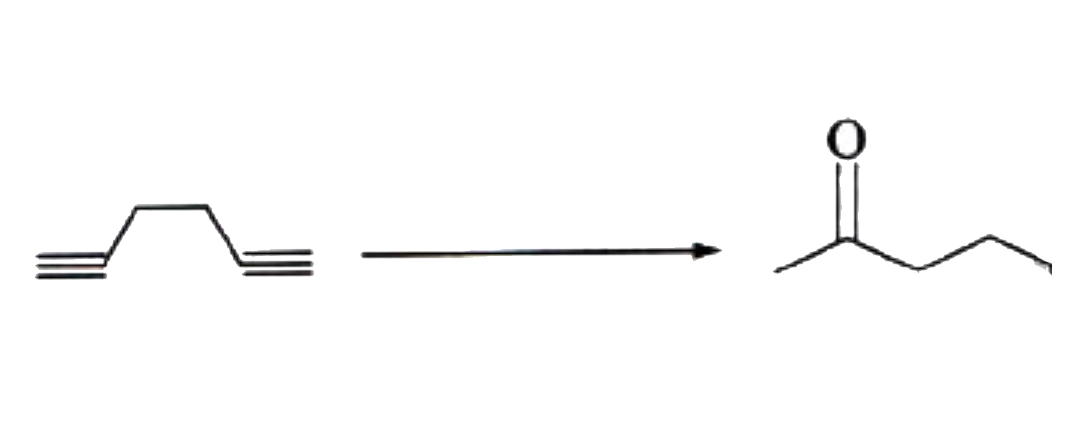
View Solution
Knowledge Check
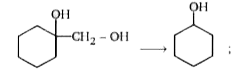 Above transformation can be carried out by :AH+/Δzn(Hg),HClBHlO4,LiAlH4CHlO4,H+/ΔDH+/Δ,HiO4
Above transformation can be carried out by :AH+/Δzn(Hg),HClBHlO4,LiAlH4CHlO4,H+/ΔDH+/Δ,HiO4How is the following transformation best carried out?
 AOsO4,NaHCO3BH2SO4/H2OCHgSO4/H2SO4DHIO4
AOsO4,NaHCO3BH2SO4/H2OCHgSO4/H2SO4DHIO4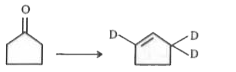
Arrange the following reagent in the correct order in which above transformation is carried out :AKOD/D2O,H+/Δ,LiAlH4BH+/Δ,KOD/D2O,LiAlH4CKOD/D2O,LiAlH4,H+/ΔDLiAlH4,H+/Δ,KOD/D2O
Write the logarithmic equation for E=12mv2
View SolutionVerify if the given values of the variables are the solutions of the corresponding equations.
13=4y–3,y=4
View SolutionSolve the following equations:
x4+2=3
View SolutionVerify if the given values of the variables are the solutions of the corresponding equations.
3x−1=8,x=5
View SolutionTransform the equation x+y+1=0 into normal form.
View Solution
