If A and B are two sets such that A×B=ϕ, then which of the following must be true ?
A=ϕ and B≠ϕ
A=ϕ and/or B=ϕ
A≠ϕ and B=ϕ
A=ϕ and B=ϕ
The correct Answer is:B
To solve the problem, we need to analyze the condition given: A×B=ϕ, where A and B are two sets, and ϕ represents the empty set.
1. Understanding the Cartesian Product: The Cartesian product A×B consists of all ordered pairs (a,b) where a∈A and b∈B. If A×B=ϕ, it means there are no such pairs.
2. Condition for the Empty Set: The Cartesian product A×B is empty if at least one of the sets A or B is empty. This is because:
- If A is empty (A=ϕ), then there are no elements a to pair with any element b in B.
- If B is empty (B=ϕ), then there are no elements b to pair with any element a in A.
3. Conclusion: Therefore, for A×B to be empty, it must be true that either A is empty, B is empty, or both are empty. However, the strongest conclusion we can draw is that at least one of the sets must be empty.
4. Final Statement: Thus, we conclude that if A×B=ϕ, then at least one of the sets A or B must be the empty set.
Answer:
The correct conclusion is that either A=ϕ or B=ϕ (or both).
---
- If A is empty (A=ϕ), then there are no elements a to pair with any element b in B.
- If B is empty (B=ϕ), then there are no elements b to pair with any element a in A.
The correct conclusion is that either A=ϕ or B=ϕ (or both).
Topper's Solved these Questions
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - B) Objective Type Questions (one option is correct)
86 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - C) Objective Type Questions (More than one option are correct)
17 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Try Yourself
70 Videos
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-J (aakash challengers questions)
11 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers
11 Videos
Similar Questions
Knowledge Check
If ab>0, then which one of the following must be true?
Aa/b>0Ba−b>0Ca+b>0Db−a>0If x+y=90∘, which of the following must be true?
Acosx=cosyBsin x=-sin yCtan x=cot yDsin x+cos y=1If a and b are nonzero numbers such that a lt b , which of the following must be true ?
I. 1a>1b
II. a2<b2
III.b2≥1ANoneBI onlyCII onlyDIII only
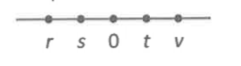
Which of the following MUST be true?
rs>v
View SolutionIf A and B are two sets such that A⊂B , then what is A∪B ?
View SolutionIf A and B are two sets prove that A∩(B−A)=ϕ
View Solution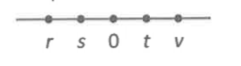
Which of the following MUST be true?
v+s>t+r
View SolutionIf AandB are two square matrices such that they commute, then which of the following is true?
View Solution